Community Tip - You can change your system assigned username to something more personal in your community settings. X
- Community
- Creo+ and Creo Parametric
- 3D Part & Assembly Design
- Combine three curves made with equations
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Combine three curves made with equations
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Combine three curves made with equations
Hello everybody,
I was wondering if there is a way to combine/merge three curves made with equations into one curve.
As you can see in the attached files, the curves form a spring.
If zoomed in, little differences between the curves can be seen.
I'm looking for a way to eliminate these gaps and get one whole curve out of three.
Thank you for your time and with best regards.
- Labels:
-
General
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The only way I can think of is to develop a single equation-driven curve to build the thing. The problem I've always had with this type of thing is the tangency between the two curves doesn't match so any further geometry created using the curves will have a discontinuity.
If you're trying to model a realistic spring that can be adjusted to compress/stretch to fit a particular assembly, you really should do a search here and on YouTube to see some very helpful methodologies people have developed. It's not a simple thing to do, but once you have a robust model it's very nice for all the places you might need to use a spring. Downloaded springs from suppliers are always "dumb" geometry, lack the features needed to place them, etc.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If the three curves are tangent, you can copy them using approximate to create a single curve.
Select first curve and press Ctrl+C and Ctrl+V.
Change curve type to Approximate.
Hold Shift and select remaining curves.
Complete feature
Curves must be tangent for Approximate to work.
There is always more to learn in Creo.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
A datum graph may be able to control a single curve to create the curve shape you want.
There is always more to learn in Creo.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Add small fillet radii at the discontinuities where the curves should "join". Once the radii are present you can create a composite curve (as previously mentioned) by selecting the three curves and the 1 or 2 radii required to get C1 continuity between them. A formed coil will have the bend radii in the actual part so the model should match the formed part more closely.
The spring shown below transitions from a helical path to a planar one at both ends. This model uses curves on each end that match the helix to deal with the discontinuity. This approach can also be used to resolve your issue but is more tedious than adding the fillets between curves.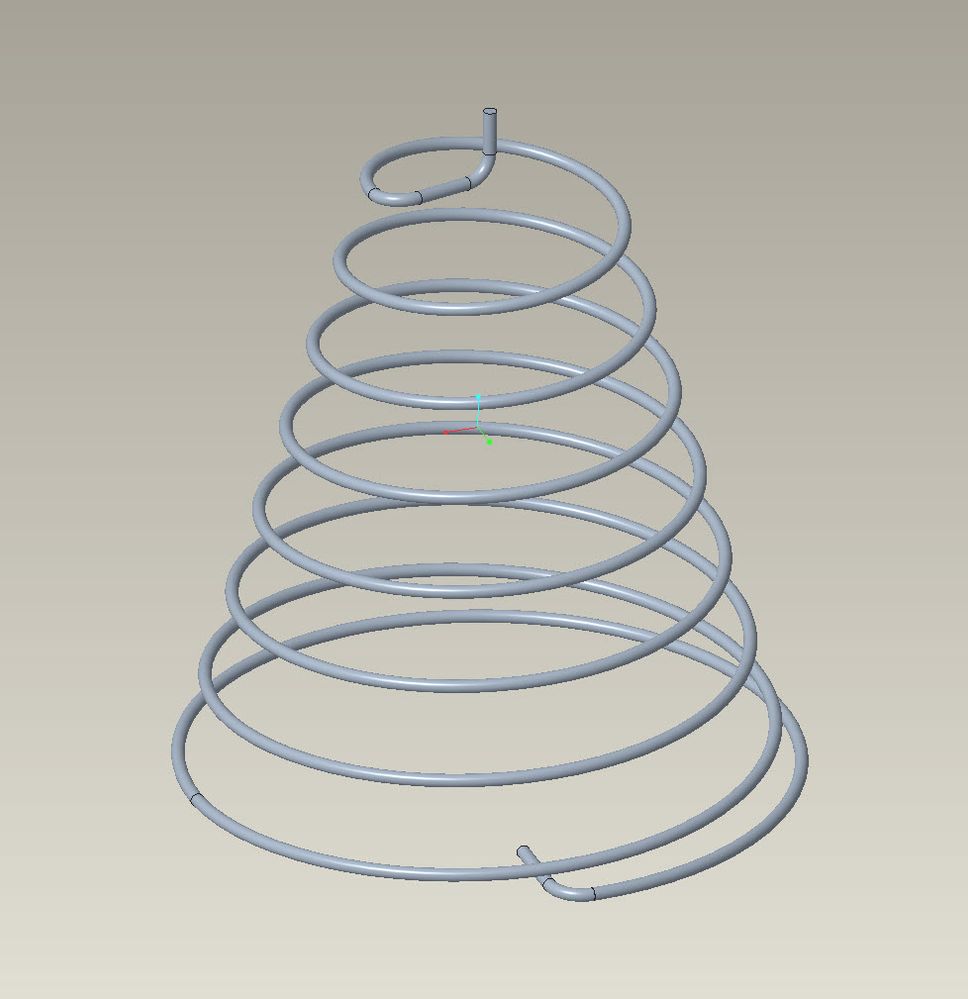
Involute Development, LLC
Consulting Engineers
Specialists in Creo Parametric
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you for your reply.
The spring will be exported and used in finite element methods. It will not be processed for any Creo assembly at all, so no real functionalities are required.
Thing is, as you can see in the attached picture, the whole curve cannot be modeled within one equation-driven curve because the z-coordinate is seperated into three functions, depending on a parameter. At the bottom and the top, a half of a circle is needed on the same z-coordinate, while between the top and the bottom a helix form is needed. To my knowledge it is not possible to use if-conditions in an equation.
Thank you for your replies and your suggestions. I will try them out.
After all I found a workaround: It was possible to extract points along all three curves and then create one curve interpolating these points. The result was quite accurate and could be successfully exported.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
In case it's amenable to your situation, it is true that IF conditions are not allowed for equation driven curves. But, in some situations, I've been able to cheat by using the MAX or MIN functions as a pseudo-IF clause. Doesn't necessarily solve the tangency problem, though.
For other messes I've gotten myself into, like a twisted wire, the previously suggested sketch-driven profile has worked nicely.
This stuff is kind of stress-inducing, but when you figure it out it's pretty cool.


