- Community
- Creo+ and Creo Parametric
- 3D Part & Assembly Design
- Simple things sometimes just aren't
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Simple things sometimes just aren't
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Simple things sometimes just aren't
Hello,
This is a problem I have managed to avoid or work around for years and annoyingly I have failed to retire before it came back again demanding a solution.
I think it has been mentioned before but I couldn't find the reference.
Does anyone have a sensible method (with compromises)?
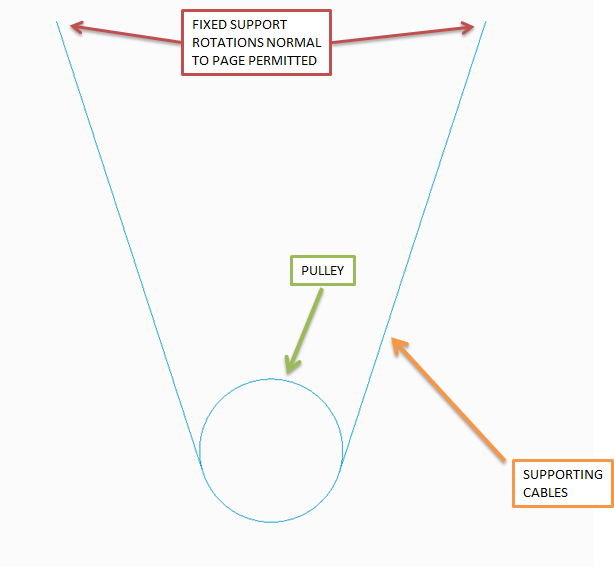
The first image is of a pulley and cables. text book stuff.
Assume frictionless pulley, light inextensible cables. Gravity acts vertically down.
Frictionless means that the tension in the cables are equal.
Now we point gravity in a direction other than down.

Frictionless and 'light' means that cable tensions are the same.
Inextensible means that the length of cable between supports (via the pulley) does not change.
The tangent points on the pulley change.
There is nothing I have put here that this forum would have difficulty with.
In the model I have, the supports are part of a flexible structure and will change their positions according to the gravity vector.
How to model a general case?
Nearly Easter
Thanks
This thread is inactive and closed by the PTC Community Management Team. If you would like to provide a reply and re-open this thread, please notify the moderator and reference the thread. You may also use "Start a topic" button to ask a new question. Please be sure to include what version of the PTC product you are using so another community member knowledgeable about your version may be able to assist.
- Labels:
-
Routed Syst. Design
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The simplest way is probably to do hand calculations using lots of trigonometry to figure out the position of the pulley, then model that state in Creo Simulate.
Or is there an objection against this method?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I think you're right Patrick.
No objection per se. Create the locus of each tangent point, construct geometry to point loads in directions using datum points.
I wanted to attempt to include the support movements whose relative magnitude of movement is a bit more than second order.
Thanks


