Community Tip - When posting, your subject should be specific and summarize your question. Here are some additional tips on asking a great question. X
- Community
- Creo+ and Creo Parametric
- Analysis
- Calling trajpar wizz-kids
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Calling trajpar wizz-kids
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Calling trajpar wizz-kids
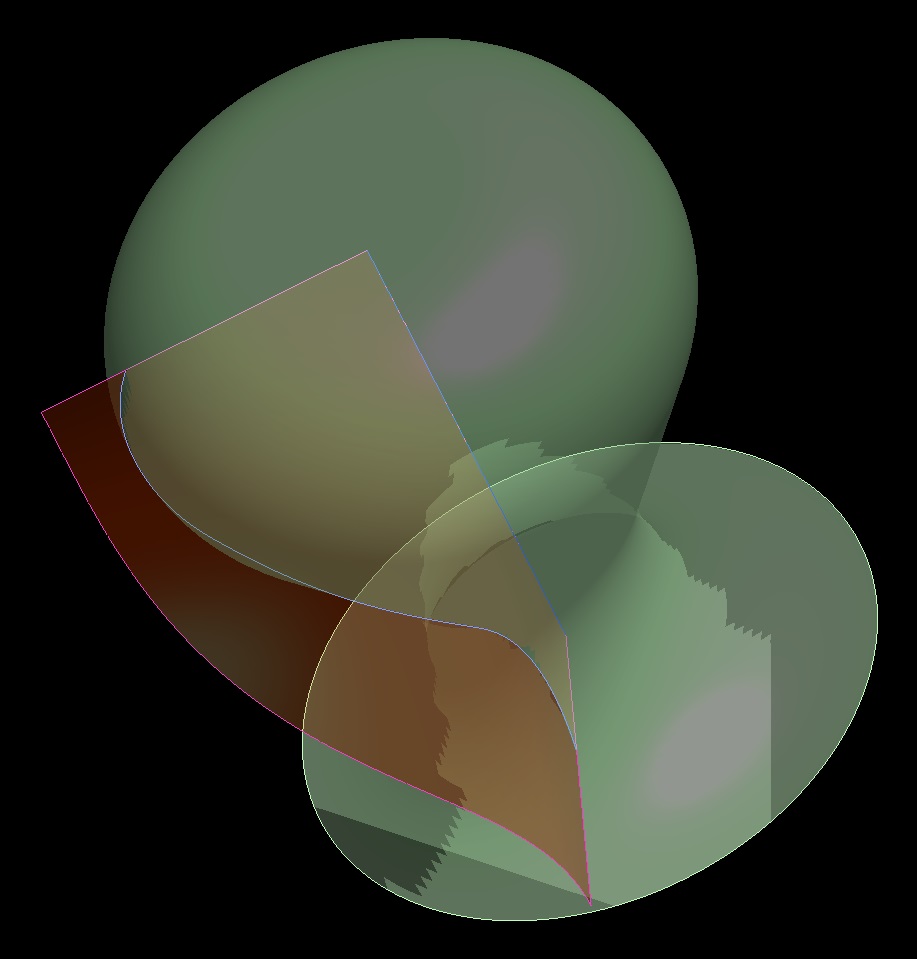
Hello, i am trying to create the curve shown in the attached image, by relation, using trajpar function. In this image the curve was simply projected onto the surface (which is a revolve), just to show what i require, but i would like to control it by relation, as mentioned already. The curve needs to turn through 75 degrees & be normal to the horizontal planes at each end. I just need someone to point me in the right direction, with how to right the relation. I am getting close, i just cannot get the normal condition on the ends. Any Ideas?
John
This thread is inactive and closed by the PTC Community Management Team. If you would like to provide a reply and re-open this thread, please notify the moderator and reference the thread. You may also use "Start a topic" button to ask a new question. Please be sure to include what version of the PTC product you are using so another community member knowledgeable about your version may be able to assist.
Solved! Go to Solution.
- Labels:
-
General
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
At 75 degrees, you will not obtain a normal on both ends... technically, that is 180 degrees of phase to obtain that.
What I think you -are- trying to tie the 75 degrees to is the wrap angle around your object, and this is easily enough to accomplish.
the trajpar in this case is a sin/cos function. Technically, a cos() because you want a tangent end (to vertical). Your sign sweep is 180 degrees; your amplitude is whatever makes the project cover 75 degrees around your object. For that you place points at your endpoints and capture a measurement that can be used in your curve sketch relations.
Simple remember a few things... your amplitude is the horizontal distance... while the cos() function will swing from -1 to 1. Add the math to get the appropriate amplitude based on the horizontal measurement (you can do some math here too but it is not directly cylindrical so you have to know the diameter at each endpoint).
Open trajpar features are values from 0-1, however, cos and sin versions require the angle. The angle will be the value from (0 to 1) * 180
Do be careful, however... this is still a projected sine curve and it will not be accurate at any angle other than the projected angle. A wrap will be different as well and will require different math. In order to make is a sine curve along the surface, you will have to use some extra techniques and a different approach.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I do not 100% understand what you are asking.
Is the red line going to be a trajectory for something on the side of the revolve?
Or are you talking about the blue line?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Yes, the red curve is what I am after, & yes, it will be a trajectory for a sweep on the revolved surface. Sorry for the confusion.
John
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I knew I could count on you to think outside the box, Frank ![]()
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I've been told sometimes I'm not even on the same PLANET! Is my "Colorado" showing? LOLZ ![]()
I totally suck at math, so I avoid it when I can! I think more in images, geometry. Too bad we don't live in the same area, I think we would make an AWESOME team if we were to do a start-up! ![]()
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I took your evalgraph() version and took it to the next level to control the length of the vertically swept line to meet the revolved surface (relations for the profile driving the graph), and although it worked flawlessly, it looks quite funny.
And I tried wrapping to cosine curve and of course, if fails.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hopefully soon we'll be on creo2 so I can FINALLY see your models!
I made the trajectory shorter because I thought that's what Juhn wanted from his example.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I was just playing with that one. If I extend the blue surface out to a cylindrical extent, it again has the curve like yours.
I still used the sweep's true cosine equation but the graph controlled the edge... the length of the line. It fell right on the body. It certainly doesn't look like a "projected" sine wave. What I never thought about was that you can tie relations to the graph, making the graph be the same as the shape of the object always track. That really helps.
I wish there was a way to actually put equation curves in sketches using a "trajpar" method. Splines are still approximations. As we all know, they make very poor circles as well unless you bind them by an equation.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hmmmmm, you just gave me an idea........ ![]()
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Oh-oh ![]()
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks guys, for all your help with this. In this instance, Frank takes the gold medal. When i said i was close, it was his approach i was attempting, using evalgraph & trajpar, i just could not get the graph right, which led to some rather strange results. Thanks again.
John
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks John/Tom! The nice thing about graphs, is that they are so powerful. You can drive just about anything with one, and get exactly the result you want. You can reverse rotation, or get the ends tangent to the end planes if you wish. I'm assuming this is for some kind of cam profile on a barrel cam perhaps?
I've actually been using this technique for many years, since maybe '99. I as playing with trajpar from a Pro/E lesson, and wondered if I could call up a graph. After trial and error I got it to work. To my knowledge PTC hasn't formally taught this techniwue. Or, at least, I've had several advanced classes over the years where trajpar was taught and never was a graph used like this.