Dimensional Constants in Valve Sizing Equations?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Dimensional Constants in Valve Sizing Equations?
The various equations for finding required Cv for valve sizing contain what look like dimensional constants that depend on the units chosen. For example:
But, I'm having trouble using this equation in Mathcad, since I don't know what is included in the constant 63.4 and I don't know if Cv should have any units or not. Anybody have any experience or ideas? Please see the worksheet attached. Thanks for any help you can lend.
- Labels:
-
Other
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I would assume that Cv is unitless (described as a "coefficient" and no unit listed).
I can't open your file, but here are two possible ways to approach equations like this:
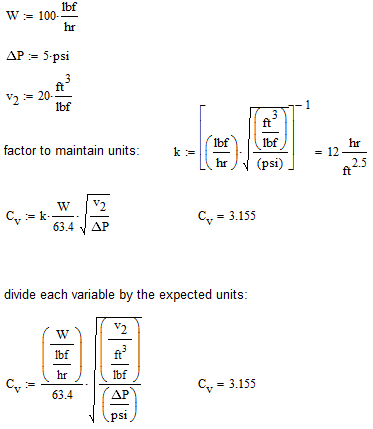
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks Mark. I like your idea of using the "k" factor to deal with the units within the equation. I think it's rather elegant. However, it leaves unanswered the question of what goes into the 63.4 constant. I wish I could see where that came from. Regarding Cv, the discussions of its origins say that it was the gallons per minute of 60 degree F water that would flow through a valve with a 1 psi pressure drop. I think that would mean that Cv would probably have some units, even though, as you point out, some similar things, like discharge coefficients, are dimensionless.
I noticed something else yesterday. In the English unit system, 2g often appears in equations. 2g is 2*32.17405=64.35, which is close to the 63.4 constant in the equation. I wonder if that means anything?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Eric.
Maybe it's 2g, maybe not. Those kind of equations was established by the 'ancients', or manufacturers. Sometimes carries with the units coefficients, but others also include empirical factors. If you don't reference from where came the equation, have less chance to obtain some help, but those equations sometimes are so obscures that even with the complete book no one can say from where came those coefficients.
Best regards.
Alvaro.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Possibly, the density of water in lb/ft^3. I've seen it referenced as 62.4 lb/ft^3.
Alternatively, If you search for a worksheet in this collab, Colebrook-Friction in Pipes that may be of interest.
I hope this helps.
Norm
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Equations appear to be from Crane's 4-10 Note Book. At the front of the book the variables are defined with units. The equation in the notebook should be used without units. The conversions are included in the equation. I have run into this issue with other empirical function. One way to resolve the units is define the variable in the units you are using, i.e. delta P in pascals. Divide delta by psi for the equation. This gives you the unitless value, but the correct number for the equation. This approach also works for Mathcad 15 Solve function.
Cheers
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
What you can say for sure is that C.v x 63.4 is an area:

Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
One regular method for comversting these empirical equations into a coherent SI equation (in mathcad) is to, for each variable, divide it the variable by the requested units (within the equation), and then multiply the final answer (which will then be numeric) by the stated output result units.
This works for all types of equation that have the conventional style of units and dimensions. It can fail for advaced phyics where they can use plank units and loose all feeling for scale (every constant is unity, and many convetional 'variables' disappear). Though this works fine for regular engineering calculations.
Luc's method gives a nice feel for what is hidden in that final constant.
If the equation's conversion can be taken in stages it is possible in some cases to re-align the equation to being 'science' with understandable material constants being added.
Philip
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
By basic balancing of dimensions, your 63.4 needs to be an area. (There is also probably a unit conversion factor or 5 thrown in):

The bottom equation would report the same flow coefficient regardless of the units used.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The units are area, but A = 63.4*ft^2. The conversion 63.4 is for US units. Adding the units to the 63.4 will allow the use any units.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
David Tietje wrote:
The units are area, but A = 63.4*ft^2. The conversion 63.4 is for US units. Adding the units to the 63.4 will allow the use any units.
Well, not really. The attached file takes an example from Swagelok, where they calculate volume flow rate of water thru valves, shows why their equation needs to be adjusted for use in unit aware Mathcad, then shows why simply applying units to A in the above equation does not give the correct result.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
It looks like a basic orifice equation, except it needs an area. Regardless, if you read this document (Hint search for N6 factor) you might find your answer with a little legwork.
http://www.documentation.emersonprocess.com/groups/public/documents/reference/d351798x012_11.pdf
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Here's the derivation I came up with for the units. The value changes between 63.3 and 63.4 depending on what standard water density is used.

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Excelent!
Best regards.
Alvaro.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks everybody for your generous help on this question. I'm sorry I haven't been able to interact much lately, but I got busy on another project and had to put this aside. Now, however, I hope to get back to this and read/digest all that has been written, especially the posts from dferry and Fred Kohlhepp. As soon as I am able to do that, I'll let you know how I make out.
I hope you all hade a great holiday weekend!





