Community Tip - Learn all about the Community Ranking System, a fun gamification element of the PTC Community. X
- Community
- Creo+ and Creo Parametric
- Analysis
- Re: Use a measure to calculate force in simulate
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Use a measure to calculate force in simulate
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Use a measure to calculate force in simulate
Hello,
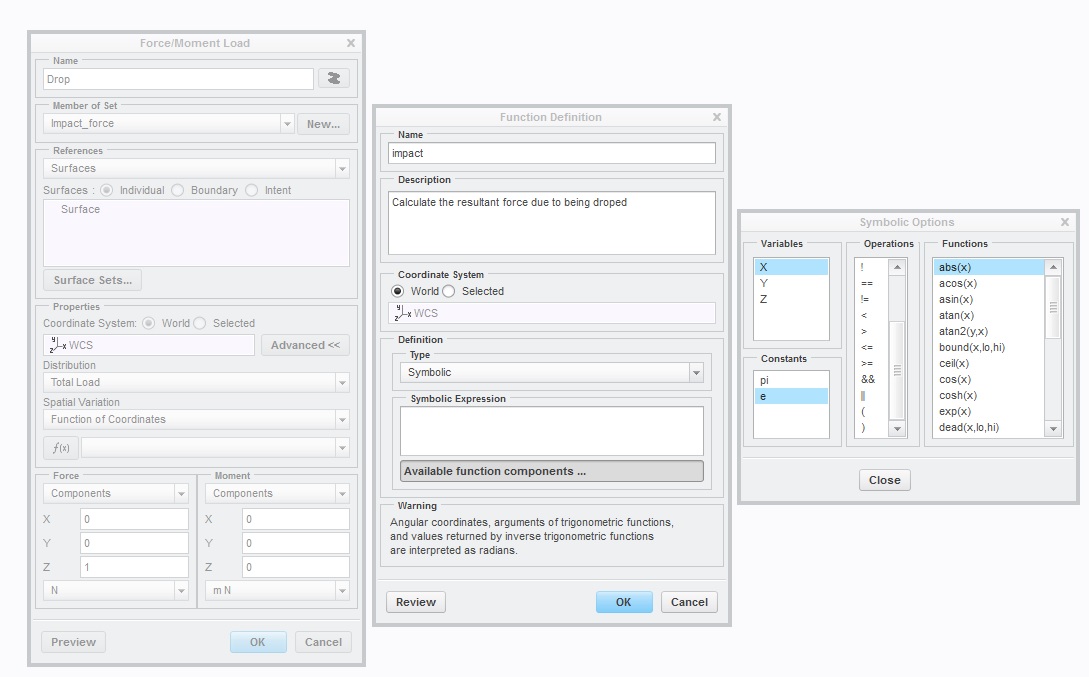
I want to conduct a drop test using simulate. I can calculate the energy on the component when it hits the floor using the formula mgh (mass x gravity x height). However, it's difficult to convert this to impact force. The impact force is given by energy divided by deflection. I need to get the deflection in order to apply force, but I need to apply force to get deflection.![]() . Catch-22, mutually dependent condition.
. Catch-22, mutually dependent condition.
I thought of a solution would be to create a measure for deflection, then use this deflection in a formulae to apply force.
Anyone know how to do this?
Below is the Load as function page in simulate where am trying to do this.
Cheers.
This thread is inactive and closed by the PTC Community Management Team. If you would like to provide a reply and re-open this thread, please notify the moderator and reference the thread. You may also use "Start a topic" button to ask a new question. Please be sure to include what version of the PTC product you are using so another community member knowledgeable about your version may be able to assist.
Solved! Go to Solution.
- Labels:
-
General
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks all for your help. I came up with a solution,
Just to clarify the question. The part am analysing does not come into contact with the floor. It's a sheet metal part mounted inside a rugged plastic case (Pelicase). The sheet metal deflects when the plastic case hits the floor.
My solution was to guess the deflection and use it to calculate the impact force. I then compared the deflection from Creo simulate to my guessed deflection. From this I made a better guess of the deflection and run the analysis again. I did this till I got the accurate deflection.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello,
You could use the Mechanism Dynamic Extension and perform the drop test in order to find the forces acting on your body when it hits the floor.
After that, you can export this loads to simulate and perform a simulation to see the deflections that it causes to your object.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hmm... this is probably stretching the capabilities of Mechanica a bit!
If you assume that your component only deforms elastically (no yielding or fracture), then perhaps you could set up a contact analysis (including a model of the floor with appropriate material properties), probably using a gravity load to represent the maximum deceleration of the component, and iterate the gravity (deceleration) value manually until the Total Strain Energy measure is equal to your calculated m×g×h.
I would strongly recommend using a Quick Check to get close to the right answer, before running a final few analyses using SPA or MPA!
You may also be able to use non-linear materials to simulate yielding, but I don't know what measures are available - presumably you would need to look at the sum of elastic and plastic strain energy?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So, with this
Total Strain Energy measure is equal to your calculated m×g×h.
You are saying that at the critical point, the mgh energy is converted into the Total Strain Energy?
Based on this, defining a FIXED CONSTRAINT to a certain value, you don't need any load at all and you can iterate the Total Strain Energy value to the mgh value. I believe that this is a valid approach also.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If nothing yields and the deformation is purely elastic (both component and floor) then yes, I believe that all the energy should be converted from kinetic to strain at the instant of maximum deformation (and therefore maximum force, and maximum de/acceleration).
I'm not sure that you can do it purely with constraints though, as it's a transient, quasi-static situation in which the reaction force from the floor is in equilibrium with the mass × acceleration of the component and therefore the 'constraint' is distributed over the entire mass of the component (which will have a varying displacement throughout). You can do it either with a force and inertia relief (possibly), or with a constrained floor and a gravity (= acceleration) load (more reliably), I think.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks all for your help. I came up with a solution,
Just to clarify the question. The part am analysing does not come into contact with the floor. It's a sheet metal part mounted inside a rugged plastic case (Pelicase). The sheet metal deflects when the plastic case hits the floor.
My solution was to guess the deflection and use it to calculate the impact force. I then compared the deflection from Creo simulate to my guessed deflection. From this I made a better guess of the deflection and run the analysis again. I did this till I got the accurate deflection.





