Difference method for an initial value problem

[-MFra-]

Is there a person who kindly would like to dedicate some time to my little problem? I would like to know if the difference  method  and the amplification matrix are accurate.

Thanks so much.

F. M.

[sm�ller-2]

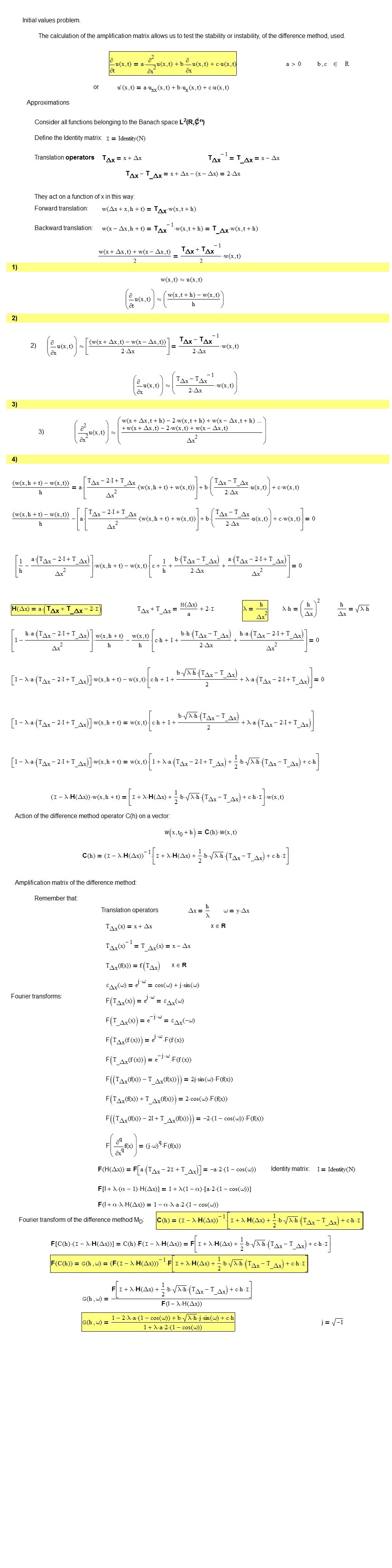
Is it really correct to introduce the "h" from Chapter 2 to Chapter 3?

d[u(x,t)]/dx = ...

d2[u(x,t)]/dx

its normal: u(x+delta x,t)/d2x + u(x- delta x,t)/d2x -2*w(x,t)

you did not use h in chapter 2 in the first order

why ad it in the second order

Out from wikipedia:

https://www.google.at/search?q=differenzenquotient+h%C3%B6herer+Ordnung&biw=1920&bih=947&source=lnms&tbm=isch&sa=X&ved=0…

[-MFra-]

The first side of the equation,  is the time derivative of w(x,t), while the second member is constituted by the derivative of w(x,t) with respect to x. Furthermore, h is the time increment.

However I redo a review of what I have done.

Greetings

F. M.

[-MFra- · Accepted solution]

[sm�ller-2]

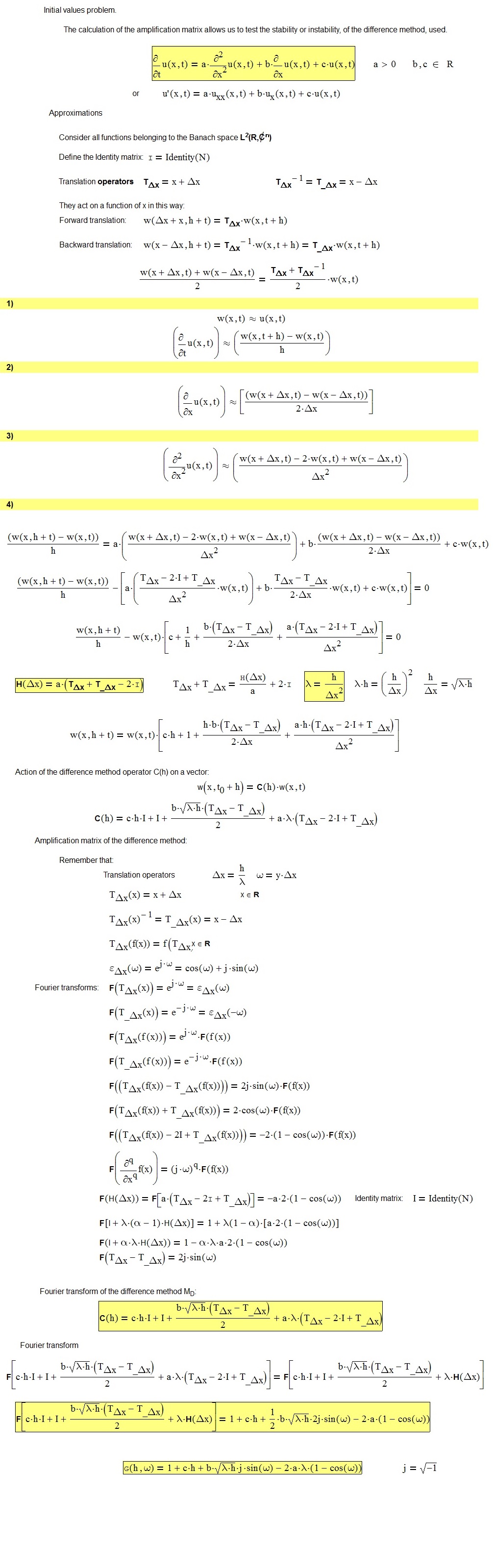
Hmmm the h is now gone away in the second order which seems to be logical for me in Chapter 3.

OK Forward and backward translation is understandable for me but after you define forward and backward translation (t+h)

you are saying:

(w(x+dx,t)+w(x-dx,t))2 = T(dx)+T(dx)^-1/2 *w(x,t)........  BUT Without time increment / That is inconsistent with the upper 2 Definitions with h

Maybe im wrong 🙂

Chapter 4

Translation operator:

i think it should be this way: delta x = sqrt(h/lambda) as defined before in the equation transformed to delta x

Or it is a new definition i dont know.

Rest is too much maths for me  

Greetings

[-MFra-]

[sm�ller-2]

Ok thx for the posts. Trying to understand. Will need to use my math Book 🙂