Community Tip - If community subscription notifications are filling up your inbox you can set up a daily digest and get all your notifications in a single email. X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Expanding equations in Solve block turns values to 0
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Expanding equations in Solve block turns values to 0
I have (6) equations with (6) unknowns that I have in a solve block. In addition to showing the equations I am using, I have to show the equation with the known values shown
For example, the fourth equation is:
(V.S2) = (I.2) * (Z.L2)
I have put the variables in parenthesis to indicate the entire name for the purpose of the discussion.
The variables (V.S2) and (Z.L2) are already pre-defined. I would like to show the same equation next to the first euqation with the values of (V.S2) and (Z.L2) shown. When I try using the "simplify" or "expand" or "-->" next to the equation, the values in my results change to 0.
I have attached the Mathcad file. Going into the solve block and trying to expand the equation will cause the values in my results to change to zero. Can someone please provide some guidance? Thank you.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Sorry, the symbol math of Mathcad does not know units - ohm, V, deg.
See the attach
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Valery nothing attached.
Mike
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
As Valery indicated the symbolic engine doesn't deal with units, see the attached is of help.
Mike
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Again Valery, nothing is being attached.
Mike
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
1. Now in Mathcad Prime we can have in one vector volt and ampere
2. It is a system of LINEAR Equations and we can use the lsolve function
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
@ Mike: If the variables did not have units, could I use the symbolic engine to expand on the equations?
@ Valery: In Mathcad Prime, would the Isolve function allow me to work with units and expand the equations?
Thanks in advance.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
@ Mike: If the variables did not have units, could I use the symbolic engine to expand on the equations?
You can tell to symbolic Math of Mathcad that V =A*ohm
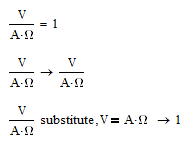
@ Valery: In Mathcad Prime, would the Isolve function allow me to work with units and expand the equations?
Thanks in advance.
The Isolve function of regular Mathcad allow you to work with units of one dimentions.
The Isolve function of Mathcad Prime allow you to work with units of different dimentions.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Do you mean this?

Because if this is what you mean, I don't see the 0's.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
May be so:
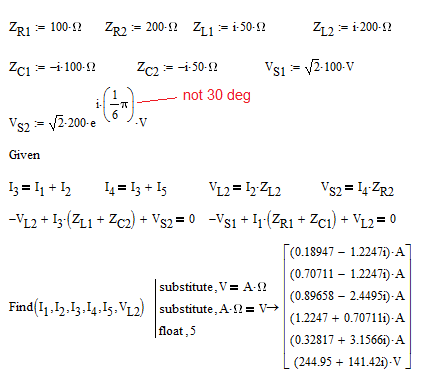
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
This is one of the few tasks where Mathcad Prime has a distinct advantage over traditional Mathcad: the solving by the help of the lsolve function or by the help of the operator A^(-1)*v a system of linear equation A*x=v where arrays A, v and x have elements with different dimensions - Potential and Current!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
This is one of the few tasks where Mathcad Prime has a distinct advantage over traditional Mathcad: the solving by the help of the lsolve function or by the help of the operator A^(-1)*v a system of linear equation A*x=v where arrays A, v and x have elements with different dimensions - Potential and Current!
Massive, massive improvement IMO.
Mike





