- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
New problem with a catenary – a specific pendulum
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
New problem with a catenary – a specific pendulum
New problem of the catenary – a specific pendulum.
We loaded our catenary with weight and looked like changing its form – see please here http://communities.ptc.com/videos/4438. If the weight is too heavy, then either Mathcad not be able to solve a system of equations or a catenary... breaks and we have two parts as a… pendulum. I tried to simulate the swing of this pendulum-chain with five units - see please http://communities.ptc.com/videos/4448
The problem we have is not a chain, and a weighty chain is flexible, which we rejected from the vertical to the desired form (with what desired form?). And released. How to simulate the swing of this pendulum-chain? Is there a symbolic solution of this problem. There is solution is for the ordinary pendulum.
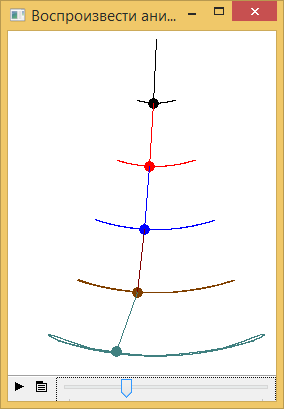
ЗЫ
"Knowledge will break the chains of slavery"

- Labels:
-
Other
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
An animation for 10 ball - see the attach too!
What is the best initial position of balls? The catanery line?
Can we create a sheet with infinity number of balls?
Etc...
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Your 10 mass pendulum has (if my accounting is right) 55 different degrees of freedom; the motion solution has multiple answers that will depend on the initial displacement arrangements.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks, Fred.
The problem is very interesting. I can not find something about it in Internet ![]()
One real video:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Fred Kohlhepp wrote:
Your 10 mass pendulum has (if my accounting is right) 55 different degrees of freedom; the motion solution has multiple answers that will depend on the initial displacement arrangements.
One initial displacement arrangements:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
There's a large amount of analysis for compound pendulums; your problem has the additional complication of flexible links but that might be a place to start. (A Lagrangian analysis seems to be a favorite starting place.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks Fred.
One general question.
There is a mathematic pendulum see for example here
Can we say about a mathematic pendulum-catenary? About its period etc.
PS
A mathematic (a=sin a) and physical Pendulum solutions in Maple:
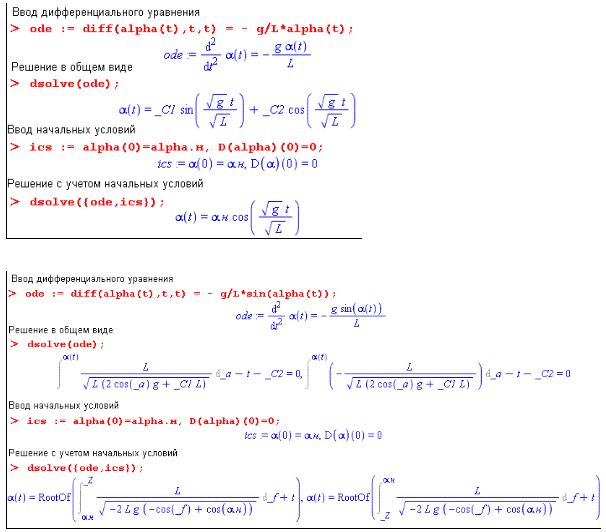
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Fred Kohlhepp wrote:
Your 10 mass pendulum has (if my accounting is right) 55 different degrees of freedom
Does my catanery-pendulum have an infinity degrees of freedom?
I feel this problem has a simple solution by specisic initial position of athe catenary.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Combine a pendulum with a vibrating string.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Fred Kohlhepp wrote:
Combine a pendulum with a vibrating string.
Like this http://communities.ptc.com/videos/1357 ?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Daniel Bernoulli has solved this problem in 1732 (in Russia ?) - see http://librarum.org/book/5372/689
But now we can try to solve it numerical without constrains. In Mathcad. With animation.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
One fine pendulum lesson in the Amarcord by Federico Fellini!
The teacher has a catenery (chain) on his stomach for new demonstration ![]()

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Actually, as demonstrated by the segment of cord between the professor's hands, this is a catenary. The relative mass of the "chain" to the weight is such that it's not visually obvious, but the "pendulum arm" is flexible.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Fred Kohlhepp wrote:
Actually, as demonstrated by the segment of cord between the professor's hands, this is a catenary.
Ops - one more catenary - Thanks Fred!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator





