Solving an Equation

[JayVattappilly]

Hi

I am trying to slove an equation using the solve command and unable to get the answer. A template is attached.

Can someone please help

[AlanStevens]

Use a Given ... Find solve block. That is put Given before your equation and Find (N.f)= after it. Provide an initial guess for N.f before the word Given.

However, you first need to get the expression correct. The first term on the right-hand side has units of 1/Pa, the other two terms have units of Pa.

Alan

[Werner_E]

However, you first need to get the expression correct. The first term on the right-hand side has units of 1/Pa, the other two terms have units of Pa.

No, the units on the RHS depend on the value of the exponents, the values of b,c. Thats the reason it won't solve numerically, too. Guess the equation has errors or is kind of an empiric formula.

[Werner_E]

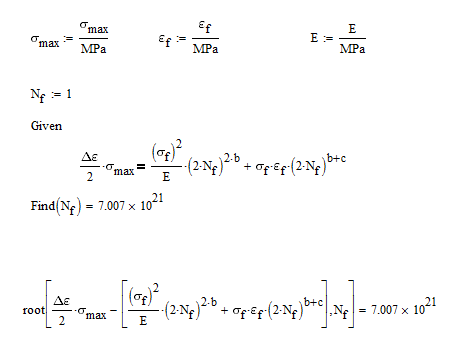
There are some errors:

1) Wrong spelling of "solve"

2) The equation and the solve command are in different regions!

3) Probably Mathcad can't solve that equation symbolically. Use a numerical appraoch (root or a a solve block)

4) Unit problems: N.f^(2b) should yield pressure^2 and N.f1(b+c) should yield dimension pressure for the equation to be unit consistent. This would mean that c=0 which does not correspond to the value you provide.

So I guess you have to check your equation.

If its some kind of an empiric formula, you can solve it by stripping off the units.

See attached a way to do it with a solve block and another using the root command. Hope it helps.

[AlanStevens]

Werner Exinger wrote:

There are some errors:

4) Unit problems: N.f^(2b) should yield pressure^2 and N.f1(b+c) should yield dimension pressure for the equation to be unit consistent. This would mean that c=0 which does not correspond to the value you provide.

I agree that Nf^2b would have to be pressure squared, but Nf^(b+c) would have to be dimensionless (because sigmaf*epsilonf has dimension pressure). That (and the fact that sigmaf^2/E is - rather neatly! - 1/Pa) is why I assumed Nf would be dimensionless.

However, I think we agree that, one way or another, the equation as it stands is either incomplete or in error.

Alan

[Werner_E · Accepted solution]

AlanStevens wrote:

Werner Exinger wrote:

There are some errors:

4) Unit problems: N.f^(2b) should yield pressure^2 and N.f1(b+c) should yield dimension pressure for the equation to be unit consistent. This would mean that c=0 which does not correspond to the value you provide.

I agree that Nf^2b would have to be pressure squared, but Nf^(b+c) would have to be dimensionless (because sigmaf*epsilonf has dimension pressure). That (and the fact that sigmaf^2/E is - rather neatly! - 1/Pa) is why I assumed Nf would be dimensionless.

However, I think we agree that, one way or another, the equation as it stands is either incomplete or in error.

Alan

You are right - I overlooked the unit of epsilon.f and I guess its wrong - it should be dimensionless.

I guess you that N.f should be unitless as probably N.f is the number of cycles needed for a crack to grow a specific length. It looks to me now that epsilon.f and sigma.f get mixed up - at least concernung their units. epsilon.f should be a unitless coefficicient and sigma.f as stress should have dimension pressure. This would straighten all out.

[AlanStevens]

Yes, that looks a lot more sensible Werner! I guess we'll have to await confirmation or otherwise from Jay to be sure.

Alan

[JayVattappilly]

Dear Werner and Allan. Thanks for all the help and the final version that Werner uploaded really worked. This equation is the Smith Watson Tooper Mean Stress Correction equation and I was checking the math from the strain -cycles curve versus the equation. Werner is absolutely right that units of epsilon f and sigma f got mixed up. One is strain and shouldn't have any units and the other is stress and should be in MPa.

It is working now and thank you so much guys for all your help

Jay