Skip to main content
R
Raiko17-Peridot
17-Peridot
August 3, 2017
Solved

Weibull fitting problem

  • August 3, 2017
  • 6 replies
  • 7614 views

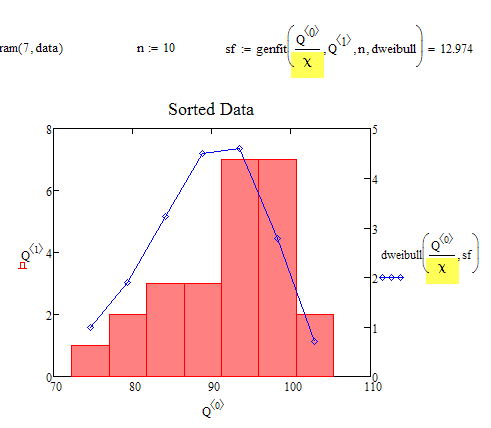
Can anybody point me to my error. I can't get a decent fit for my data; see attached MC15 file.

 

Thanks in advance

 

Raiko

 

P.S. For some reason the website does not accept the MC15 file claiming that "...the contents of the attachement doesn't match its file type." henec, I've added a pdf.

Best answer by Werner_E

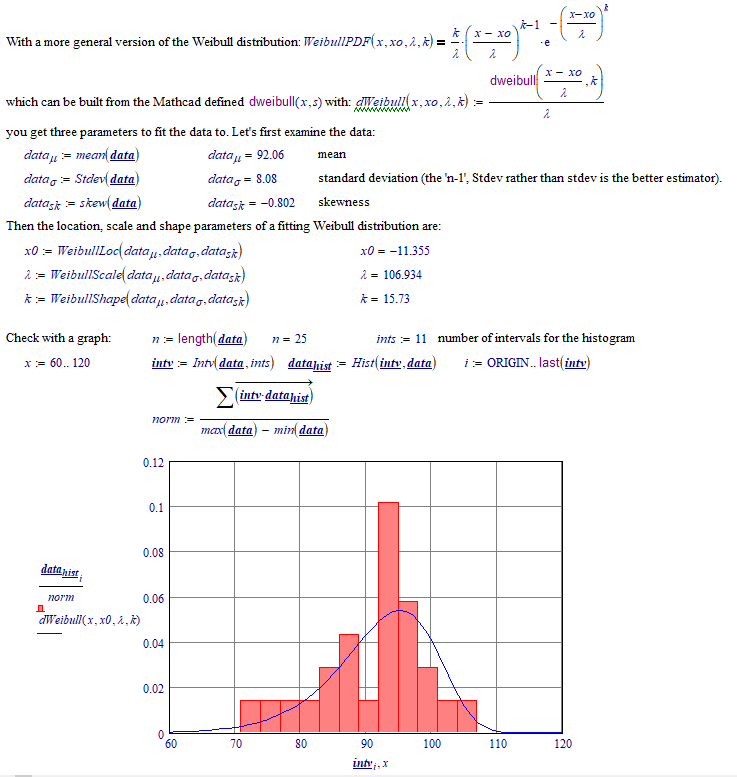
I am not sure about the Gaussian.

Here is a direct comparison side by side (worksheet attached):

Pic.png

 

6 replies

L
LucMeekes23-Emerald IV
23-Emerald IV
August 3, 2017
Attachments is a known problem that is being worked on. You can attach if you first put the worksheet in an archive (.zip).
Luc
R
Raiko17-PeridotAuthor
17-Peridot
August 3, 2017

OK Luc, here we go

 

Raiko

-
-MFra-21-Topaz II
21-Topaz II
August 3, 2017

Hi,

The data aren't distributed according to the Weibull distribution that you have traced in your graph (for s<=1). So you will never be able to adapt them to Weibull's distribution. The sample is small. How do you say it is distributed according to Weibull? With a larger sample it may turn out to be Gaussian.

weibull.jpg

W
Werner_E25-Diamond I
25-Diamond I
August 3, 2017

Maybe a scaling problem

Pic.png

    M
    mvenich14-Alexandrite
    14-Alexandrite
    August 3, 2017

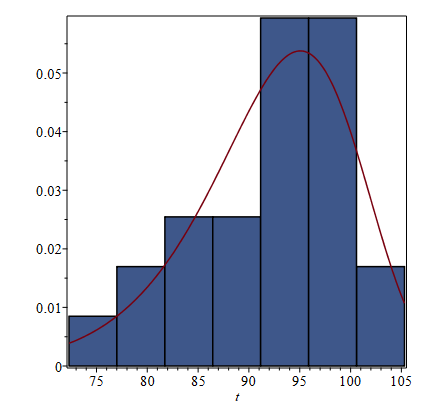
    Here's a PDF of what Maple gives you

    wb.png

    W
    Werner_E25-Diamond I
    25-Diamond I
    August 3, 2017

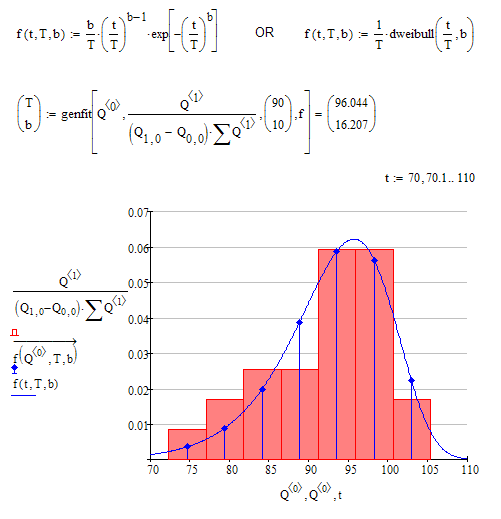
    Mathcads weibull unfortunately is standardized to scale parameter (in German: charakteristische Lebensdauer) T=1.

    To fit we need a function with two parameters, scale T and shape b.

    Guess thats the way to achieve that in Mathcad:

    Pic.png

     

     

      F
      Fred_Kohlhepp23-Emerald I
      23-Emerald I
      August 3, 2017

      F M is (I believe) correct, This should be a normal fit; if you're measuring hardness that's what I'd expect.  You can get a normal fit as shown--the same basic method as Werner did for the Weibull.

      W
      Werner_E25-Diamond IAnswer
      25-Diamond I
      August 3, 2017

      I am not sure about the Gaussian.

      Here is a direct comparison side by side (worksheet attached):

      Pic.png

       

        R
        Raiko17-PeridotAuthor
        17-Peridot
        August 9, 2017

        Hello Werner,

         

        sorry for the late reply but I wasn't in office for a few days. Thanks a lot to all of you guys for your effort and thank you Werner for the solution.

         

        Raiko

        L
        LucMeekes23-Emerald IV
        23-Emerald IV
        August 4, 2017

        Your data is skewed, hence a Weibull distribution is more plausible than a normal distribution.

        WeibullFit.png

        Success!
        Luc

          F
          Fred_Kohlhepp23-Emerald I
          23-Emerald I
          August 4, 2017

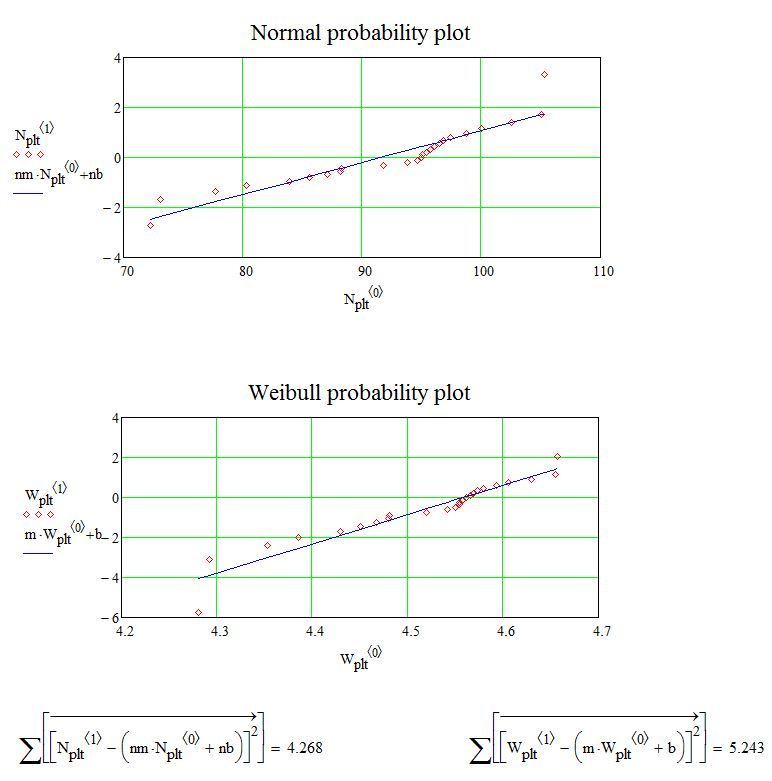
          Not sure that I agree.

           

          Using the probability plotting of the Data Analysis Extension for both Normal and Weibull:

          comparison of distribution fitscomparison of distribution fits

            R
            RichardJ19-Tanzanite
            19-Tanzanite
            August 5, 2017

            There is no realistic way to know what the distribution should be, and 25 data points is surely not going to allow you to determine it statistically (although I have not done any statistical calculations to prove that Smiley Wink). Maybe there is some systematic error that skews the hardness measurements, maybe not. If not, it's probably Gaussian. If there is such an error, it probably does not follow any statistical distribution. So what distribution to use in the fit likely depends on the end goal more than anything else.

              Terms & ConditionsCookie settingsAccessibility statement