Drawstring bag design

[joe_c]

Folks

i need help modeling a drawstring bag in its relaxed and compressed position. I figured the top section can be modeled using trajpar or helical sweep. The bottom with surfaces and will connect together using merge, trim, etc. Would anyone share a cad model that resembles this

thanks

[Pettersson]

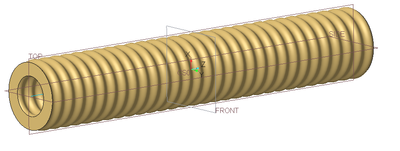
Here's a quick idea. First is a Curve from Equation with a cylindrical coordinate system. Equation:

theta = t*360
z = 0
r = radius+sin(t*360*20+90)*variance

I used radius 50 and variance 5 for my example. I use parameters for these, as I'll explain below.

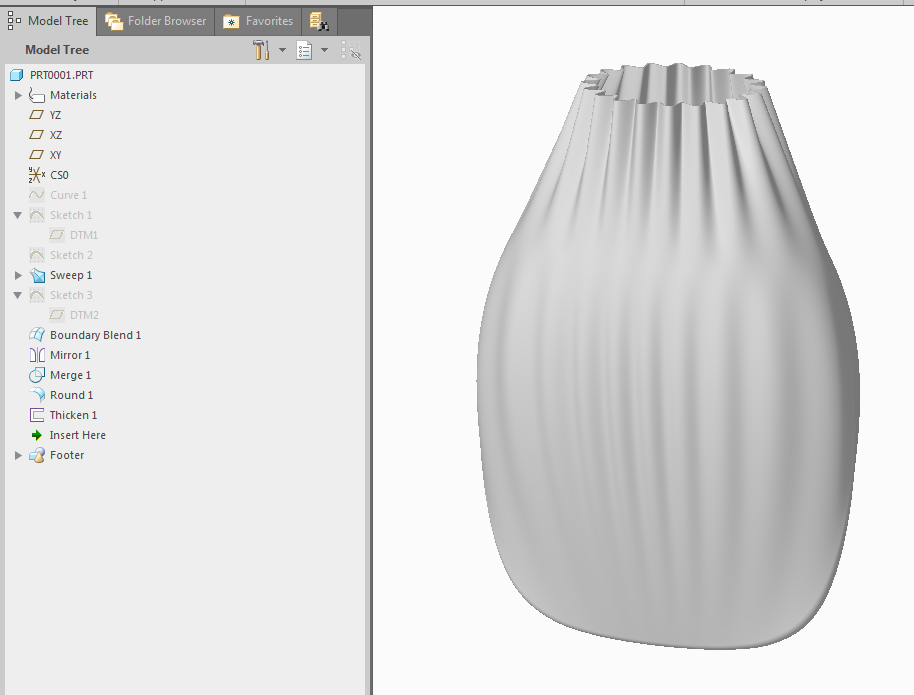
Adapt measurements as needed. Inside is a circular sketch which is only used as the sweep origin. Lower down, there's an oval sketch. Make a surface-only variable section sweep connecting a line between the wiggly line and the oval. Make sure to use the circle as origin, so the sketch doesn't change angle as it goes around.

Then I made a single line further down, and connected it to the side surface with a tangency requirement and mirrored that.

Finally merged it all and made a round to make it less pointy at the bottom. Then thicken. You could play around some more if you want a different shape below. Result as above.

I then made a family table controlling the radius and variance. I increased the radius to 80 and set the variance to 0, which gives you a nice circular opening to show an open version of the bag. Result as below.

It's not super pretty, but it's a quick job. If you want it more realistic-looking, the Freestyle tool might be your best bet. Sorry, can't upload the part from here.

[joe_c]

Thanks Pettersson

Is the origin sweep superimposed on the equation (wiggly) curve? In the VSS, i tried to sketch the section as a vertical line between the origin-oval and as well the equation-oval and it produces a cone.  In VSS, whats the section looks like? 

[Pettersson]

There are three sketches: the equation, the origin (a circle) and the oval. When making the VSS, select the origin first, then the other two. Then draw a straight line from the equation curve to the oval. The origin isn't really part of the sweep; it's just used to set the normal direction of the sketch. Therefore it doesn't matter where it's placed or how big it is; it just needs to be a perfect circle. Hope that helps. I can't upload a part, but here are some screenshots.

In the final two, instead of a straight line, I did a spline and constrained it to being vertical at the oval. This stops the ripples from propagating into the boundary blend.