Community Tip - Want the oppurtunity to discuss enhancements to PTC products? Join a working group! X
- Community
- Creo+ and Creo Parametric
- Analysis
- Components for Stress or Strain
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Components for Stress or Strain
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Components for Stress or Strain
Hi everybody,
I want ask you where and why one need to use Max, Mid, Min or Max Shear principal components of Stress/Strain.
How can I utilize them to understand if a component, in a particular point, will resist or not?
I attach two pics where I want to analyse a specific point. One is the vectorized display of the three components. One is the fringed display of the Max,Mid,Min and Von Mises Stress. Then I post also a sheet where I compare Von Mises tension from principals tensions manipulation and directly from Simulate.
I've a little bit confusion in my head redarding this argument. Could you let me understand better the point?
In my opinion principal tensions are not useful with fringe plot, but only with vector plot, but which one should be considered?
Thanks
Bye
- Labels:
-
Simulate
- Tags:
- components
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You are touching on the area of what criteria to use for a failure.
Different materials fail in different ways according to the full stress state. The Von Mises criteria is just one such theory for failure, but it is just a theory and works great for some materials and load cases, yet does not work very well at all for others.
I like signed Von-Mises which differentiates between compressive and tensile failure. This can be accomplished in CREO using failure criterion of Modified Mohr with different tensile/compressive failure stresses. The fringe plot is then "failure index" The failure index can be confirmed by looking at the Max/Min Principal stresses and doing a ratio to the tensile/compressive material property failures respectively.
Also, nearly the same information regarding stress is shown between fringe and vector plots. Min principal shows the compressive, and max principal shows the tensile, The fringe plots give the vector arrow magnitudes and signs (two plots to show). The only thing not shown by a fringe plot is the orientation of the vector which is best shown by a vector plot. I would think crack propagation cares about the orientation, but I would not see how the origination point of failure would depend on orientation much if any.
This related answer includes references that show more on how and when these stress components might be used. See this answer
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So:
- Max Principal shows the tensiled points
- Min Principal shows the compressed points
- Modified Mohr criterion is to use where you have material, like concrete, that can collapse also in compression
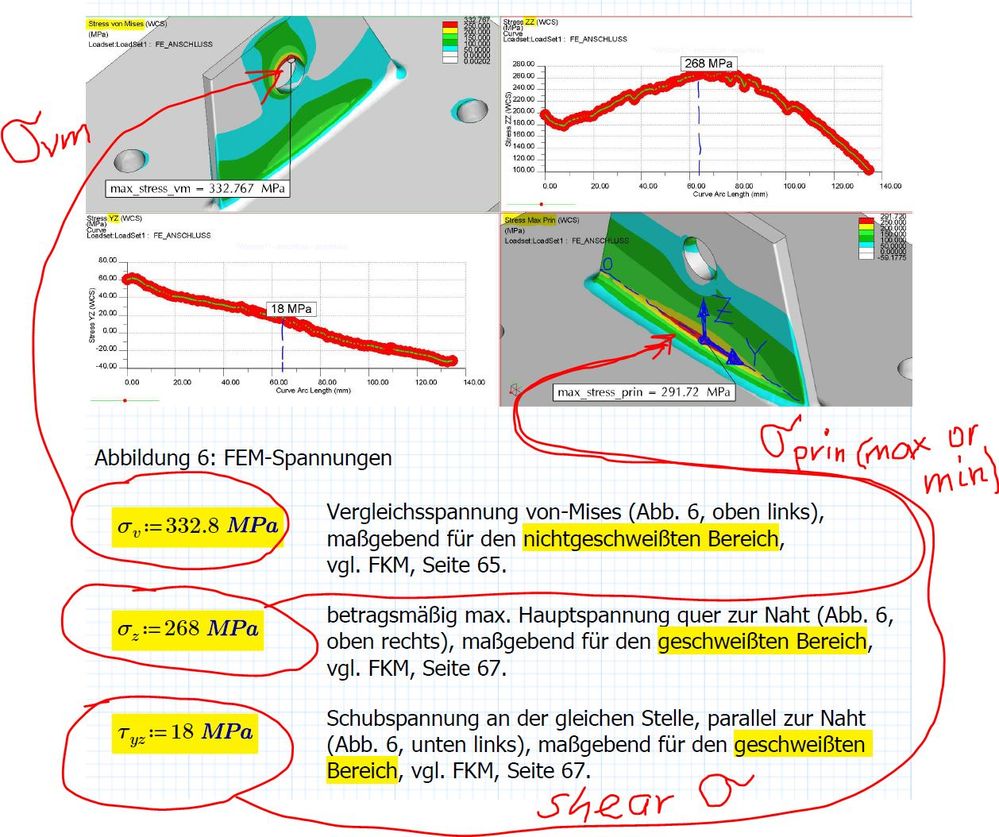
@skunks If I've translated well from german, in the example you are gone to find the normal stress (along Z) and the shear (along Y) to use them subsequently in the welded section calculus.
So XX,YY,ZZ stress are useful to see what happen in a particular section. But how can I use usefully Max and Min stresses?
I attach two new pics where I show the assembly (the zones fixed and the ones loaded) and the fringe plots of max and min principal stresses.
I can't understand the plots. For example, in the Min principal plot: why the blue zone (that It should be compressed being where the mass acts through the weighted link) is blue, so negative? If Min principal mean "compressed", a negative compression is a positive tensile.
???
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Negative stress is always in compression and positive stress is in tension regardless of MAX/MIN principal being shown. So your MIN principal could be tensile. For principal stress space by definition MAX >> MID>> MIN. In principal stress space the stress state matrix is rotated to show where the max possible stress is and then the other 2 orthogonal axis stress states are ordered according to MAX>>MID>>MIN. It is essential like XX YY ZZ only rotated to the max direction (maybe the max tensile is at 45 degrees to XX) and then ordered from max to min. (sort of like XX ZZ YY = MAX, MID, MIN because XX was highest then ZZ then YY)
For concrete for example, the more compressive MID and MIN are, the higher the magnitude MAX principal tensile stress can be before failure. Vise- versa. The more MID and MIN are also tensile, rather than compressive the lower magnitude MAX principal can be before failure occurs. Basically a compressive "hydrostatic stress" increases the strength of concrete and a tensile "hydrostatic stress" decreases the strength.
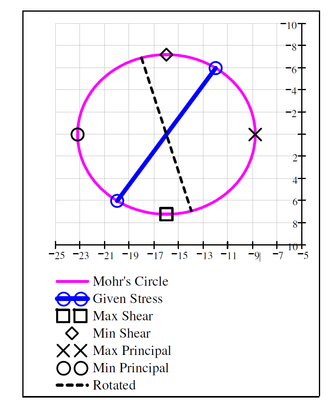
Here is an explanation with Mohrs circle. This is for planar (2D) stress to simplify.
Ignore the "rotated" line. The 2D stress state given in some world coordinate system - (blue) is the same but the reference coordinate system is rotated to give the max/min principal, max/min shear stress. Thus there is a new local principal stress coordinate frame. The vector plots show the orientation based on how much the angle is between the world coordinate system and the principal stress coordinate frame.
Maybe another way to think of this is this example.
Two observers, one looking north, one looking east, observe a car appearing to travel west at 60mph and south at 60mph respectively. The car is actually travelling at 84mph to the south west. In a similar way stress in X direction and Y direction add to a larger stress in the principal direction. It only might appear smaller because of the reference frame, not being in the principal direction.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So what I've obtain in the previous MC Sheet should be right and the VM stress (calculated with the formula with the three principal tensions) should be egual to the one directly given by the program. But this is not.
VM doesn't discern between tensile or compress state, being a mean. If I want to see if a zone is in tensile or compress state, how can I do? Because if I see the Max Principal I see only a part of the stress, being the real stress dues to the set of three Principal Tensions.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I have an idea that might work. I'm not on my Creo machine at the moment.
Try using modified mohr with compressive failure set to -100000 (large) and tensile set to 1.0.
Thee failure index results should then only show tensile stresses and they should be in proper stress units because they are ratio. Vise versa might only show compressive stresses. Not sure about this but if it works I will definitely use this idea often because my customers do not like or understand failure index plots as ratios to yield stress. This way I could show then in stress units.
Your stress components either in X,Y,Z or in principal space should compute to match the von Mises ctriteria.
If you were just inspecting fringe plots, instead try putting measures for all the stress components at a single point of interest and then doing a user defined measure with the manual conversion to von mises and compare to a measure using CREO von mises.
see the summary table in the wiki article. You would use one of the first two rows (general or principal space)
but for general you need 3 axial and 3 shear stress components.
https://en.wikipedia.org/wiki/Von_Mises_yield_criterion
If your calculations then do not come very close to Creo stated von-mises stress then I share your concern as to why.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Before trying your suggestion I attempt a different way; I've seen the zones where the material was in contraction thanks to the deformed fringe plot. So I've taken values of max and min principal stresses in that points to calculate the Tresca Stress by myself.
Then I try to use "your method" (PS: I known the Modified Mohr criterion as Rankine Criterion), but it is for brittle material and I have steel (S235).
However I tried but some areas don't appear, but I know that were under stress.
So I tried the Tresca Criterion and I find corrispondency with what I done first (by hand...ehm that is MathCad ![]() )
)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
That is interesting how that stress does not appear. Modified Mohr should show more stress than Tresca or Von Mises so I guess it is the limits affecting the failure index. Did you set C failure to very slightly more magnitude than T failure, or did you set it very large? If those areas are in compression stress then a large value for C strength would mean low failure indices in compressively stressed areas.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
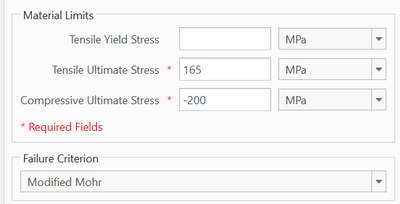
This is how I set the material and this is what I obtained by Simulate as failure index.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
" If I want to see if a zone is in tensile or compress state, how can I do?"
By using 360 and -1000 the areas in compression are no longer high failure index. So Mohr result now shows areas in tensile with emphasis. Those stress areas that no longer show in plots are in compressive state.
If you are treating as ductile material, why do you need to know tensile vs compressive?
Is -1000 what is expected for compressive failure stress?
If this ductile, we expect C approximately -T for ductile and VonMises or Tresca as criteria.
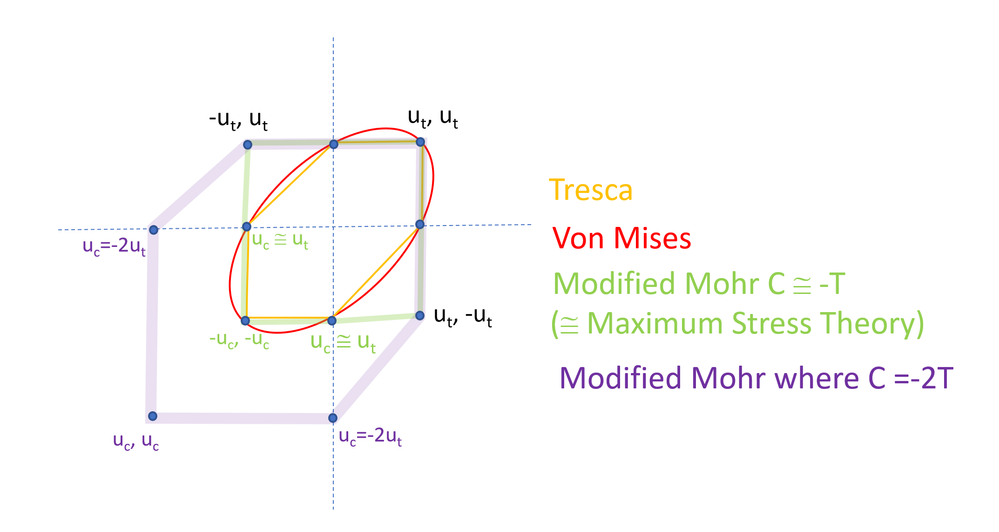
Try this explanation of failure theories.
Also, correction, Modified Mohr with C approx -T is the same as Tresca except for stress states in corner of 2nd and 4th quadrants. There it is less conservative than Tresca.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You're right. I attach new pics for material definition and failure index fringe plot.
I putted high negative value following previous suggestion "Try using modified mohr with compressive failure set to -100000 (large)".
My objective was to investigate areas where VM stress exceeded Sy and understanding the stess state.
- Tags:
- Mohr