Community Tip - New to the community? Learn how to post a question and get help from PTC and industry experts! X
- Community
- Creo+ and Creo Parametric
- Analysis
- Re: Simulation of squeezing a hollow ball
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Simulation of squeezing a hollow ball
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Simulation of squeezing a hollow ball
Hi,
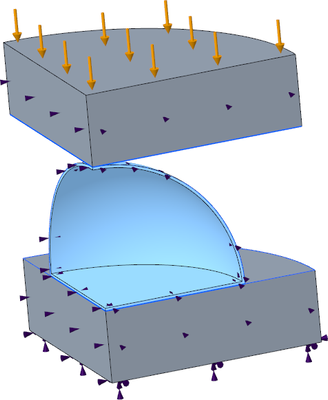
I'm interested in simulating the squeeze of a hollow hemisphere.
I'm unable to run this simulation because there is only one element (the tip of the ball) at the start, and other elements come into contact with the squeegee as it moves.
I attached the model, and I'm not sure what I'm doing wrong?
I'm grateful to anyone who can help in advance.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Andra
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi @achirila
My topic is self-explanatory, and all necessary setup appears within the model.
In the topic, I also discussed the gap, which occurs when the ball is squeezed and forms an interface between the hemisphere's elements and the pressing face (gray).
Thanks
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi @ProFeature ,
This is a most difficult situation for Creo and involves extreme nonlinearities including snap-through. Once contact is defined you cannot select snap-through analysis in Creo. The material you defined for the dome is also extreme E=2MPa, nu = .499, rho = 1E10 g/cm^3 (unit issue) and looks like something that should be modelled as a Hyperelastic material. These all add difficulties however when I initially ran in 3D using Polypropylene the analysis worked fine. Your attached model had no simulation model information so I am guessing you did not grab the highest numbered Creo file. Here are my notes:
1. Use enforced displacement instead of a load. The load can be an output measure. I used Y = -5 enforced displacement. This way you do not have to guess what load is reasonable to apply.
2. Sometimes starting contact condition makes all the difference. Some models like starting with clearance, some just contacting, and some with initial penetration.
3. I like to override contract penetration to 15% (but depends on element size) This relieves the contact calculation difficulty.
4. I had some success with element sizes 4mm on steel 0.5mm on dome.
5. Non-linear options, large displacement and 41 or 81 master steps. Due to the thin model you need to make sure the timesteps are small enough that the contact condition is not stepped past. In theory you may be able to have a few smaller steps then increase to larger ones to make the simulation faster but I generally space them evenly. The point in the deflection that the center of the ball starts pulling away from the pusher may need tighter steps as well.
A progression I have had some success for the first few steps is 0.001,0.003,0.01,0.03,0.1
6. 2D Axisymmetric model is the fastest solving.
7. Adding a small amount of friction can help some situations by stabilizing the solution (adds damping)
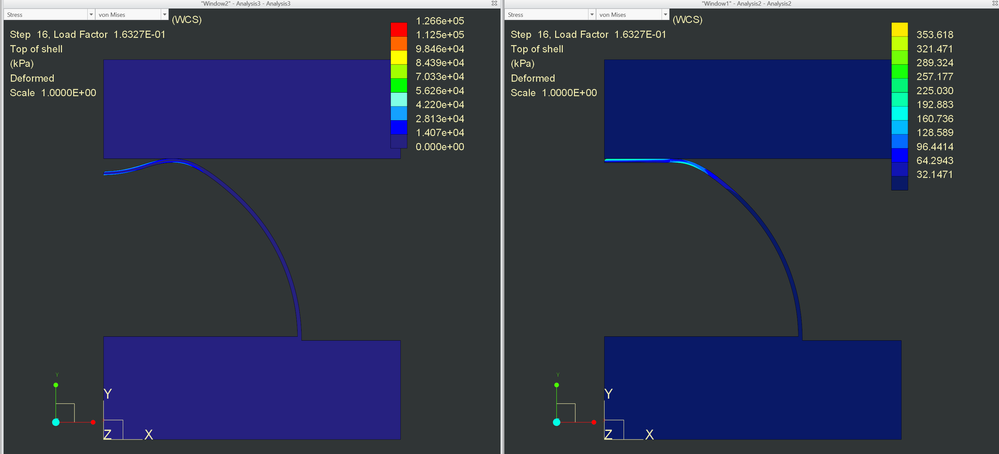
Left image with DOME in PP E= 1GPA material can simulate. Right image with hyperelastic around E=2MPA fails simulating due to snap-through at the shown deflection and about 0.13N load.
Also, a real dome like this would need an air hole, otherwise a Multiphysics model using the ideal gas law is needed.
For contact with snap-through we switch to an explicit FEA code from another vender.
-regards
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you for the detailed explanation.🙏
Can you share the model that uses the 2D axisymmetric approximation?
-Thanks
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator