Community Tip - Have a PTC product question you need answered fast? Chances are someone has asked it before. Learn about the community search. X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
AC induced voltages on buried pipelines.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
AC induced voltages on buried pipelines.
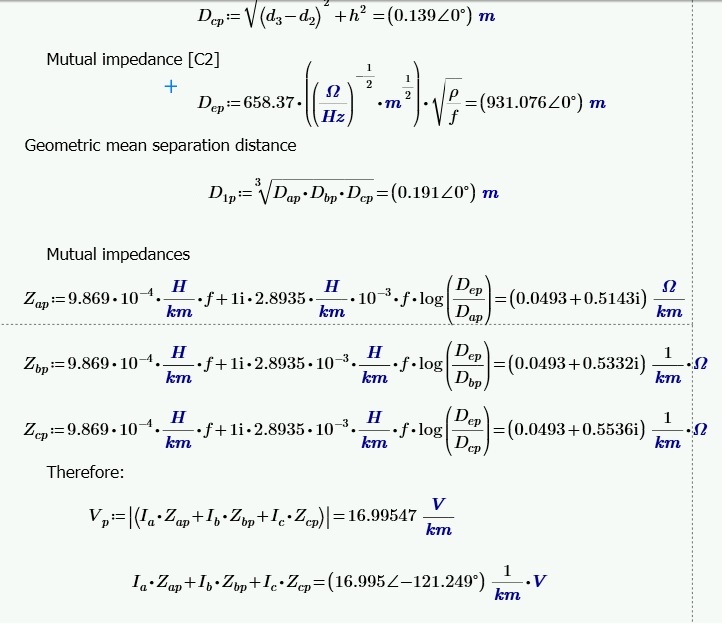
I am struggling to show the result of the calculation in terms of the voltage and the phase angle (in degrees). Vp calculation is correct but it does not show the phase angle. The phase angle should be -121.4 degrees as shown on the sample calculation at the end of the works sheet. I can produce the correct result in Matlab using the rad2deg function ( [abs(Vp) rad2deg(angle(Vp))] ) but I cannot find an equivalent function in Mathcad. Any guidance gratefully received.
Thank you.
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If you take the absolute value it calculates the magnitude and you lose all the phase information. Get rid of the absolute operator and just format the result (on the math formatting tab) to magnitude and angle:

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
X simply divide the expression you want to plot by deg should do the job.
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you for the prompt reply. I don't understand your response because If I divide Vp by 1 degree I do not get 16.9 and a phase angle of -121, which is the correct answer. I get -505.147 and -832.498. At this stage I am not interested in plotting it, just calculating it.
The real element (Re) is -8.816 and the imaginary element (Im) is -14.53
If I use the < symbol for the phase angle then the answer I wish to achieve is 16.9 < -121.4
Kind Regards
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If you take the absolute value it calculates the magnitude and you lose all the phase information. Get rid of the absolute operator and just format the result (on the math formatting tab) to magnitude and angle:

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you Richard. I did know about that feature at all!
Kind regards
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I noticed that you didn't use units, which work quite well in Prime; as an example:

You may want to try it in your worksheet to clearly communicate your application's attributes.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you Norm. Usually I am a stickler for units but on this occasion there is always an error because the constants in the formulae have some units associated with them but they are not stated. I can cancel them out by adding some fudge units in but for the moment I am happy to leave them as they are. Later on I will find the original derivation of the formula and (Carson circa 1926) sort the units out.
Kind Regards
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Sorry if I dare to intervene in the discussion now ended. What I want to point out is that using the units, the results are the same, although it is not very clear what unit has the constant Dep (but if the argument of the logarithm must be dimensionless, it follows that, having the constants Dab, Dbp, Dcp, units in meters, also Dep must be in meters).
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Spot on! Thank you.


