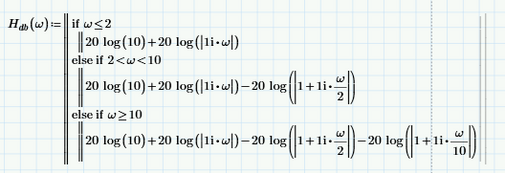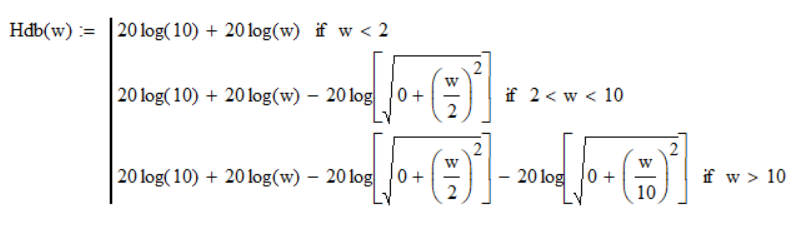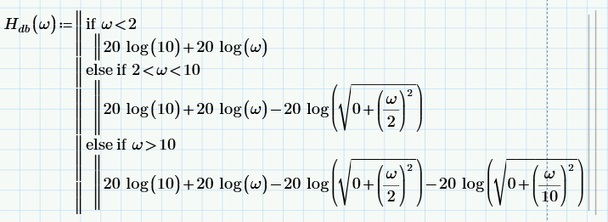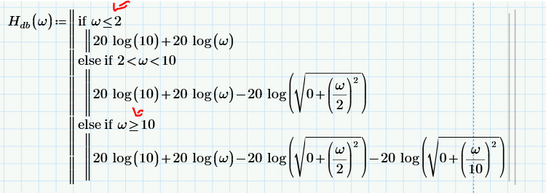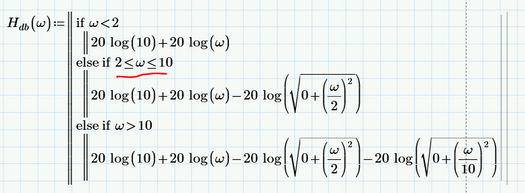Community Tip - Visit the PTCooler (the community lounge) to get to know your fellow community members and check out some of Dale's Friday Humor posts! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Asymptotic Bode Plot Issue in Mathcad Prime 7
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Asymptotic Bode Plot Issue in Mathcad Prime 7
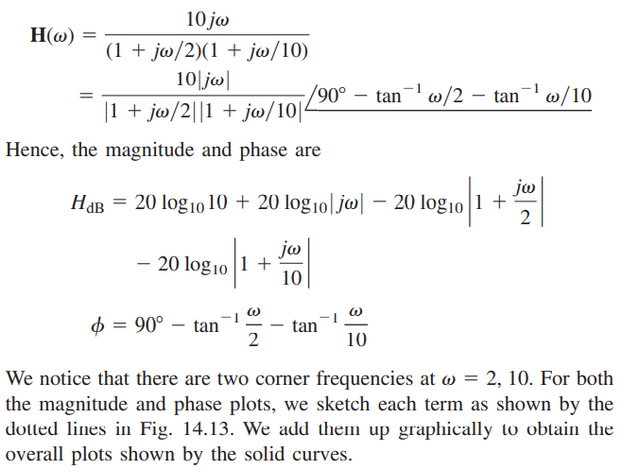
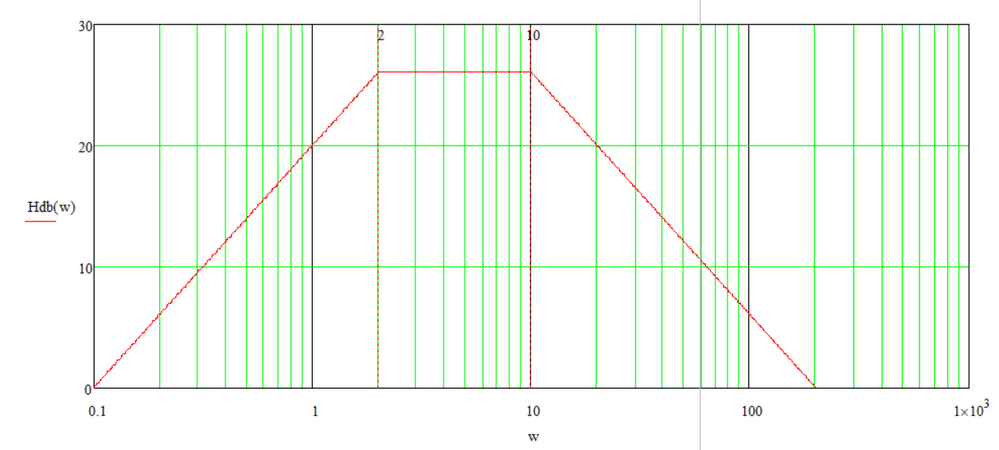
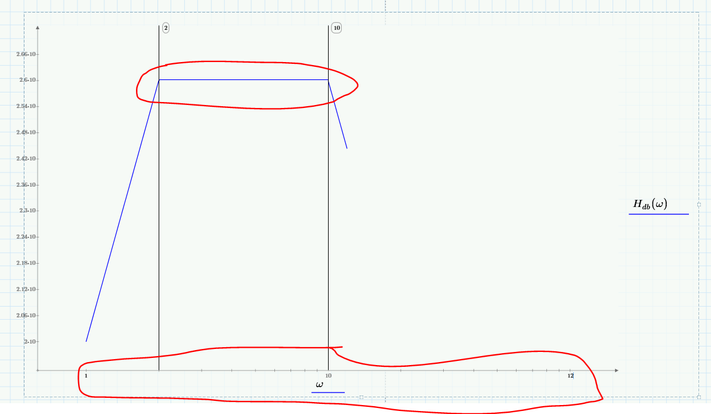
Obtaining the ASYMPTOTIC Bode plot for the following transfer function:
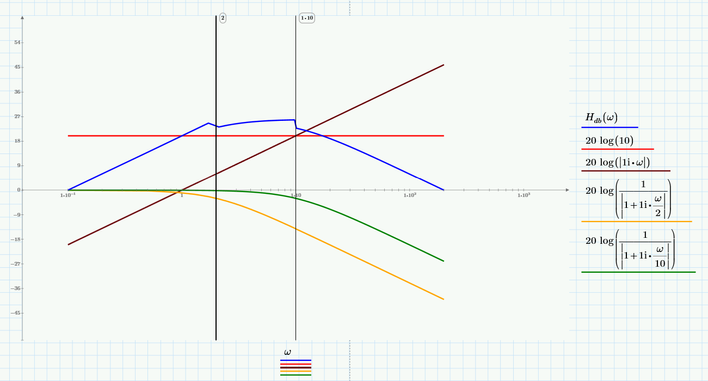
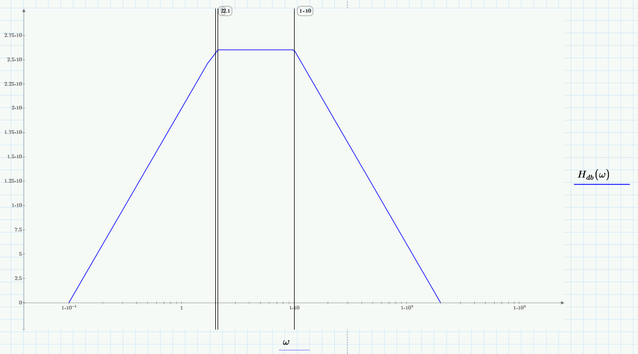
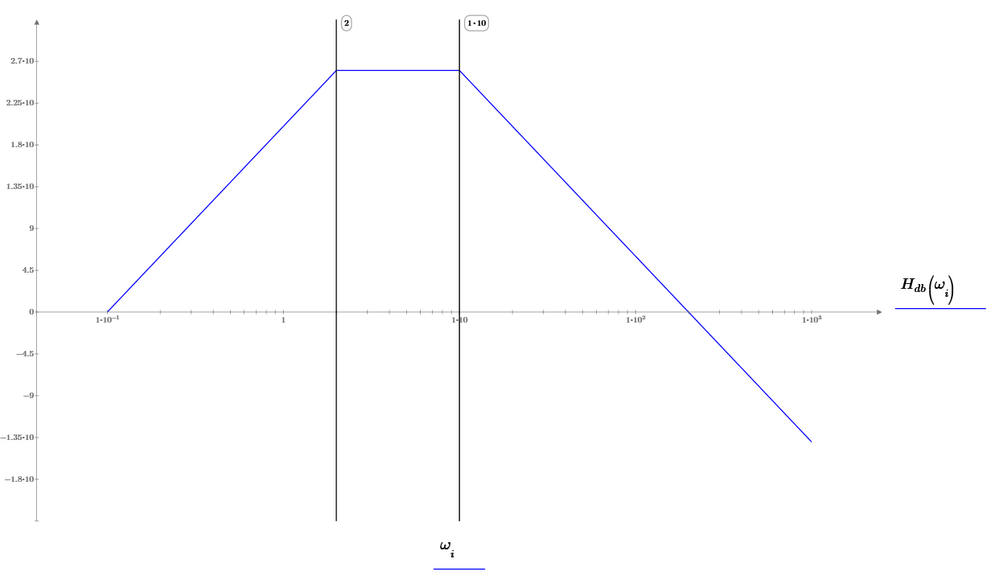
Mathcad Prime 7 result:
Why does the result in the Mathcad Prime 7 not look like it looks like in the figure in the example??
The idea is that I want the plot in Mathcad Prime 7 to look exactly like in the picture above, with straight lines and those corners, and not to bend corners Mathcad Prime 7 at w=2 and w=10.
Mathcad Prime 7 file attached.
Solved! Go to Solution.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
To get the asymptotic approximations you simply have to replace in expressions like 1+j w the 1 for a zero (aka omit it).
For w -> infinity these 1's are neglectable.
Maybe this link additionally helps to understand: https://lpsa.swarthmore.edu/Bode/BodeHow.html
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
To get the asymptotic approximations you simply have to replace in expressions like 1+j w the 1 for a zero (aka omit it).
For w -> infinity these 1's are neglectable.
Maybe this link additionally helps to understand: https://lpsa.swarthmore.edu/Bode/BodeHow.html
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Mathcad 15 result:
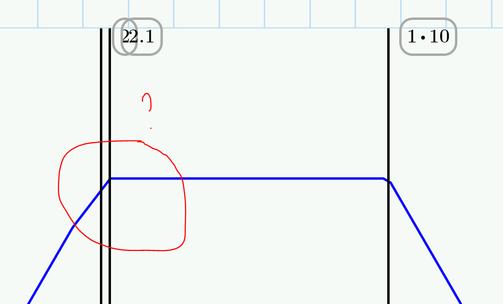
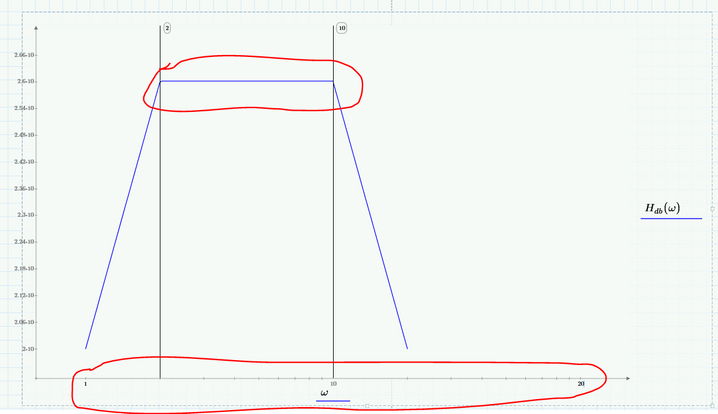
Mathcad Prime 7 result:
At w = 10, let's say it's something more ok (even if it's not even right angle there at the intersection (as it kind looks like in Mathcad 15)), but at w = 2 why is not ok (only somewhere at w=~ 2.1 seems to be more ok/accurate)?
The same results, no matter where I put the greater than or equal sign (so that it also contains the end values w=2 and w=10):
Mathcad Prime 7 file attached.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
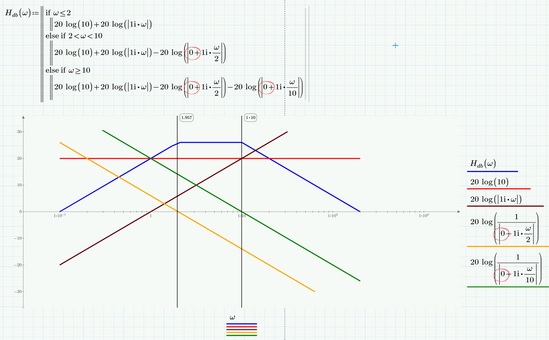
@Werner_E I think I found the answer. At least that's how it seems to me so far, but I'll post if I find anything else...
Thank you.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
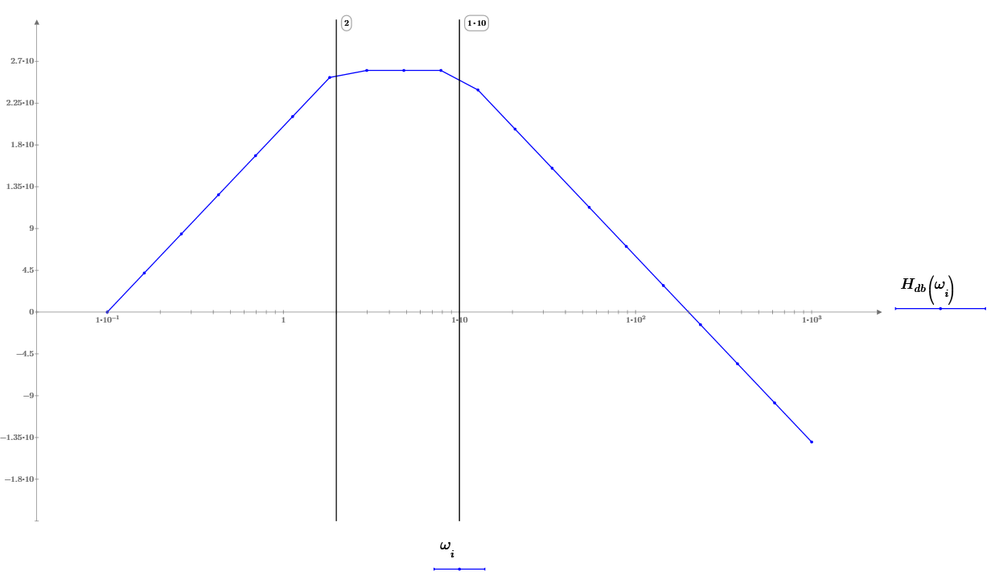
Yes, Mathcad (15-) is much better when it comes to autodetect suitable scales for the axes in a quickplot. Prime almost always requires you to manually adjust the scale to get useful values.
This seems to be what you did and it looks like it worked OK.
Normally you have a better control if you don't use a quickplot but rather define a range variable for the abscissa values (in Prime you often will still have to adjust the second axis value).
Unfortunately ranges are linear only and you would have to define a very dense range (resulting in quite many points in the plot) if you use a log scale.
Way out can be to define a vector with "logspace" as ttokoro had shown and then plot one vector over the other.