Catenary and cone - one fresh problem for Mathcad community

[ValeryOchkov]

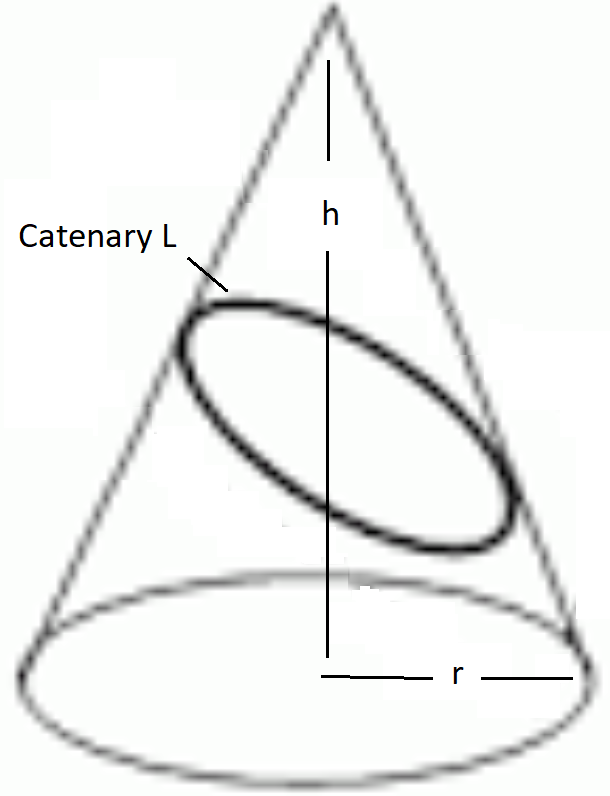
Do you know how a catenary lies on the cone? Without friction!

[-MFra-]

Hi Valery,

You should be clearer because the figure shows an ellipsis. It is known to all that the intersection of a plane with a cone is a conic (in this case ellipsis precisely). Therefore I deduce that the catenary is generated by the intersection of a cone and a non-flat surface. the system between the equation of a cone and an unknown surface must give a catenary. Does this surface exist? ......but then, since the catenary is a flat curve, the surface should be a plane, which contradicts the previous deduction.

The cone is ready...........

[ValeryOchkov]

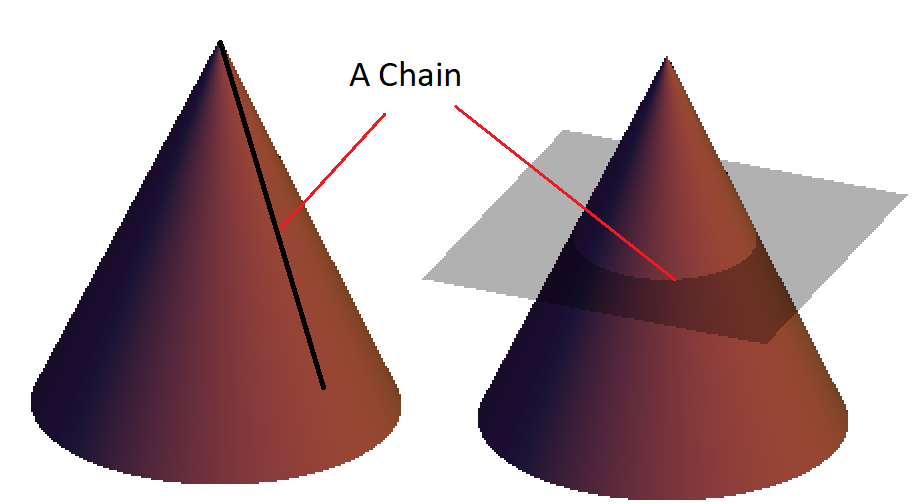
1. Sorry, it is not a catenary (one plane curve), but a chain.

2. Better use a cylindrical system of coordinates

3. This problem has two boundary cases:

- a circle

- a part line 

[-MFra-]

So the problem seems trivial because the circle is given by the intersection of the cone and a plane perpendicular to the axis of the cone, while the chain is the locus of points where the plane is tangent to the cone .....

[Fred_Kohlhepp]

The basic catenary (chain suspended from each end) is constrained by requiring the horizontal component of tension be the same for each point along the curve.

This problem requires two additional constraints:

1. the chain must lie on the surface of the cone, and
2. the length of the chain, and the end points must be specified.

A general solution is going to be complicated.

[-MFra-]

Hi FredKohlhepp,

I fear that the chain suspended between the two ends is simply your "invention".

[Fred_Kohlhepp]

---

@-MFra- wrote:

Hi FredKohlhepp,

I fear that the chain suspended between the two ends is simply your "invention".

---

What is your definition of "catenary"?

[ValeryOchkov]

---

@Fred_Kohlhepp wrote:

---

@-MFra- wrote:

Hi FredKohlhepp,

I fear that the chain suspended between the two ends is simply your "invention".

---

What is your definition of "catenary"?

Sorry - not a catenary but a chain, a round chain.

---

[Fred_Kohlhepp]

---

@ValeryOchkov wrote:

---

Sorry - not a catenary but a chain, a round chain.

---

---

Okay, but you put catenary in the title; and the statement that it's a chain implies a condition about tension in the chain.

I'm not trying to invent anything, just make an observation about the problem.  🙂

[ValeryOchkov]

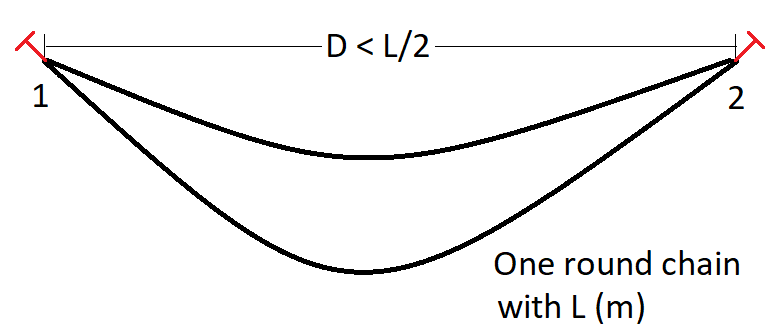
One auxiliary problem!

A round ideal chain is fixed without friction in/on/with two points.

We have one Unstable equilibria and two symmetric stable equilibria - see a picture.

Let solve this problem at first with Mathcad!

[-MFra-]

The chain is not elastic, it is inextensible like a rope. Quite right? In points 1 and 2 the chain flows freely and without friction, right? you desire the study of the motion of the system, assuming that there is no friction, and that on it acts the weight force only.  The motion starts from any position. Quite right? Or should we also consider the rotary motion of the chain?

[ValeryOchkov]

---

@-MFra- wrote:

The chain is not elastic, it is inextensible like a rope. Quite right?

Yes!

In points 1 and 2 the chain flows freely and without friction, right?

Yes

you desire the study of the motion of the system, assuming that there is no friction, and that on it acts the weight force only.  The motion starts from any position. Quite right?

No!

Let start not with a dynamic but with one static problem!

 It will be a good problem for students - to create an animation with D := L/2/999*FRAME - see please the picture above. At D=0 we have a vertical straight line segment. And at D->L/2 we have a horisontal straight line segment. Between those two boundary points we haw two catenary - a big and a small. This problem can be solved thru two ways - by Newton (forces) or by Lagrange (potential energy). 

---

[ValeryOchkov]

I am solving (try to solve with Mathcad) the problem from the article: