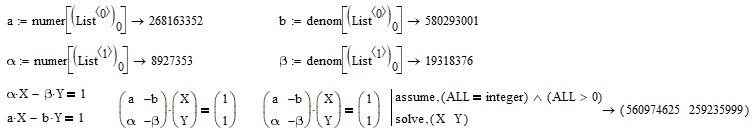Community Tip - You can Bookmark boards, posts or articles that you'd like to access again easily! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
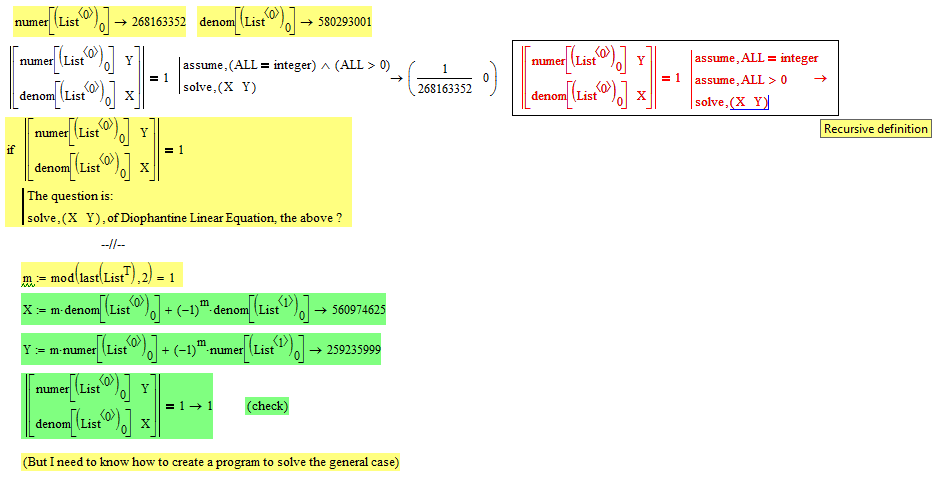
Diophantine Linear Equation ?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Diophantine Linear Equation ?
Hello Everyone.
--//--
--//--
--//--
Thanks in advance for your time and help.
Regards.
- Labels:
-
Math Homework
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
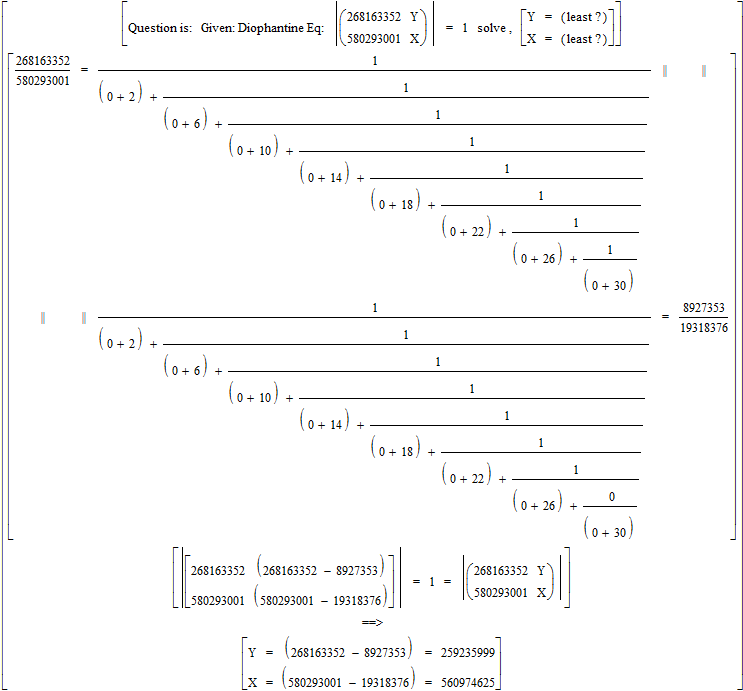
The determinant gives an equation with two unknowns. However, if I add another one relating to the next ratio, I obtain a non-homogeneous linear system of equations in the unknowns X and Y, of which there is a solution. What do you think?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks for your time, MFra. I guess we should consider something about: 
Thanks your time again.
Best Regards.
Loi.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
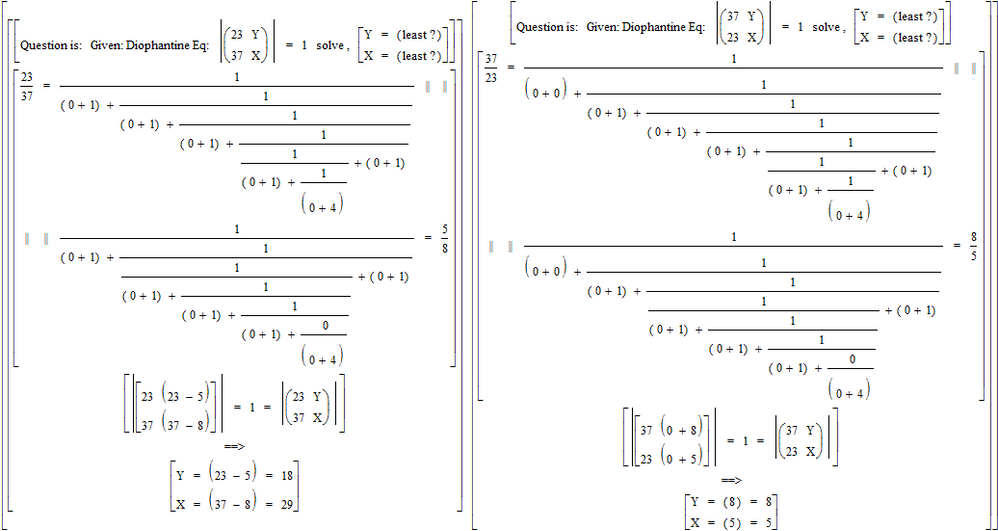
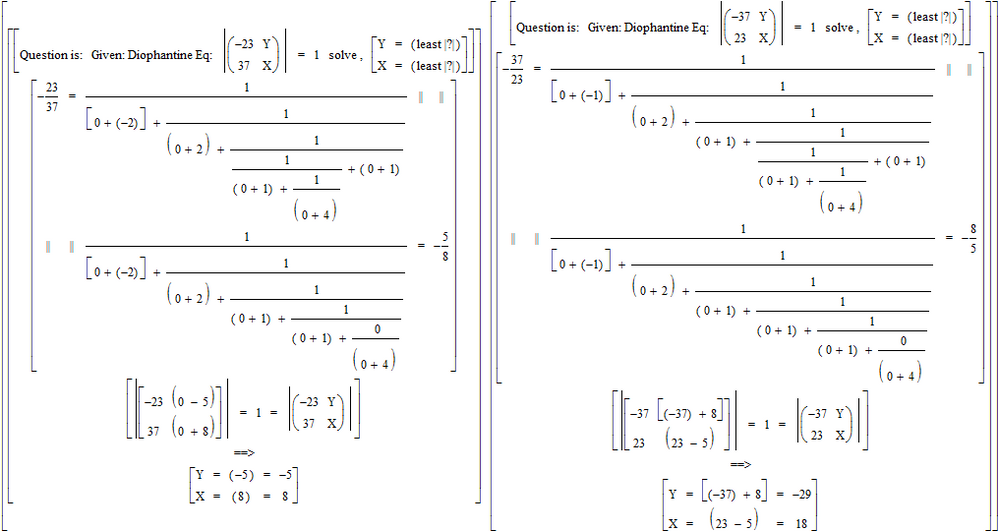
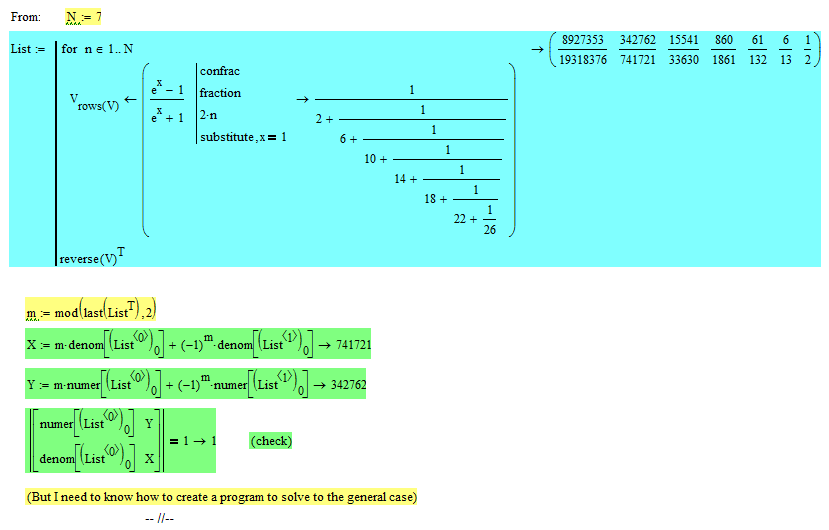
I know it's not the answer you are looking for, furthermore, some are negative and aren't diophantine solutions, but it's already something ....
Instead of defining a linear non-homogeneous system of equations with constant coefficients in two variables, in the same way, one should define such a system in N equations in N diophantine unknowns. I don't know if that's possible.
In the group "Gruppo di Matematica" of FB, there is a professor (Domenico Annunziata) who has written a book dedicated to Diophantine equations, you could turn to him for clarification.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
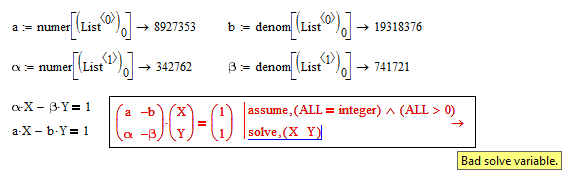
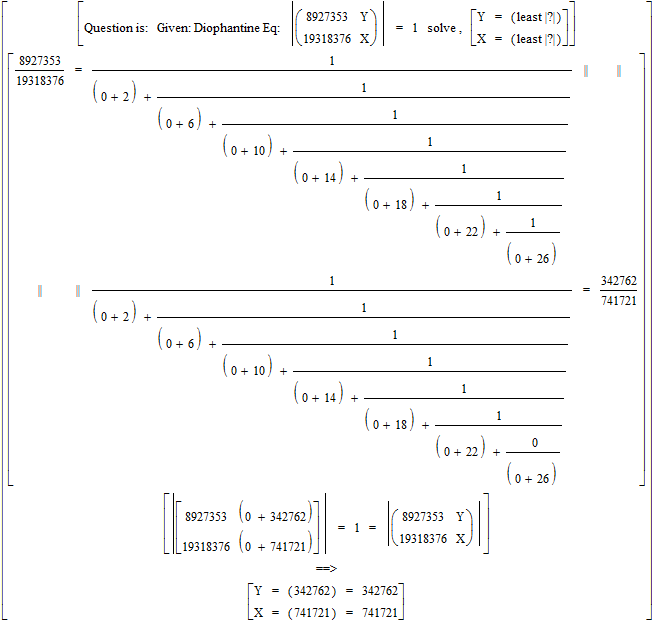
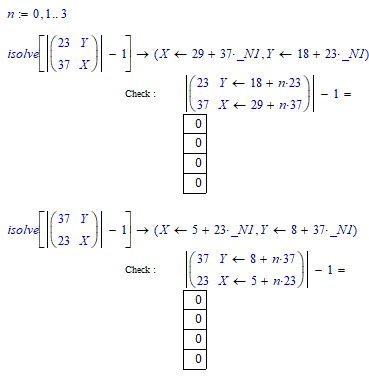
I think this shows that Maple will happily solve your equations:
Success!
Luc