Community Tip - Learn all about the Community Ranking System, a fun gamification element of the PTC Community. X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
HELP WITH GIVEN and FIND
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
HELP WITH GIVEN and FIND
I am trying to solve a set of equations using Given and Find. I wish to define some boundary conditions. Eg. I may wish to limit some answers to a range between 0 and 1 or limit some values to positive numbers numbers. I don't know how. Your help will be appreciated. File is attached.
Basically I am using current and voltage data from relays at both ends of a line to find a fault location.
If we limit all values to real and M is between 0 and 1
The expected answer for U is about 0.75 - 0.76.
Solved! Go to Solution.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
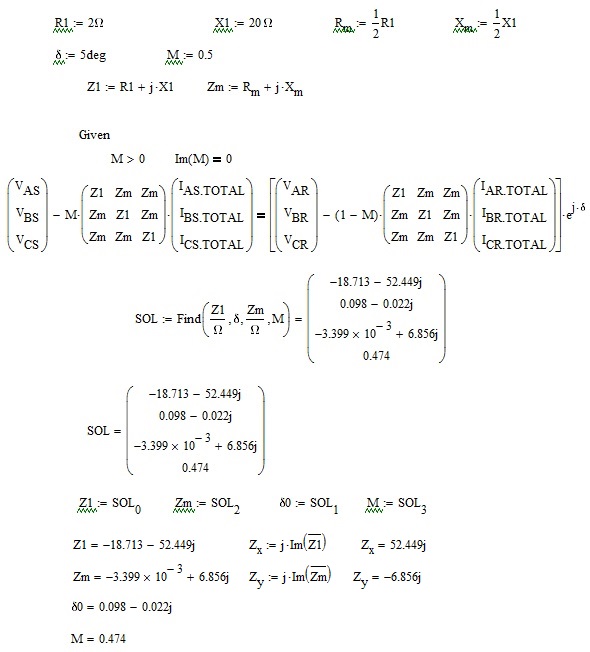
I think you have not addressed the problem properly. You should consider the impedances of which the resistance is the real part and the reactance the imaginary part. The results must adhere to these definitions.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Toby Metcalf Can you please move this question to the Mathcad section.
The PTC Community Help section of the forum is for question about the forum itself. Toby will move the post to the right place and you'll get the help you need.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Why do you name the variable M when it should be U?
You can name it U in the solve block and in all your equations and can albeit name it U in the solve vector, too.
As for the conditions, you may simply write 0<M<1 somewhere in the solve block. You get U=0.534
If the guess values are real, Mathcads solver will stay with real numbers.
If you want non-reals, then you would have to provide a non-real guess value.
But it seems that as your formulas use the complex unit j, you get a complex value for M rsp. U
To force a variable R1 to be real you could add something like Im(R1)=0.
As you have just 3 equations but 6 variables to solve for, you have a great degree of freedom concerning the possible solutions.
Werner
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I think you have not addressed the problem properly. You should consider the impedances of which the resistance is the real part and the reactance the imaginary part. The results must adhere to these definitions.

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
No need to demand Im(M)=0 as M>0 implies M is real.
But of course it does not harm.





