Community Tip - You can Bookmark boards, posts or articles that you'd like to access again easily! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Help With An Instrumentation Amplifier
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Help With An Instrumentation Amplifier
I'm trying to make an instrumentation amplifier with a 10v/v gain. My friend asked me for help, and I'm a little embarrassed to say that I couldn't help her out, but her question piqued my curiosity.
From what I knew, gain was decided by the following equation:
(Vout)/(V2-V1)=(1+(2*R1/Rgain))(R3/R2)
But every time she tested it, she got some strange result. Am I wrong about 10v/v meaning that Vout=10(V2-V1)? How can I make this work? Any help would be appreciated.
Here's a link to what we're working with.
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
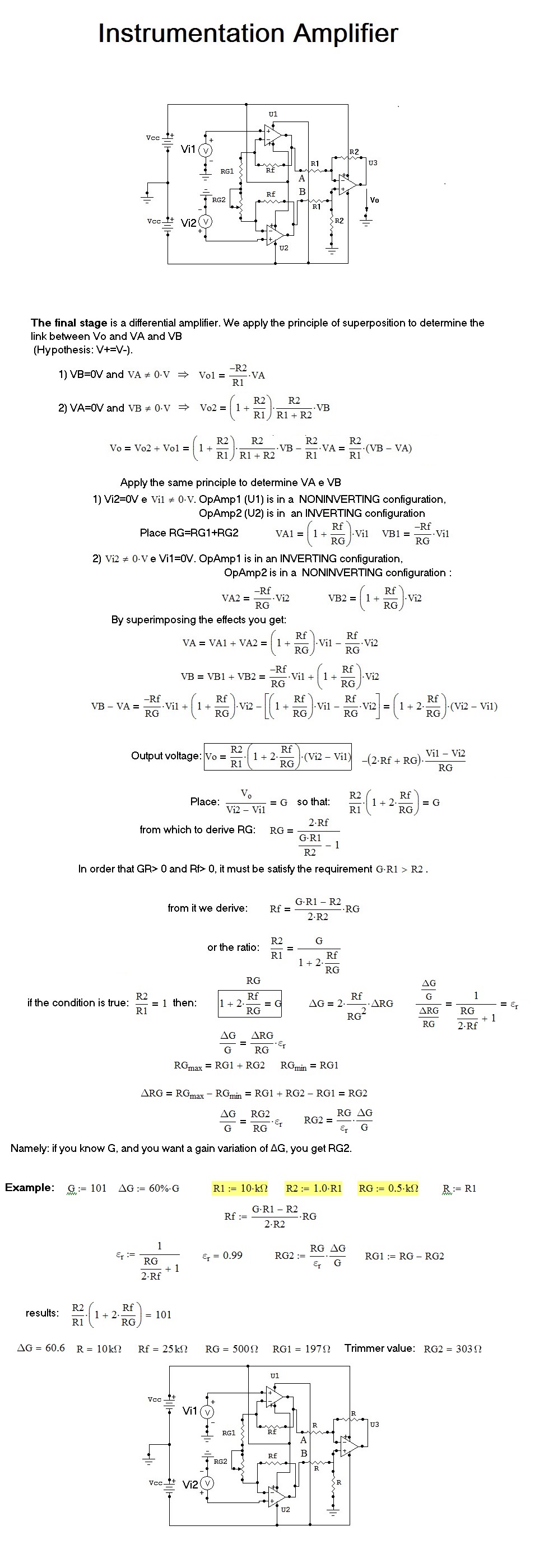
If you analyse it more thoroughly:
This should explain why the Instrumentation amplifier wasn't reacting as expected.

This gives a fixed gain, independent on input voltage.
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You have a very non-symmetrical instrumentation amplifier: why is R2 value different from R1's?
You are putting a lot of input on the V2 input. What is your power supply range, and does it accomodate 19 V input?
Have a look at the internals of an industry instrumentation amplifier, e.g. INA 121: http://www.ti.com/lit/ds/symlink/ina121.pdf.
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello LucMeekes!
What do you think about my solution?
Bye
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I think your analysis is OK, but doesn't match the schematic Here's the original poster (OP) is working on.
The two feedback resistors in there (R1 and R2) are completely different in value. whereas you have them (Rf and Rf) nicely exactly the same.
Plus the OP is putting an awefull lot of voltage into one input, and I cannot see the supply voltage. In your analysis you're starting off from idealized OpAmps, (infinite supply, infinite open loop gain) which is OK if these factors don't play a significant role. The simulation on the other hand must take account the supply voltage and (I hope) uses realistic models of the OpAmps used. I don't think it's strange that the simulation gives results that are unexpected ("But every time she tested it, she got some strange result.") if you use the setup as depicted with the gain formula { (1+2*R1/Rg)*R5/R3 } that applies to a nicely balanced instrumentation amplifier. The picture shows that that would result in a gain of 11.6... In fact if I fill in his data, I get a gain of:

But the InAmp doesn't have 2x10k for R1 and R2. It has 10k and 470, and with those values I might try:

This should explain part of the "strange result". The other part may have to do with the large input on V2, which would probably clamp one input of OpAmp U2.
Regards,
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If you analyse it more thoroughly:

This should explain why the Instrumentation amplifier wasn't reacting as expected.

This gives a fixed gain, independent on input voltage.
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you for your thorough answer.
I have approached the problem from the practical point of view, thinking that Shennae was experiencing the circuit in the lab.
The circuit of Shennae, I didn't watch endeed, thinking that Shennae was referring to one of the most common schemes of the instrumentation amplifier, also, I have considered the Opamp ideal.
Bye
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You're welcome.
Note that I too have considered the opamp close to ideal. But taking the open loop gain in is the minimum to solve such systems.
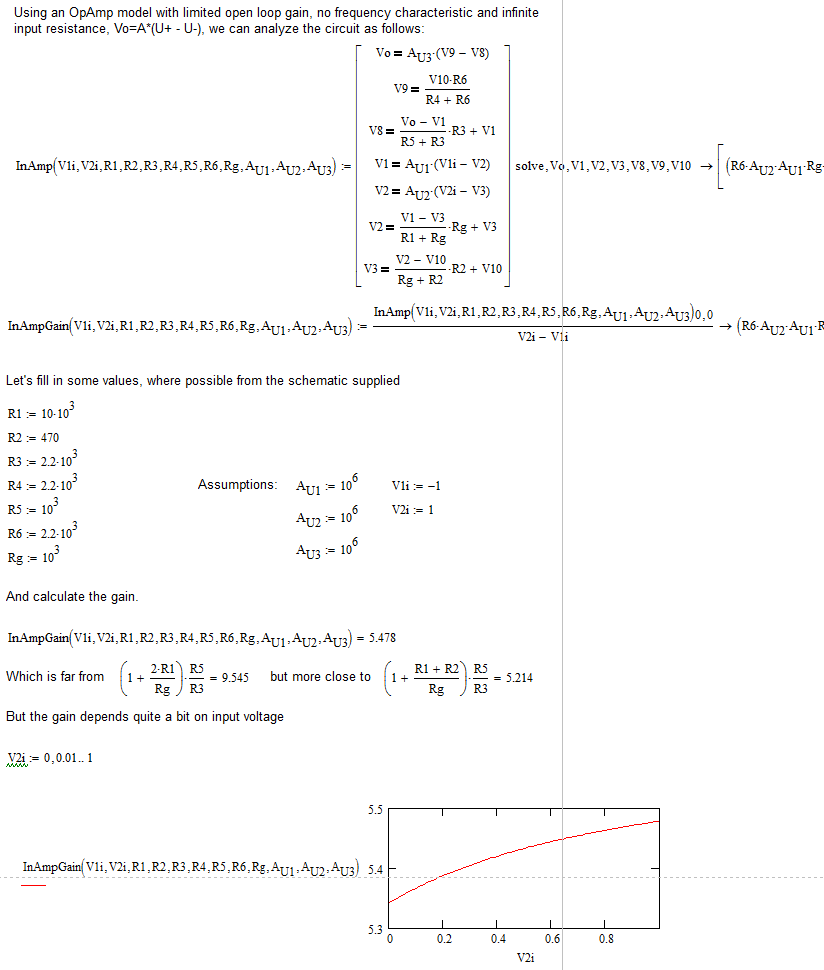
You can take the analysis a little further:

Which, in the end, if R1=R2, results in the famous G= (1+ 2* R1/ Rg)* R5/ R3.
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi! I'm in the Mathcad community for some years,
Very nice model of opamp. I suppose it's only used for teaching, because in practice the opamps have, I would say all (or the most common), open loop differential voltage gain of the order of 50/100000; so my approximation is perfect. One might as well remake the model taking into account the high open loop differential voltage gain. I think it would certainly be more useful, without passing for every op amp, in subsequent calculations, to the limit of the closed loop voltage gain for the open loop gain tending to infinity.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
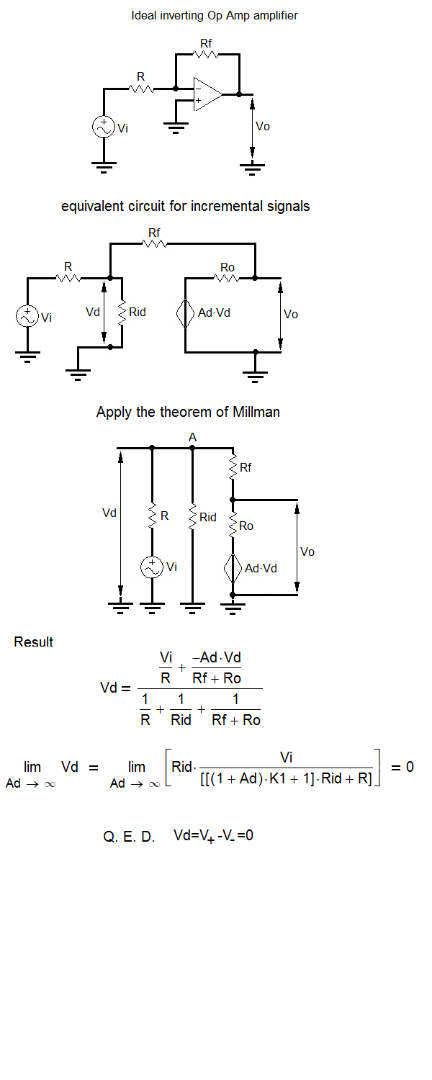
Essentially the assumption that the voltage difference on the two inputs of an OpAmp is (exactly) 0 is equal to the assumtpion that the open loop gain is infinite.
So, contrary to my statement above that the open loop gain is needed, there is another way to solve such networks mathematically, and that is using the zero input voltage difference, you've used this assumption implicitly in your analysis.
With the Open loop gain in, as shown, the system is solvable. But the resulting equations tend to be complicated and difficult to grasp. You can see that from the InAmpGain() function before I take the limits. But if you're willing to make the effort, you can restructure the equations such that simple gain formula comes out with the factors that disturb it as a result of limited open loop gain. But now we're focussing on just one non-ideality of the OpAmp....
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Attached is a schematic and calculation for a two stage amplifier with a gain of 400 and a 100 Hz low-pass filter.
Built, tested, works. Adjust as needed.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I wish I could list you all as correct answers, thank you so much for your help!