Community Tip - New to the community? Learn how to post a question and get help from PTC and industry experts! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
How to finde the volumen between 2 Cylinders?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
How to finde the volumen between 2 Cylinders?
Hallo,
I would like to ask for your experience. I need to find the volume with triple integrals between 2 cylinders. They are not along the same axis.
To imagine how they are found, they form a cross, like this: +
The first one is an ordinary cylinder and it follows the form z= 3 sin (phi). This was really easy.
The second one is an elliptic cylinder. Here are starting the problems. The area of this second cylinder is given by x^2/a^2+y^2/b^2. For a = 2.6 and b= 3.0
But I just can transform this second one into cylindric coordinates and at the same time turn it 90° from the first cylinder to form this "+" cross.
Do you have any idea?
Thanks a lot!
Best regards.
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The trick to the integration is to set the limits correctly.
I wouldn't bet lunch that this was right.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Here is a basic MAthCAD document... it is missing the main part =S
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Is this what you mean about two cylinders?
If so, then we can move forward to develop the integral
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Fred
You´ve got it! Wow, yes it is like that. How would you start the integral?
Due to a some reason, I can not see the graphs...
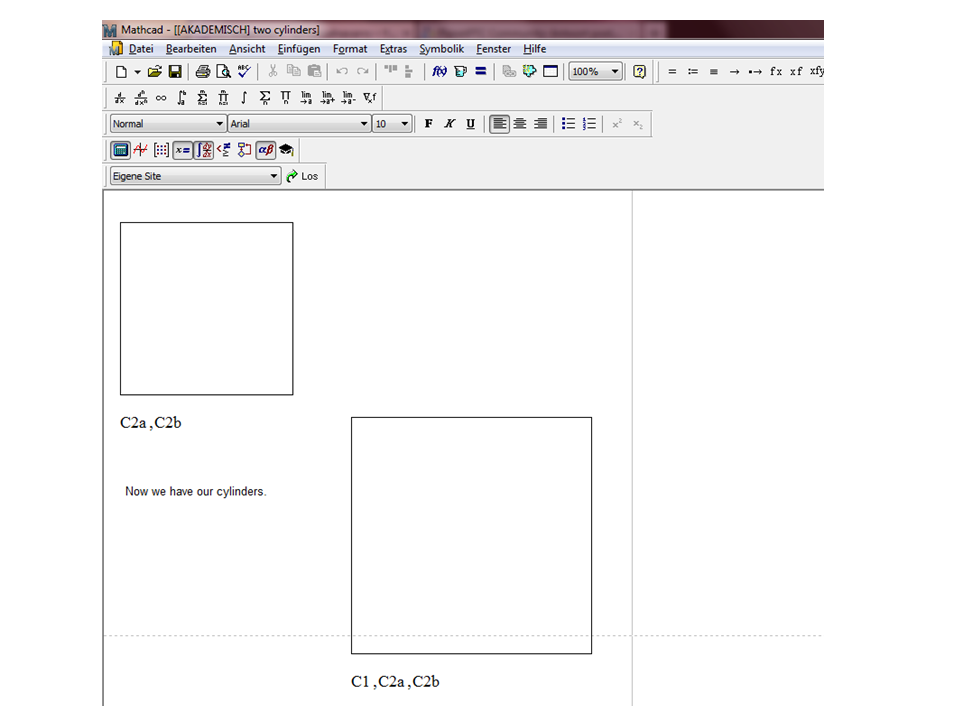
Thanks y lot!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Right click on the graph and uncheck "border". Depending on your OS, that might fix it.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Richard,
it fixed it!
Thanks!
It is getting more and more exiting. Now, how can be the volume found? It looks like you have the answer is almost there.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Fred,
I have solved the problem in another way. But I am very interested in the way you are solving the problem. I would like to ask you if you could continue with it? It was really sophisticated and I would really like to see your answer.
Here is my version as well.
Thanks!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The trick to the integration is to set the limits correctly.
I wouldn't bet lunch that this was right.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I wouldn't bet lunch that this was right.
I wouldn't bet my lunch on anything ![]()
Mike
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks Fred,
I wouldn't bet my lunch as well... Sorry for not answering before. My Hard disk just died.
I think it is quite well, numerical approaches give a little smaller value, but it is ok. I need to learn more about MathCAD to fully understand that what you did.
Regards and thank all of you for helping.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello!
Hope this helps: https://www.math.drexel.edu/~jwd25/Calc4_Winter_09/lectures/Lecture8B.pdf
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks Vladimir,
it was quite helpful, however, the definition of the function is still not perfect...





