- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
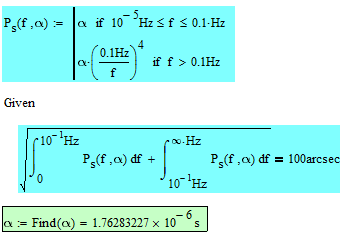
I'm having difficulty with this solve block problem
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I'm having difficulty with this solve block problem
Any suggestions on how to approach this problem would be appreciated. Thanks.
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
As always, thanks for your prompt and detailed help. The solution you provided makes it obvious what I overlooked ... I needed that! Also, thanks for the "otherwise" usage help, it made it much more straightforward. Oh yes, thanks for leaving the clue to the definition case that I overlooked and left out as well.
Jay
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You are welcome.
Looking at it again I still don't understand why the integral from 0 to infinity (we had that in another thread) does not work.
And in this example I don't understand why the first integral (from 0 to 0.1) works, because the integrand is not defined for the range from 0 to 10^-5). Mathcad - always good for a surprise 😉
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
Yes, interesting.
There appears to be something there ... ?
However, this says otherwise ... ?
Perhaps there's some sort of round off residuals ... ?
It's over my head,
Jay
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I am not sure what your second expression will show. You are aware of the fact that sqrt(a+b) is NOT equal to sqrt(a)+sqrt(b)!?
First we would had to agree upon what value(s) Ps should yield for 0<f<10^-5 and define the function accordingly.
I set the lower limit to 10^-5 instead.
Then some kind of scaling could do good - so it seems you are right about the round offs:
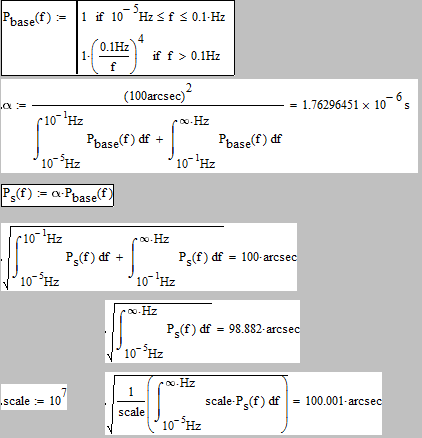
You see I catched on Alan's suggestion and omitted the solve block.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
Yes, thanks for keeping me on track! I apprciate your (and Alan's) time. Your scaling idea was very good, I wish I had thought of it. Your (and Alan's) insights have been very helpful. Thanks again.
Jay
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You might also notice that, in this case, it is a trivial matter to do the integrations analytically:
Alan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Alan,
Thanks for your input. Please share with me how you arrived at the 1/30 part ... it's not that obvious to me.
Jay
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
As follows:
Alan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Alan,
Thanks for the explanaton ... I thought that you maybe had something in mind that didn't involve an integral. What you show is very clear. Thanks for your insight, this was helpful for my thought process.
Jay