Community Tip - You can Bookmark boards, posts or articles that you'd like to access again easily! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
I need help understanding these results. ALl comments & suggestions welcomed. Thanks
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I need help understanding these results. ALl comments & suggestions welcomed. Thanks
I'm sure there's something fundamental that I'm overlooking.
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
When in doubt, ask the symbolics for the exact results. The symbolics does not know anything about units and would treat them as variables, so I omitted them.

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Obviously a conflict between the way the function is defined and the numerical algorithm used. You get very different results whether you chose Romberg or Adaptive method (rightclick menu).
You get the result you expect if you split the integral at 0.1 Hz yourself.
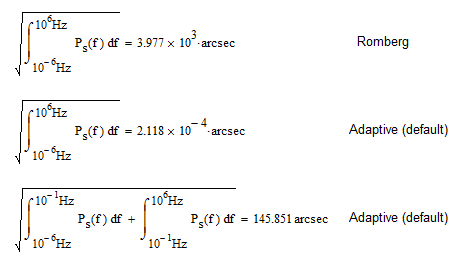
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
When in doubt, ask the symbolics for the exact results. The symbolics does not know anything about units and would treat them as variables, so I omitted them.

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
Thank-you very much for your time and help. Your suggestions and reminders about the numerical integration process (both sheets) were very helpful and appreciated. Thanks for the tutorial and pointing me in the right direction.
Jay
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You are welcome.
Nevertheless its still unclear to me why Mathcad's numerics fails that bad.
Mathcads symbolics wont operate on a function defined by if (neither programmed nor using the if-function).
So I rewrote it using the Heaviside function.
The numerics still fails
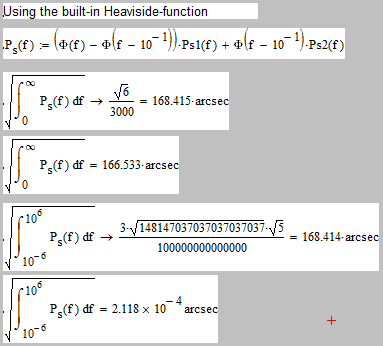
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
Thanks for your continued thoughts and efforts. I think the take-away here for myself is as you pointed out. With function discontinuities, always break them up at the discontinuities and sum seperately. The answers seem to be pretty good then. I too am also puzzled by the errors for such a simple function albeit with just one straighforward discontinuity. Otherwise, your suggested approach achieves spot-on results.
Thanks again,
Jay





