Community Tip - Did you know you can set a signature that will be added to all your posts? Set it here! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Integral calculus and Laplace transforms
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Integral calculus and Laplace transforms
Hi,
I am trying to solve the equation below to compute the dynamic pressure on a vertical sluice gate subject to rotational oscillation (q(t)) which is a sinusoidal function.
I am stumped evaluating the last integral that contains the cos (fo(s)) term for the phase function. It is not obvious to me how the integral is evaluated given the variable "s" is not the variable that is the subject of the integral.
When I type the equation below into Mathcad it gives an error saying the "s" variable is undefined.
Any clues to assist in solving this equaition would be appreciated.
Cheers
ross
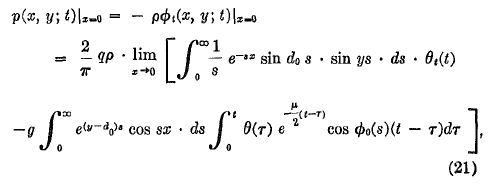
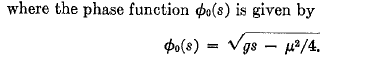
and g = acceleration due to gravity (g = 9.81 m/sec2) and m is a "small positive constant".
y = vertical displacement, x = lateral displacement, q,r,do are constants.
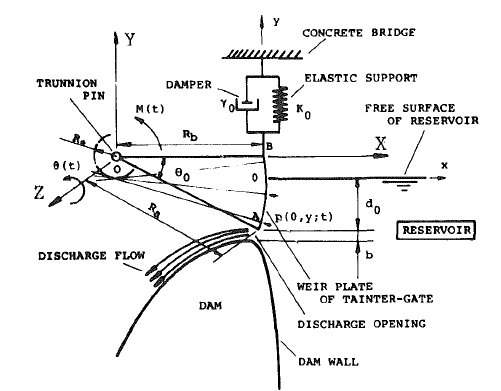
- Labels:
-
Calculus_Derivatives
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Your problem might be units. g is gravity, s has no defined units, mu is unitless. What would the radical evaluate to? If phi is phase, then it's radians (unitless.)
What units should s have to make the phase function work?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
As I interpret that function with all those missing constants (d0, tau) and functions (theta.t, theta) it depends on just the two parameters y and t and Mathcad is right, the s in Phi.o(s) in the last integral IS undefined.
So either you would have to check the formula or make s and additional parameter of the function.
What can you tell about Phi.s(x,y,t) - especially the meaning of the index s?
Proper units will be the next problem, I guess.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Fred and Werner,
Thanks for taking the time to review my problem.
Equation 21 I have posted is derived using Laplace transforms and Cosine transforms, hence the variable "s" comes from the Laplace operator. The first 2 integrals in equation 21 are integrated over "s" so there is no problem with these. The third integal is integrated over tau which is a time based function so the integral can be evaluated successfully if the cos(phio(s)) term is omitted. What I do not understand is how the phase function phio(s) can be evaluated within this integral with the Laplace variable "s" undefined. For the phase function phio(s) to be unitless (i.e. radians) this implies that "s" must be in units of the inverse of the gravitational constant g or units of sec2/m. This phase function appears in more than one tech paper by the author so it doesn't seem to be an error.
Cheers Ross
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So should it be a formula in time-domain or in s-domain? Looks like things got mixed up or there is a double meaning of s.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
From the phase fct definition, there is clearly a minimum threshold value for s, which is mu^2/(4g). Presumably, this has some physical meaning. If s is the Laplace variable, is it possible that the cos phi(s) term should be inside the s-integral, and that it may have slipped position when the double integral term was separated?
Lou
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Lou, Werner and friends,
One of my work collegues has I believe pointed me in the right direction. The final integral needs to be evaluated assuming the Phase Function fo(s) is a constant. The resulting expression which contains the "s" variable is then inserted in the middle integral which is integrated over "s".
The way I achieved this was to evaluate the final integral symbolically in MathCad (goodness knows how you write software to do this!!) which gave a nasty looking expression in "s". This was then substituited in the middle integral of Equation 21 and evaluated over "ds". The results look plausible in terms of expected magnitude and phase of the pressure p(x,y,t).
Thanks for your interest and assistance.
Ross
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So you treat the formula as if the ds is not before the last integral but at the end, after the dtau?
Hmm!?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
I have attached the mathcad file where I solve the third integral in equation 21 symbolically which gives a result containing the variable "s" and then insert the result into the second integral and integrate over "ds". Let me know what you think.
Cheers
ross
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
As I see it you have definitely changed the formula given in the way I suspected above. I can't judge if this would make any sense for your application.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
I guess the question is, is what I have done mathematically correct! I believe we should take it that the formula as derived is correct given the paper is by a chap who did a PhD in the topic, has published over 50+ papers in the topic and went on to become a professor (not that professors aren't exempt from errors!). On the assumption the forumla is correct how else would/could you evaluate the integral?
Cheers
Ross
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I don't doubt the competence of the author and I wouldn't be able to judge it anyway.
But its hard to say if anything done here ist mathematically correct.
What you evaluated is not equivalent to what the picture you posted shows.
What the picture shows cannot be evaluated, at least not if its interpreted the way most of us obviously do by common mathematical understanding. The notation and typeseting used is furthermore not helpful. The notation with the variable as index to denote a derivation I'm used just for partial derivatives of functions in more than one variable - wouldn't have thought that theta_index_t should have that meaning. We don't know anything about Phi_index_s(x,yt) or Phi_index_0(s). Also the arbitrary use and omit of multiplication dots is not helpful, either.
As you have access to the whole document, I hope the deduction of that formula may give you further hints.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
The theta_index_t notation does represent the time derivative - this is stated in the paper. I agree the omission of the multiplication "dots" is confusing.
If you have an interest I have attached the paper. Its frustrating, as without resolving this 3rd integral I am stumped solving the problem. Thanks for your time.
Cheers
Ross
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If you have an interest I have attached the paper.
Didn't made me any wiser on first sight. Especially the step from equ (20) to (21) is unclear to me. should be "just" the time derivative and a lim x-->0 and sign change.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
With some perseverence I have been able to solve the problem via another route. The same author pubilshed another paper that derivess the same problem equations using a slightly different method where he provides final equations without the mixed integral with Laplace variable "s" and time functions in the final integral.
The final equation is attached as pdf.
I have used the equations provided to benchmark some graphs in his paper and get the same results! The attached sheets show the solution and divergent unstable behaviour is predicted.
Many thanks for your assistance and interest in the problem. Have a good Xmas.
Regards,
Ross
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Perseverance pays off mostly 😉
Will look at the documents you sent later.
Merry Chistmas
Werner


