Hi
I am unable to calculate inverse of the matrix. The result is taking accuracy upto 3 decimal places. Even I tried to change decimal precision from "Math Formatting Option but the error become unchanged. "Please find given image and attached file for your reference.
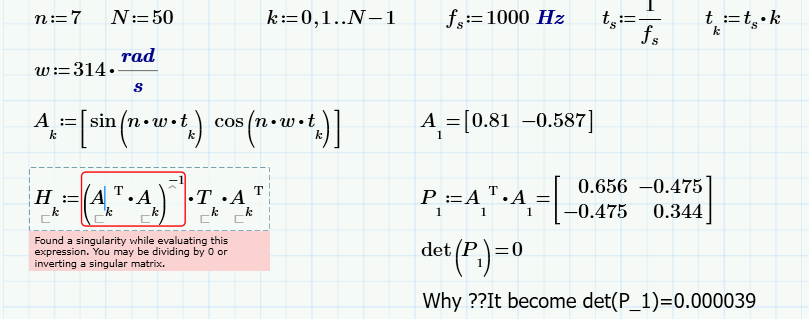for your reference:
I'm not sure what you mean by your final comment, or where 0.000039 comes from. The determinant of your matrix is zero. Always.
Edit. I figured out where you got 0.000039 from. That's what you get if you copy and paste the result matrix, with three decimal places, and then take the determinant. So it's non-zero because of serious round-off error. Changing the precision under math Formatting has no effect on the precision of the calculations, only on the precision of the displayed result, and will not generate that result.
As Richard had shown, any matrix which is built the way you build yours by multiplying any column vector with its transposed pendent gives you a matrix with a zero determinant and so ins not invertible.
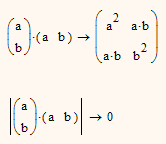
You calculated with the values you see
but Mathcad calculates with much more precision. So what you experience when you get 0.000039 is just an approximation of the real result, at best valid up to three decimals (you can't expect the result with a better precision than the input values) . And if you round your result to three decimals, you get .... Bingo!
Werner
Additional remark:
If you want Mathcad to calculate with inexact, rounded values, you would have to tell Mathcad so. Then you get the, for some reason significant, inexact and rounded value 0.000039.
Maybe this clarifies the situation (you have not shown the value of n but it looks like its 6.995):
The screenshot is of Mathcad 15, but it should be the same in Prime. You will have to change the display precision to more decimals to see the ....10^-17 value. If you leave it at the default (3 decimals), you will see 0. The same applies to the value 0.000039 at the end.
You already introduced an inaccuracy when you defined omega. Mathcad provides the unit "Hza" for this purpose or you may use the predefined constant pi to calculate with.
BTW, in which way would an inexact, rounded value be significant ?
Werner Exinger wrote:
BTW, in which way would an inexact, rounded value be significant ?
Because it's the answer in the hand- / sliderule- derived (to 3 digits) textbook/lecturer's notes answer? 
Stuart
> Because it's the answer in the hand- / sliderule- derived (to 3 digits) textbook/lecturer's notes answer?
Guess you are right. This would just explain why Sameer expected that result, but not why "the number 0.000039 has its own significance" 
Guess you are right. This would just explain why Sameer expected that result, but not why "the number 0.000039 has its own significance"
It is the duration, in ms, of the nuclear reaction when the largest nuclear bomb (the Tsar bomb) was exploded. 
Werner Exinger wrote:
Richard Jackson wrote:
Guess you are right. This would just explain why Sameer expected that result, but not why "the number 0.000039 has its own significance"
It is the duration, in ms, of the nuclear reaction when the largest nuclear bomb (the Tsar bomb) was exploded.
And I thought its because of
... or even
or 1 microstep ... (probably would only make sense to a Brit 
)
Stuart
