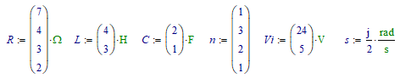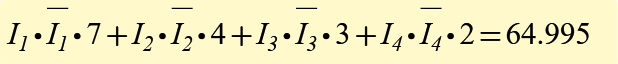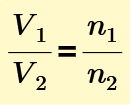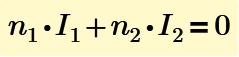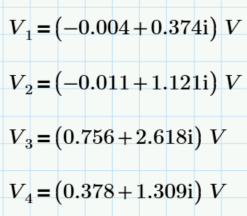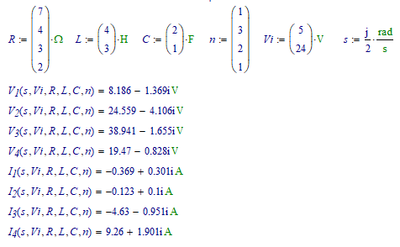- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Network analysis
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Network analysis
- Labels:
-
Electrical_Engineering
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ah, I used the wrong KCL around the transformers. After correction:
And with the correct values for Vi1 and 2:
The results now compare with those of ttokoro:
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So, we have 3 diffrent answers!
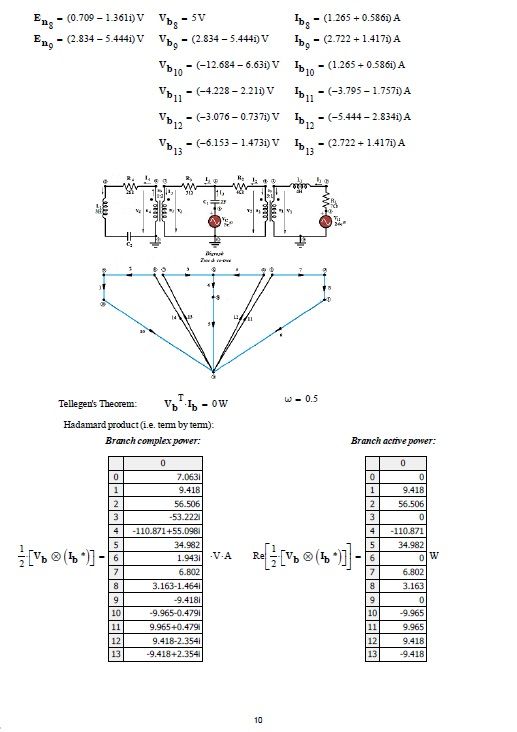
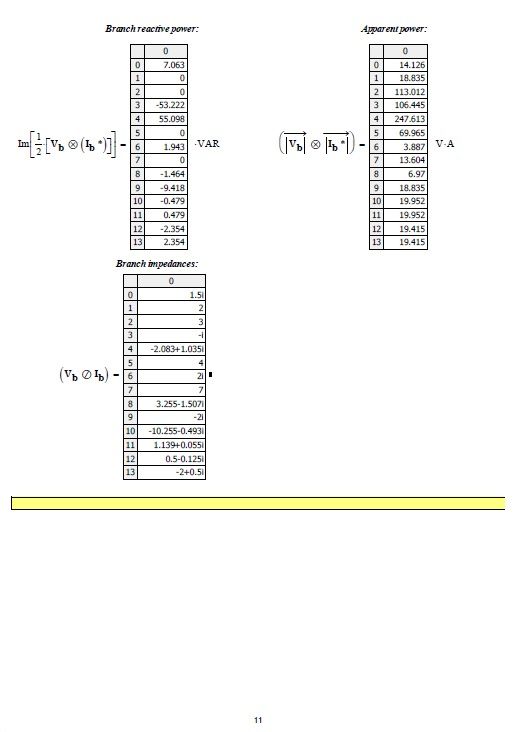
Total Power from Voltage sources.
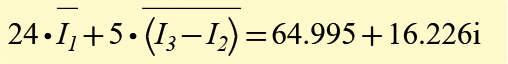
Total power loss by resistors.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
This is caused by your sign of the real part of I1 is negative.
Kirchhoff's voltage law, KVL, and Kirchhoff's current law, KCL, of transfomer are here. Therefore, assume all currents as counterclockwize, the sign is changed. This is may be the reason of your error.
PS. Your above equation should be 5V*(I3-I2)bar.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ah, I used the wrong KCL around the transformers. After correction:
And with the correct values for Vi1 and 2:
The results now compare with those of ttokoro:
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
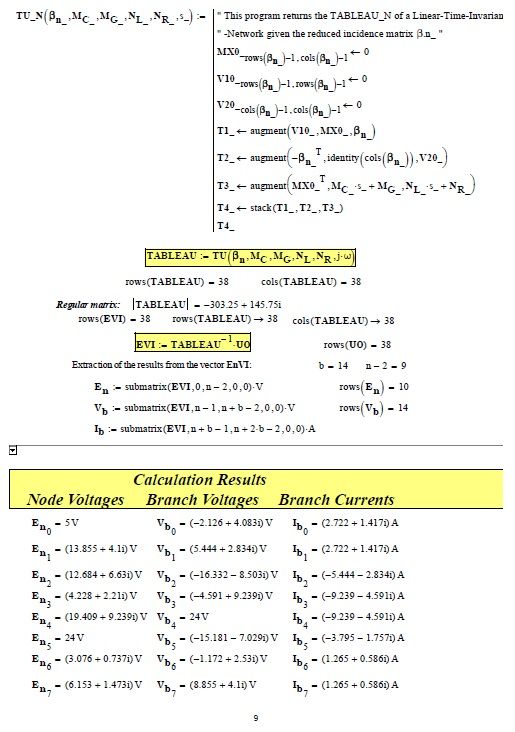
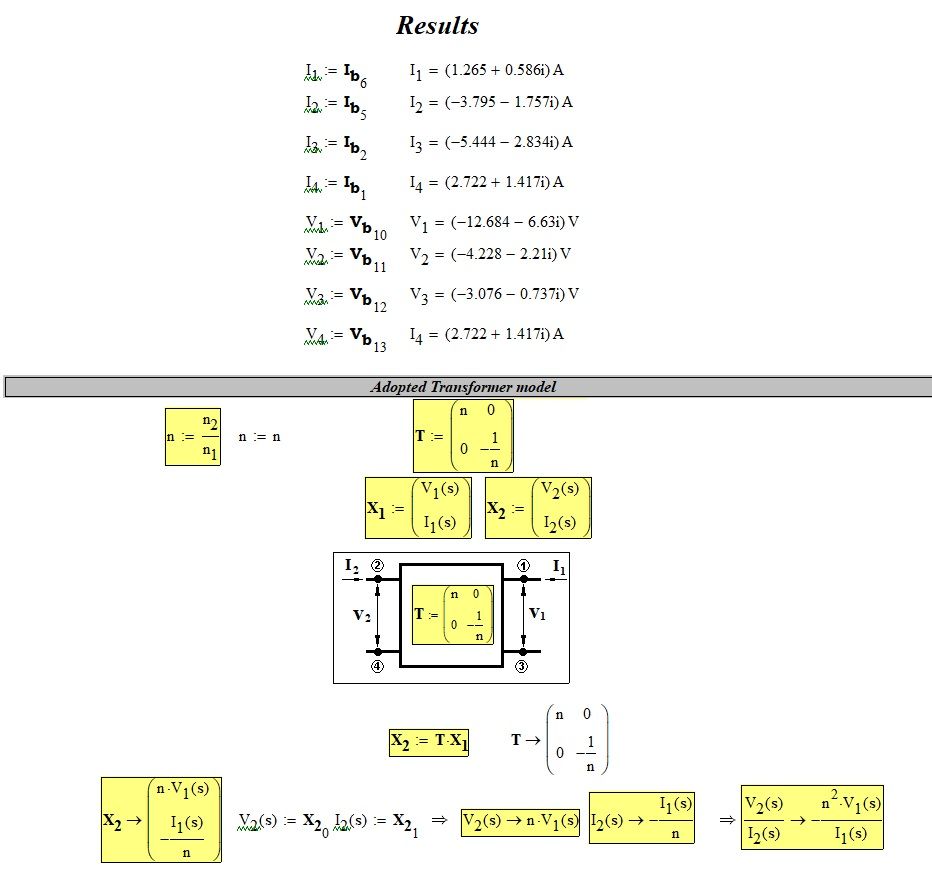
ttokoro, my result so far, but I still need to review again:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
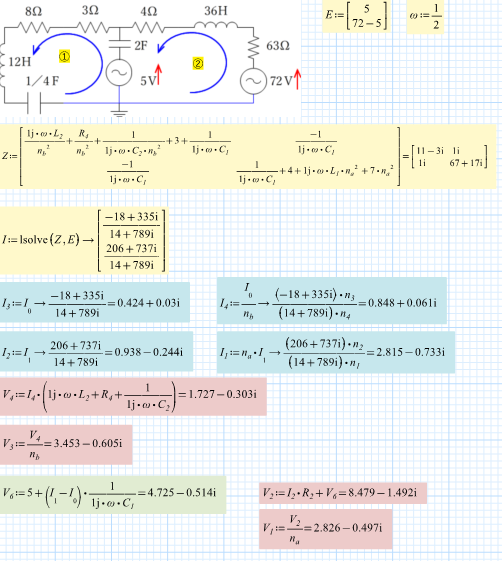
Now, we have 4th and 5th answers. My answer is solved under below conditions.
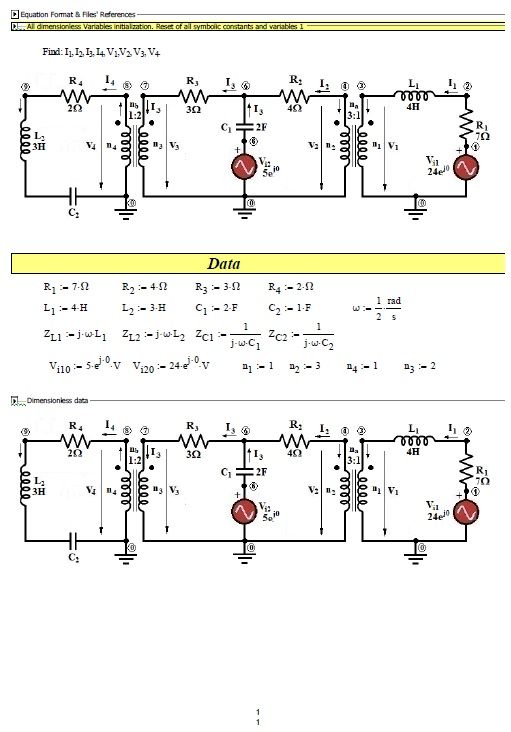
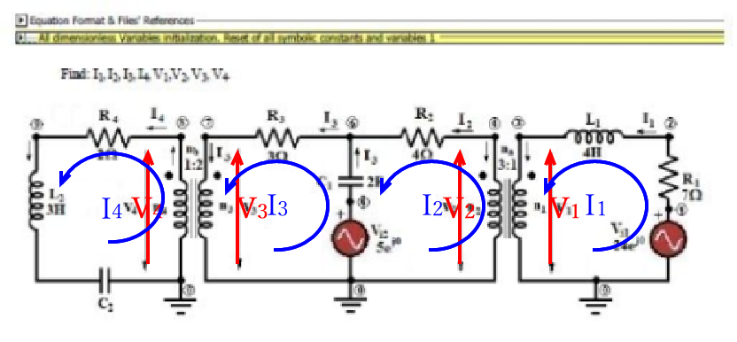
I1 to I4 and V1 to V4 are shown as below. We are asuming V1 to V4 as up arrow, instead of MFra's original one.
MFra's 5*e^j0 means sqrt(2)*5*sin(omwga*t+0) and so on for 24e^j0. e^j0 means the voltage phasor angle of t=0 is 0.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
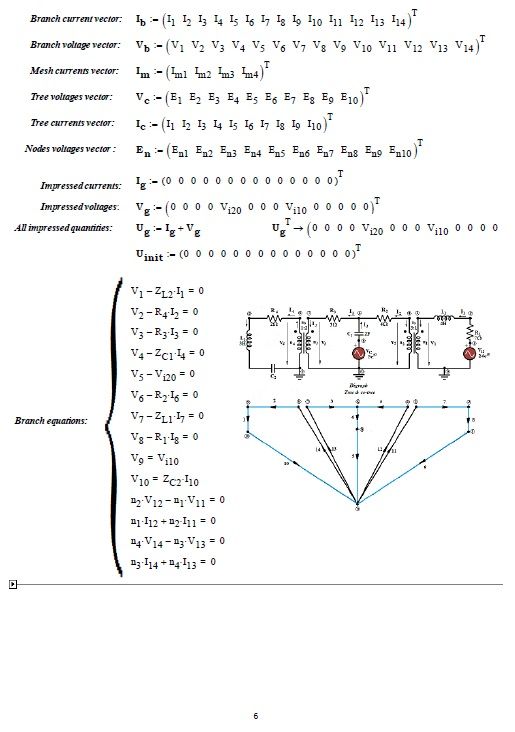
I usually indicate with arrows the potentials that go from + to -. Furthermore, regarding the transformers, the two black circles, one on the primary and the other on the secondary, placed at the edges of the transformers indicate the correspondence of the + phases.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
@ttokoro @-MFra- @LucMeekes
I do not have time now to post, but I want to say that, after reviewing again my calculations, I was able to arrived at the same values for I1, I2, I3 and I4, and V1, V2, V3, and V4 also with my calculations as ttokoro's answer, but only with 2 different signs for two currents: I3 and I1.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I get this:
Then:
and with:
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
On second view:
since both sources (Vi1 and Vi2) are DC, all voltages V1...V4 are 0 V, I2..I4 are 0 A and I1=24/7 A.
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
----
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Based on the previous calculations, I get the following:
Calculations that don't match yours.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Your above one is right. I2=-I1/n. Therefore, if I1 is 1.265+0.586i, I2 should be 3.795+1.757i, instead of -3.795-1.757i.
So, your Kirchhoff's current law, KCL, is not correct. And n is not 1/3 but 3 for n1 and n2.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
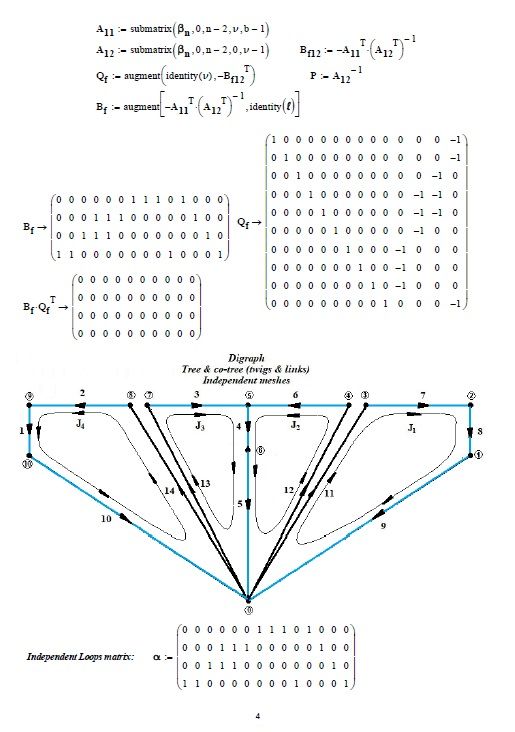
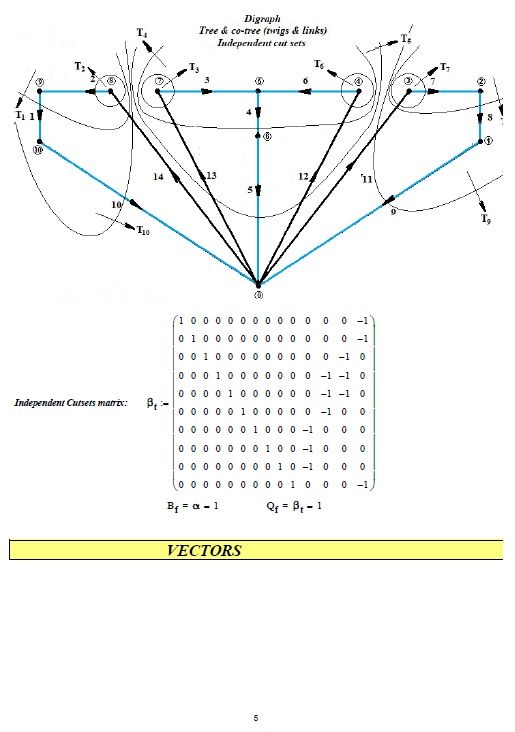
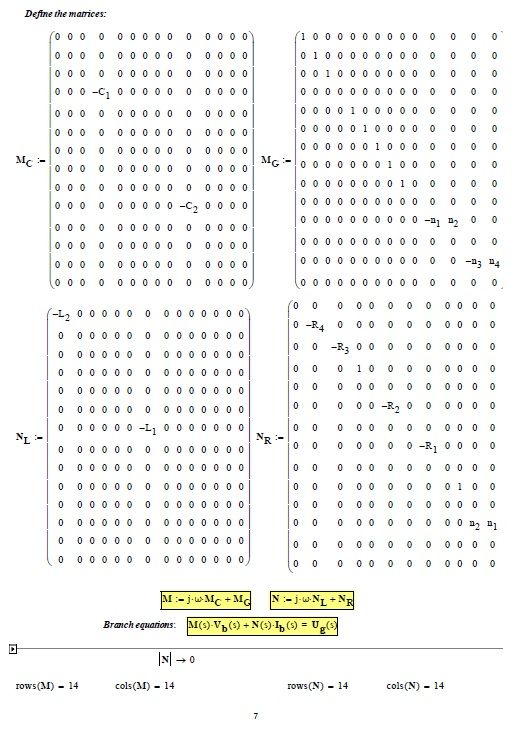
In my calculation procedure, I must respect the directions of the digraph currents. This means that if the current of a branch is negative, it has the opposite direction to that of the digraph. If you find current and voltage values different from mine, I would appreciate it if you would point out where the procedure is not correct.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
With all due respect, I think that the (latest) result I presented (on 2024-01-13, 11:59) is correct and follows the convention adopted by -MFra-:
- Voltages V1...4 are indicated by arrows pointing from + to -.
- Currents are indicated by arrows for the positive direction.
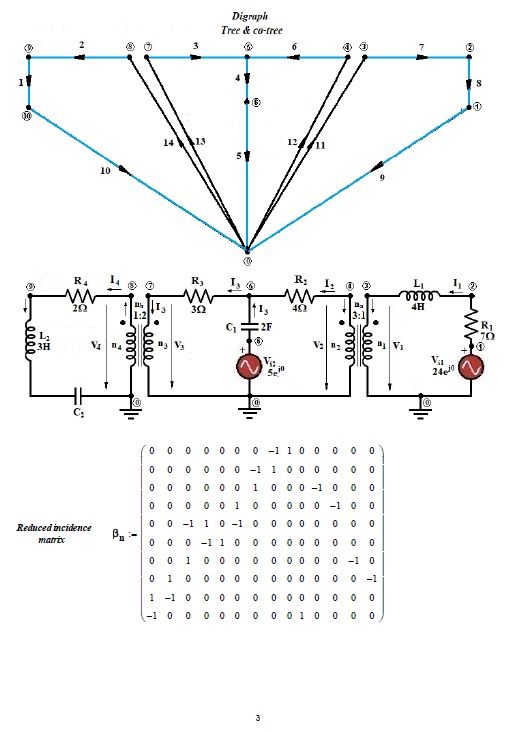
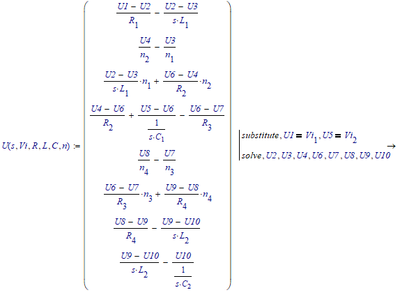
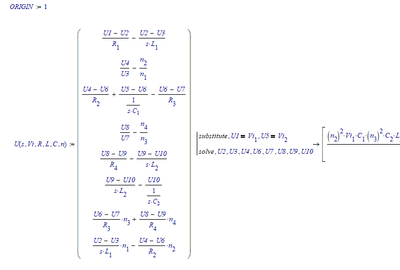
You should be able to easily follow the set of (8) equations that use the voltages of each of the nodes in the circuit numbered in circles (the node between L2 and C2 is number 10) named Ux in my equations. The direction of the currents is observed; current that flows into a node is positive, current that flows out is negative.
I solve the set for all unknown voltages (U2...4 and U6..10) and notice that V1=U3, V2=U4, V3=U7 and V4=U8, Then I calculate the currents I1..I4 by dividing the voltage difference across the resistors R1...4 by the respective resistor value.
Done.
The result equal to that of Cornel, except for signs of some values, that is because Cornel changed some directions of arrows.
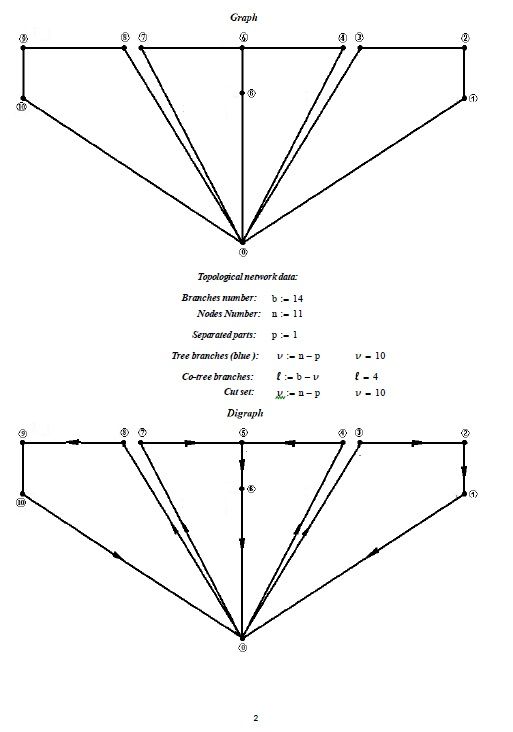
@-MFra-: Why is I3 in your very first schematic pointing to the left, while in the digraph it is pointing to the right?
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks for the reply. The circuit only served me to draw the digraph in which I1 and I3 appear with directions opposite to those of the circuit (but it would have been better to keep the directions of the circuit). In the following I have always referred to the verses of the currents of the digraph (directed graph).