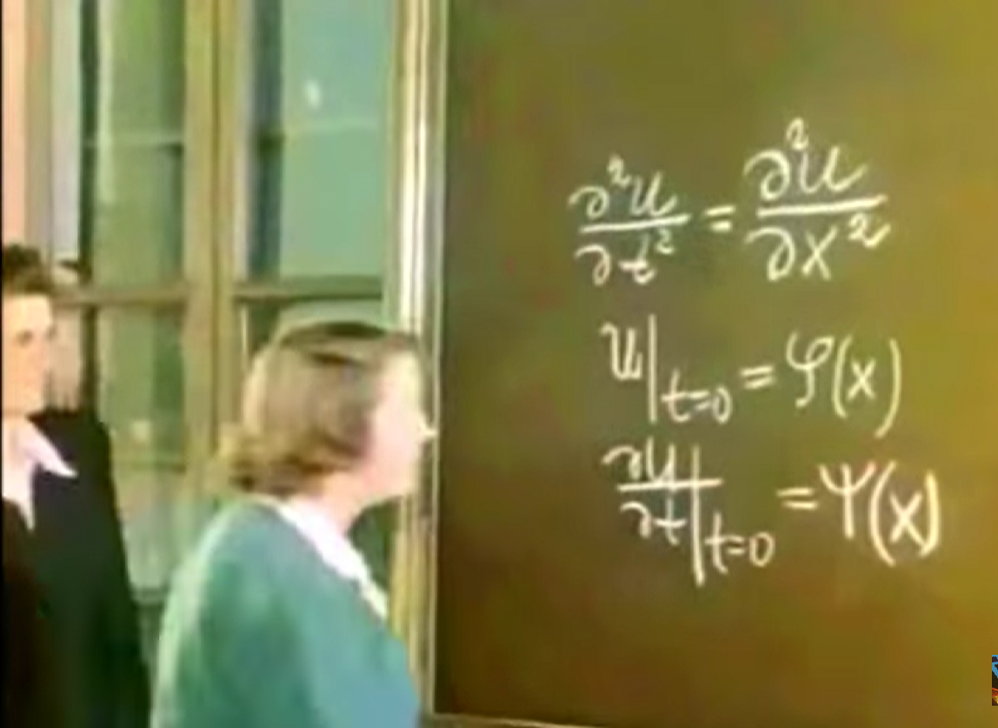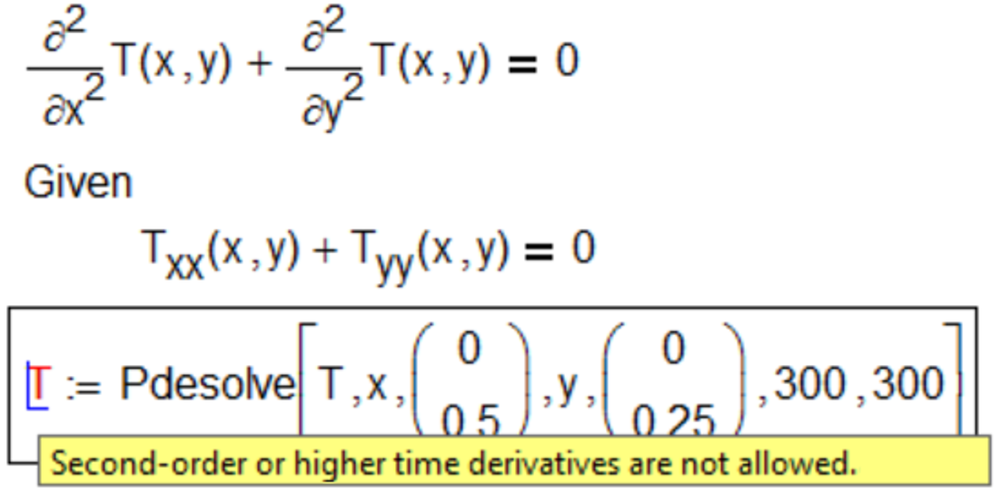Community Tip - Visit the PTCooler (the community lounge) to get to know your fellow community members and check out some of Dale's Friday Humor posts! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
PDE, Old newsreel math equations, 1950
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
PDE, Old newsreel math equations, 1950
I was looking through an old newsreel and saw such a frame: a university teacher gives a task to a student.
Mathcad cannot solve this equation. And can you?
- Labels:
-
Calculus_Derivatives
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
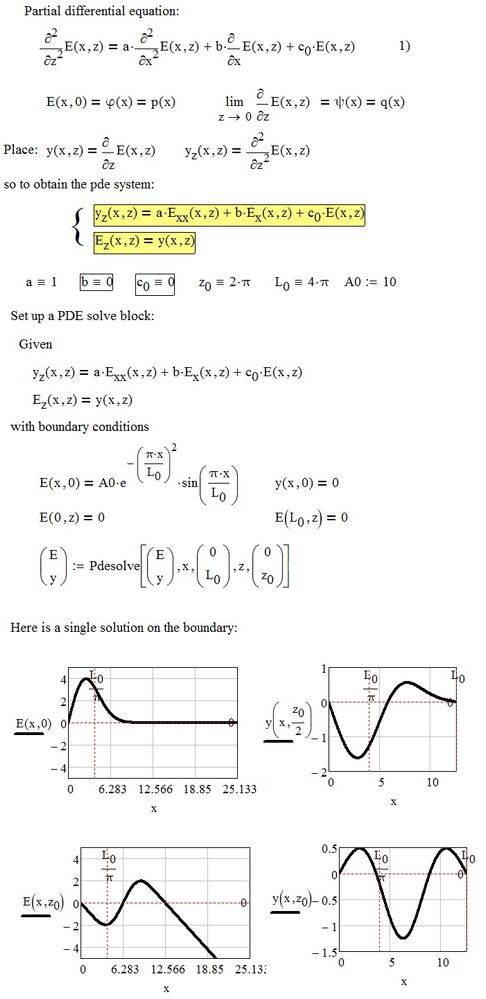
One Mathcad Prime solution
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Simple, the answer is:
U(x,t)=phi(x)+t*psi(x).
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Just to avoid typing Greek letters, let u(x,0)=p(x), ut(x,0) = q(x).
The proposed solution u(x,t)=p(x)+t·q(x) doesn't check, since utt(x,t) ≡ 0, while uxx(x,t) = p’’(x)+t·q’’(x).
The equation utt = uxx is the wave equation with velocity 1, so its general solution is u(x,t) = af(x+t) + bg(x-t) for arbitrary functions f and g, and constants a, b.
Add the initial conditions:
p(x) = u(x,0) = af(x) + bg(x), (1)
q(x) = ut(x,0) = af’(x) - bg’(x). (2)
Derivative of (1): p’(x) = af’(x) + bg’(x). solve with (2) to get
af’(x) = (1/2) [p’(x) + q(x)],
bg’(x) = (1/2) [p’(x) - q(x)].
Let h(x) be the integral of q(x). so that h’(x) = q(x). Then
af(x) = (1/2) [p(x) + h(x)],
bg(x) = (1/2) [p(x) - h(x)].
Then
u(x,t) = af(x+t) + bg(x-t) = (1/2) [p(x+t) + h(x+t) + p(x-t) - h(x-t)], where h'(x) = q(x).
Check:
u(x,0) = (1/2) [p(x) + h(x) + p(x) - h(x)] = p(x).
ut(x,t) = (1/2) [p’(x+t) + h’(x+t) – p’(x-t) + h’(x-t)],
ut(x,0) = (1/2) [p’(x) + h’(x) – p’(x) + h’(x)] = h’(x) = q(x).
Lou
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You're right Lou.
When substituting my U(x,t) into the PDE it didn't match. I should have done that before I answered (too quickly).
Your derivation is superb!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi,
I have long solved the equation given with pdesolve, what do you think?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks!
I would like to insert this text in new editions of my Russian and English book.
Can you translate it into English and/or add something?
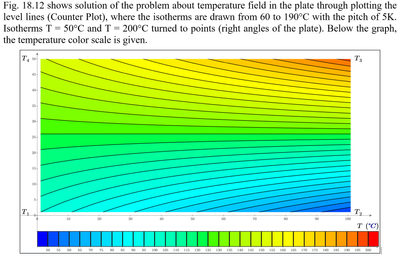
Задача о температурном поле плоской пластины, рассмотренная выше, была размещена на сайте пользователей Mathcad по адресу https://community.ptc.com/t5/PTC-Mathcad/PDE-Moscow-1950/m-p/613472 с просьбой найти аналитическое решение. У нас, как известно, решение было численное. Два посетителя сайта с сетевыми псевдонимами LucMeekes и LouP сделали попытку найти функцию двух аргументов, заложенную в уравнение, показанное на рис. 18.10. Предлагаем читателям оценить данное решение.
Ремарка. В настоящее время размещение задач на специализированных сайтах Интернета стало одним из способов их решения. У человека нет способности или времени решить возникшую производственную (но не учебную!) задачу, он «вывешивает ее на сайте и ждет, когда кто-либо решит задачу или покажет пути ее решения. С учебными задачами тоже можно так поступать, но с особыми оговорками в том плане, что я, мол, получил задание от преподавателя, а преподаватель сам не знает, как приступить к решению, или у него нет времени заниматься мною… Интернет-технологии сейчас широко используются в образовательном процессе.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
One numerical solution