- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Propulsion and Resistance
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Propulsion and Resistance
Hell to all,
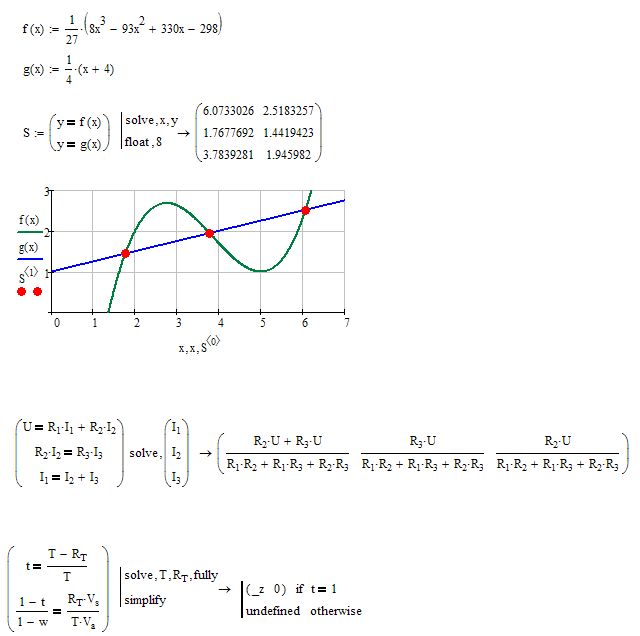
I am working on a bigger Ship Propulsion problem.
I have got to one of those scenarios where it feels like I am missing an input in order to get the ultimate solution.
Can anyone tell me if it is possible (by substitution) or otherwise (in the attached capture) to get values for either Rt or T if I have values for Vs, Va, w and t?
using MC's Symbolics it suggests not but it must be possible?
Thanks,
Andy.
- Labels:
-
Other
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Your equations can be reduced to the absurd
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Guys,
Thanks very much. I think I have (through reading the question again) worked another method.
I agree my post is not possible.
One thing I am interested in is MJG's definition of the two equations in square brakcets. How has this been done i.e. what is the region which the symbolic solve has then been applied to?
Andy.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Andy,
The square brackets are a 2x1 matrix.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
As Fred already pointed out - your system of equations is only solveable for T and RT, if 1/(1-w)=Vs/Va. And if thats the case, any values für T and RT are solutions, for which RT/T=1-t.
So chances are that there is anything wrong with your equations.
One thing I am interested in is MJG's definition of the two equations in square brakcets. How has this been done i.e. what is the region which the symbolic solve has then been applied to?
Thats the way systems of equations are solved symbolically in Mathcad. You write the equations one by one in a n x 1 matrix and solve for (ideally) for n variables, You may provide the latter comma separated or as a vector. The result normally is a m x n matrix, where m is the number of solutions.
Here are some examples: