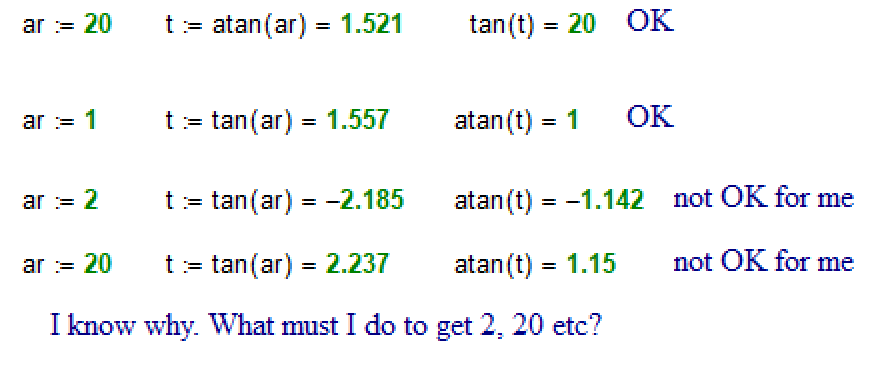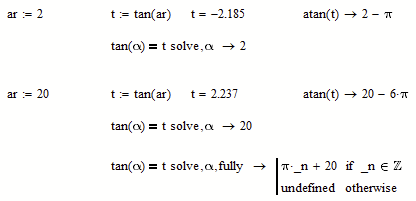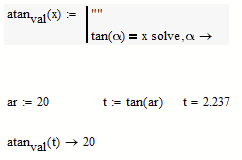Community Tip - Did you get called away in the middle of writing a post? Don't worry you can find your unfinished post later in the Drafts section of your profile page. X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Tan-Atan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Labels:
-
Algebra_Geometry
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Cannot be solved.
The tan function is periodic. A one-to-one relationship exists only if you limit the argument of the tan function between -pi/2 and pi/2.
In your 3rd and 4th lines that is not the case.
You'll find the very same problem if you change 'tan' to 'sin' in your expressions.
For 'cos' it is slightly different.
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Cannot be solved.
The tan function is periodic. A one-to-one relationship exists only if you limit the argument of the tan function between -pi/2 and pi/2.
In your 3rd and 4th lines that is not the case.
You'll find the very same problem if you change 'tan' to 'sin' in your expressions.
For 'cos' it is slightly different.
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I see
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If you use tan(..) then a lot of information about the angle is lost and can't be brought back.
The atan function is not the true inverse of tan but is limited per definitionem to the range [-pi; pi] to make it a unique function.
But if you don't use numeric inline evaluation when calculating t, symbolic solver "remembers" where it came from - at least as long as ar rational and not given as float value.
In real Mathcad you can create your own atan-function to do the job:
But it will only work if you evaluate it symbolically 😉
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks!
But если нельзя, но очень хочется, то можно. How it is in English?
I will show one solution fpr one my problema with ovals!
Now I am creating the animation!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
@ValeryOchkov wrote:
Thanks!
But если нельзя, но очень хочется, то можно. How it is in English?
Not sure, maybe something like "What does not fit is made to fit" or "You can achieve anything if you really want to" ?
In fact, my suggestions weren't entirely serious. There is hardly a way to get Mathcad to read your mind to guess what angle you actually are looking for.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
My solved problem with tan and atan