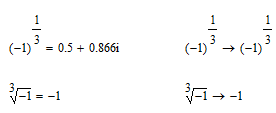The power of 1/3
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The power of 1/3
Look at this picture, and how to explain it? I use MC15 and MC 2001, the same the answer.
Solved! Go to Solution.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
zhu laojianke wrote:
Is there anyway I can get this answer without using symbolic calculation?
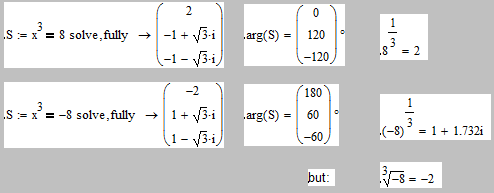
Nothing I could think of. And the symbolics won't help you - I just used it to get all solutions of my equation and show why Mathcad (and most other math programs I know) chose a complex one in case the argument is negative.
I have to change all the power expression to root expresstion to get the whole graph of the function.
That seem very troublesome.
If it would be a built-in function you could write your ownt and overrule Mathcads one, but in case of the exponentiation operator you can't. So any solution would require a rewriting of the expression. But at least in Mathcad with the root symbol you have an alternative which you don't have in many other programs (because its mathematically doubtful but helpful from an practical engineering point of view).
To state it clearly: Mathcad is perfectly right in doing what it does here! (-1)^(1/3) is NOT -1 per definition!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
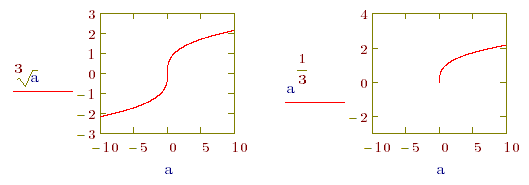
a^(1/3) defaults to the solution of x^3=a with the smallest positive argument.
Therefore if a is negative the result is a complex number which can not be plotted
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Is there anyway I can get this answer without using symbolic calculation?
When I plot or calculate a more complex algebraic expression, such as:
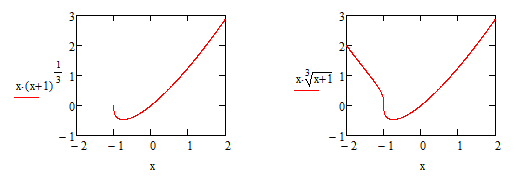
I have to change all the power expression to root expresstion to get the whole graph of the function.
That seem very troublesome.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
zhu laojianke wrote:
Is there anyway I can get this answer without using symbolic calculation?
Nothing I could think of. And the symbolics won't help you - I just used it to get all solutions of my equation and show why Mathcad (and most other math programs I know) chose a complex one in case the argument is negative.
I have to change all the power expression to root expresstion to get the whole graph of the function.
That seem very troublesome.
If it would be a built-in function you could write your ownt and overrule Mathcads one, but in case of the exponentiation operator you can't. So any solution would require a rewriting of the expression. But at least in Mathcad with the root symbol you have an alternative which you don't have in many other programs (because its mathematically doubtful but helpful from an practical engineering point of view).
To state it clearly: Mathcad is perfectly right in doing what it does here! (-1)^(1/3) is NOT -1 per definition!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
OK, I'll take care of modifing my expression when I need to research a total graph of a function in the future. It seems that there are lots of difference in logic between computor calculation and mind thinking.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Without plot: