Community Tip - Have a PTC product question you need answered fast? Chances are someone has asked it before. Learn about the community search. X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
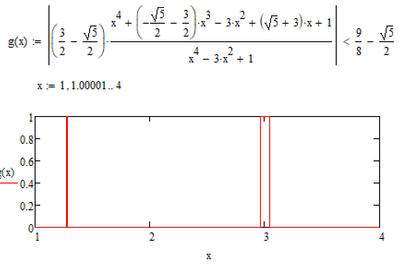
"No solution was found". What does it mean in this case ?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
"No solution was found". What does it mean in this case ?
Hello, Everyone.
From :
"No solution was found". What does it mean in this case ?
Regards.
Solved! Go to Solution.
- Labels:
-
Math Homework
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
One plot solution
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Valery, your plot is missing two of the four solution intervals.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
@lvl107 wrote:
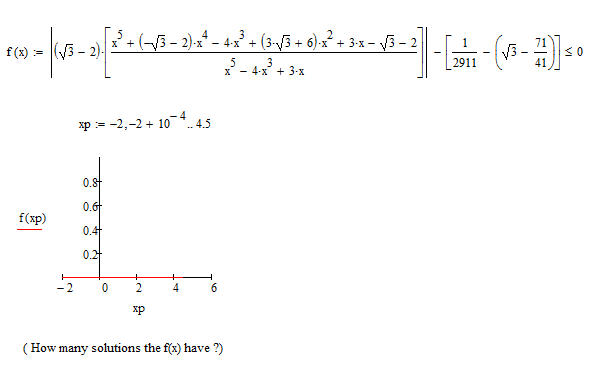
and how many solutions the f(x) have ?
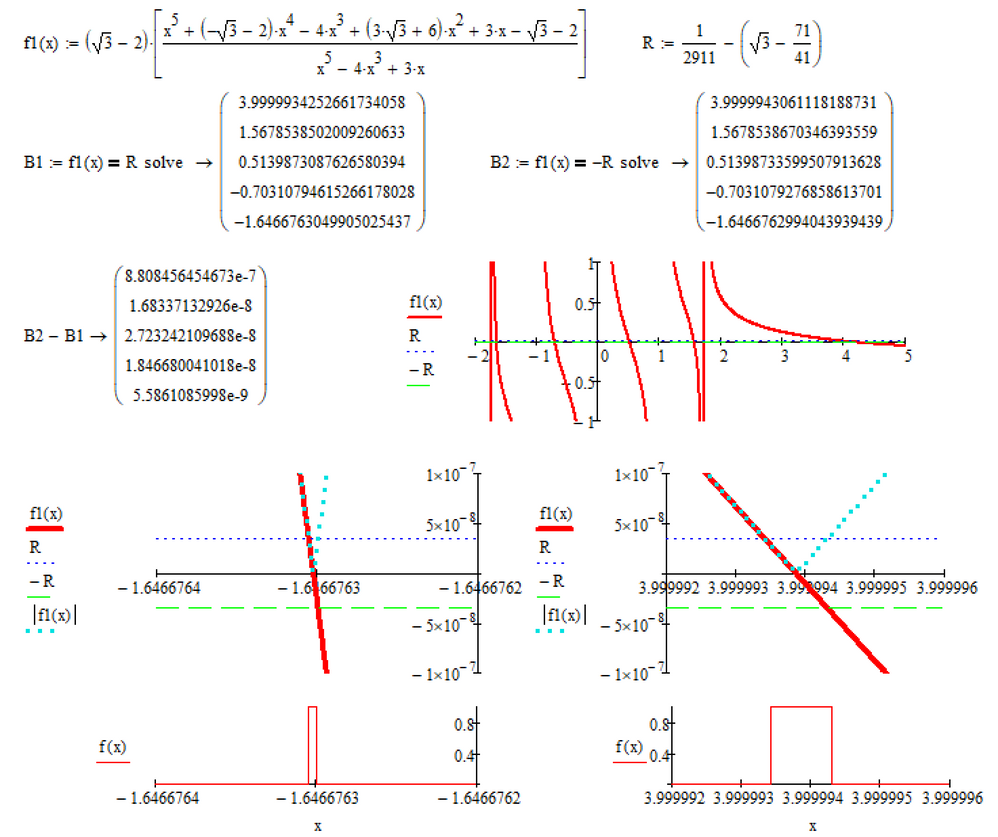
Five!
But the length of the five solution intervals are very small (in the range of 10^-8) so you may run into numerical problems when you try to plot.
Because five is an odd number, now B1 in the picture below consists of all the lower limits of the solution intervals and B2 holds all the upper limits.
The intervals you are interested in are the tiny areas between the points where the red curve crosses the dotted blue line (slightly above zero) up to the point where it crosses the green line (slightly below zero).
The picture shows a zoomed view for the first and the last solution interval.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
One additional remark:
The absolute value usually is quite beastly - either when it comes to solving equations and inequalities and also when it comes to derivatives. So its always a good idea to avoid the absolute value and either replace the calculations by separate calculations (like I did it above to get the limits of the intervals) or use squaring instead of the absolute value (see below).
BTW thats also one reason why in regression analysis we use the squares of the errors instead of their absolute value - the latter would be far more difficult to handle.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Many thanks for your time and help again, Werner ![]()
![]()
From :
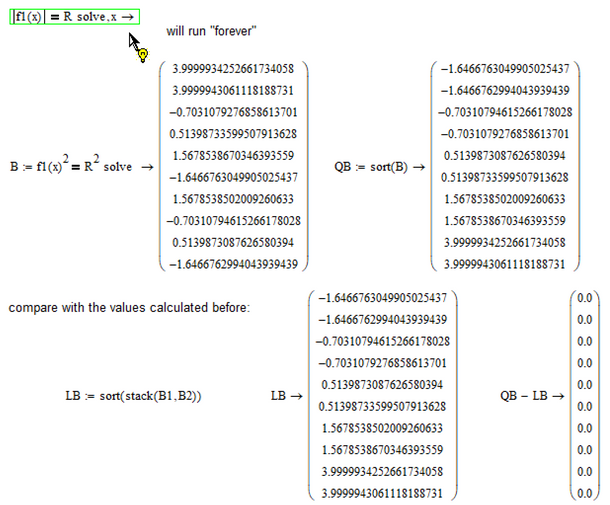
It seems my MC15 runs it forever !?, if so, is there any way to solve it ?
Best Regards.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
and by the way, please help me how to make my the graph ( on the right ) like as yours ( on the left ).
Best Regards.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
@lvl107 wrote:
and by the way, please help me how to make my the graph ( on the right ) like as yours ( on the left ).
Best Regards.
Not sure what you mean. Your plot looks pretty much like mine. The region is differently sized, the number of intervals/grids on the abscissa is differently (its 9 in my plot, I guess) and I had chosen axis style "Boxed" instead of "Crossed".
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Oh, Werner.![]()
![]() Your program command (in the prior post) say "NaN", and I guess is : "No solution was found"
Your program command (in the prior post) say "NaN", and I guess is : "No solution was found"
Best Regards.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
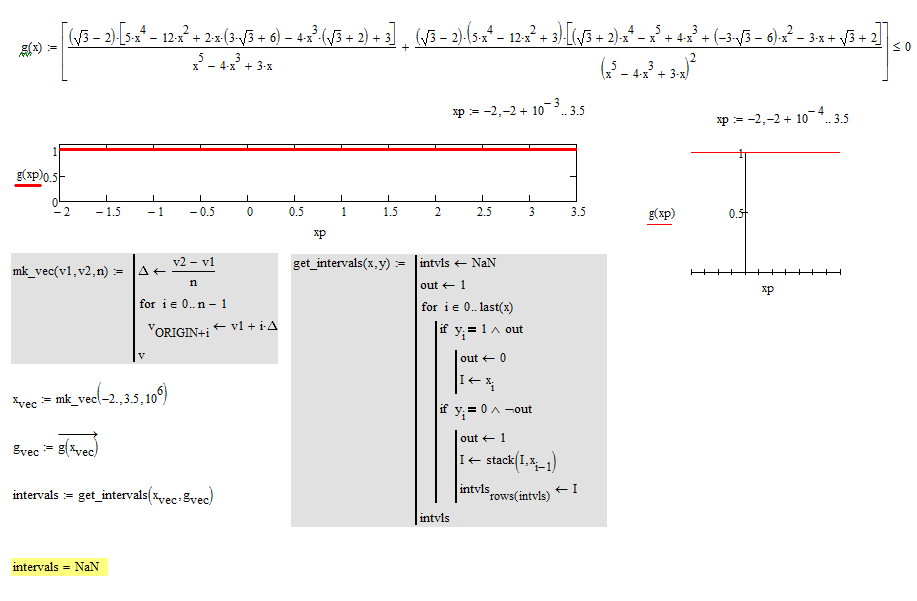
Yes. If you look how the function is written you will notice that it only returns an interval if it encounters a zero value (so it can determine the rightmost limit of the interval).
The range you examine has only value 1 throughout so the program finds no end of interval.
The program could be changed so it would assume the last value in the range to be the end of the interval.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Not sure what you are trying to do.
f1(x) is zero most of the times with few rectangles now and there.
So the derivative would be zero over the full range with a few Dirac spikes where f1 jumps from 0 t0 1 or the other way round.
The numerics sure can't deal with Dirac and the answer to d f1(x)/d x=0 would be "everywhere".