Community Tip - You can change your system assigned username to something more personal in your community settings. X
- Community
- Creo (Previous to May 2018)
- Creo Modeling Questions
- Re: Modelling a Reef Knot
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Modelling a Reef Knot
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Modelling a Reef Knot
Hello,
I have, unfortunately, a requirement to model a Reef Knot (or Square Knot, as you might know it) again.
In the past, I have made a couple of wiggly curves orthogonal to each other and intersected them and run a VSS along them to create each half loop. A few mirrors and you have a passable looking knot. It takes a lot of tweaking and fiddling to get it looking presentable, though. Then there was the added complication that I was required to model a Slip-Reef Knot - one with a loop on one side that you could pull to undo the knot, a bit like a shoelace.
Is there an equation (or equations) to define such a knot? The nice thing about the equation approach would be that it took less work to make it look good and it would also scale nicely. That way, it would be done once and for all and I wouldn't have to repeat all the tweaking every time.
I'm sure someone out there has sorted this out.
Currently using WF4, M220, but I would imagine the principle applies equally well to any version. Bear in mind that I won't be able to open your solutions created in Creo 2.0, though...
Cheers,
John
Solved! Go to Solution.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I made it.
X=.45*cos(t)+.25*cos(-t)-.45*cos(-3*t)
y=.45*sin(t)+.25*sin(-t)-.45*sin(-3*t)
z=0.2*sin(5*t)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
If anyone would have it, it would be http://mathworld.wolfram.com/SquareKnot.html
It doesn't look like anything useful for modeling, though the topology is OK.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Fun problem. It should be just a matter of parameterizing the knot and plugging the equations into a datum curve by equation.
That being said, I have no idea how one might go about parameterizing a knot. ![]()
The guy who made this website does. He has parametric equations for a granny knot which is similar to a reef knot.
http://paulbourke.net/geometry/knots/
I plugged the following into a datum curve:
x = -22*cos(t) - 128*sin(t) - 44*cos(3*t) - 78*sin(3*t)
y = -10*cos(2*t) - 27*sin(2*t) + 38*cos(4*t) + 46*sin(4*t)
z = 70*cos(3*t) - 40*sin(3*t)
And with a sweep I got this:
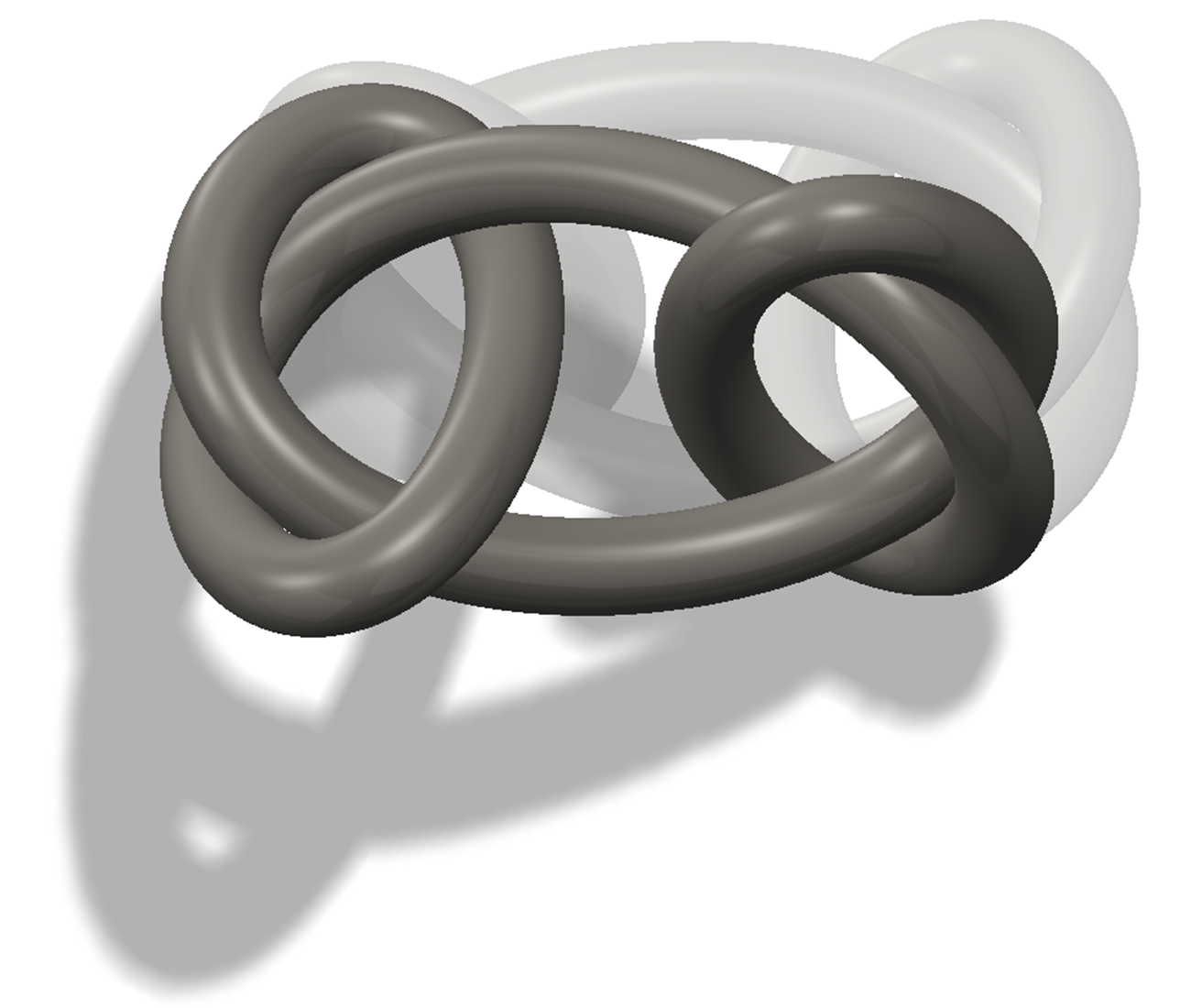
Maybe you can tweak these equations to form a reef knot. I might work on this later.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I made it.
X=.45*cos(t)+.25*cos(-t)-.45*cos(-3*t)
y=.45*sin(t)+.25*sin(-t)-.45*sin(-3*t)
z=0.2*sin(5*t)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Whoa, cool!
Done by "curve by equation" I take it?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Frank, yes curve by equation.
It's not a big thing, I think I just had more successful google searching.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Figured. I thought this would be cool to use for a coiled wire rope model..... ![]()
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
yeah it would, hopefully someone will work on that, I dont really have the time at the moment.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Nice work Matt!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Search for "Instructional Knot Tying Animations (Blender)" and view in wonder.
No doubt it's not trivial work, but still amazing.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Amazing, indeed.

