Community Tip - Did you get an answer that solved your problem? Please mark it as an Accepted Solution so others with the same problem can find the answer easily. X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
ASYMPTOTE
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
ASYMPTOTE
Hello,
Please see the attached MC 15 worksheet.
I need help in finding:
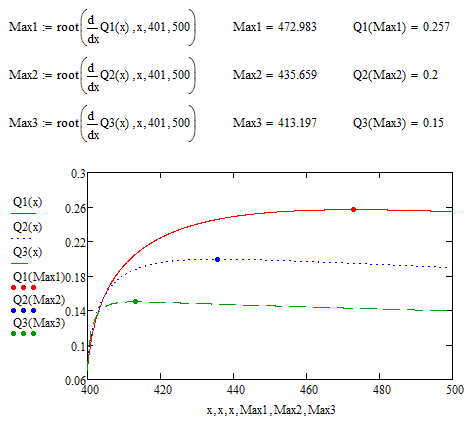
1- Maximum values of Q1(x), Q2(x) and Q3(x)
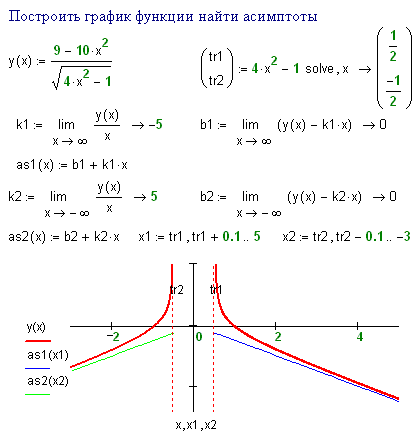
2- Asymptote to y(x), z(x) and Z(x) (How can we verify that these curves have horizontal ASYMPTOTES?)
Thank you so much.
Anousheh
Solved! Go to Solution.
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
ad 1) you could use root and the derivative, as odesolve returns sort of a pseudo-functions (vector of points but implicit interpolation) from which you can take the derivative and use root on it. See attached.
ad 2) you can't. The "functions" you have are only valid for 400<=x<=1000, so you can't explore what happens if x approaches infinity (which would be necessary to prove that a horizontal asymptote exists). The best you can "prove" is that all available values Z(x) are smaller than Z(1000) - thats no prove for a limit of course.
To verify a horizontal asymptote you would need Z(x) in an analytical form, that means you will have to solve the DE manually or with the help of Laplace, if possible. Then you could take the limit x-->inf and see what happens.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
ad 1) you could use root and the derivative, as odesolve returns sort of a pseudo-functions (vector of points but implicit interpolation) from which you can take the derivative and use root on it. See attached.
ad 2) you can't. The "functions" you have are only valid for 400<=x<=1000, so you can't explore what happens if x approaches infinity (which would be necessary to prove that a horizontal asymptote exists). The best you can "prove" is that all available values Z(x) are smaller than Z(1000) - thats no prove for a limit of course.
To verify a horizontal asymptote you would need Z(x) in an analytical form, that means you will have to solve the DE manually or with the help of Laplace, if possible. Then you could take the limit x-->inf and see what happens.

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Valery, do you really think thats of any help here?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner Exinger wrote:
Valery, do you really think thats of any help here?
Werner, do you really think thats of any help here?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Valery Ochkov wrote:
Werner Exinger wrote:
Valery, do you really think thats of any help here?
Werner, do you really think thats of any help here?
I'm not sure. If it helps avoiding inappropriate posting in the future, the answer is yes, if not - no.![]()
No offence intended, but its sometimes really hard to follow a thread if its cluttered with "side postings" not related to the question other than it seemed to was triggered only by the mention of a single term ("asymptote" in this case). No hard feelings, anyway.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello Valery,
Thanks for the information. I am trying to see if my functions, y(x) have any horizontal asymptote.
Werner correctly pointed out that:
The "functions" you have are only valid for 400<=x<=1000, so you can't explore what happens if x approaches infinity (which would be necessary to prove that a horizontal asymptote exists). The best you can "prove" is that all available values Z(x) are smaller than Z(1000) - thats no prove for a limit of course.
To verify a horizontal asymptote you would need Z(x) in an analytical form, that means you will have to solve the DE manually or with the help of Laplace, if possible. Then you could take the limit x-->inf and see what happens.
Nevertheless, your information is really good for constructing other type of asymptotes. Thank you.
Anousheh
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello Werner,
Thank you so much for the input. Very helpful.
All the best,
Anousheh


