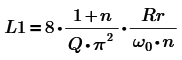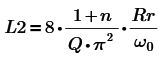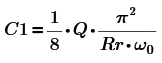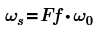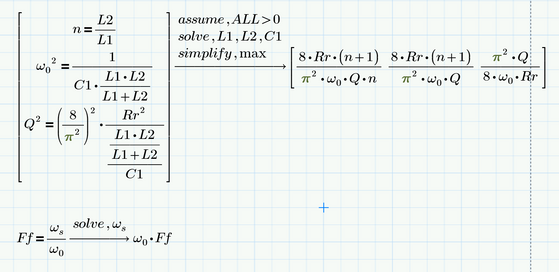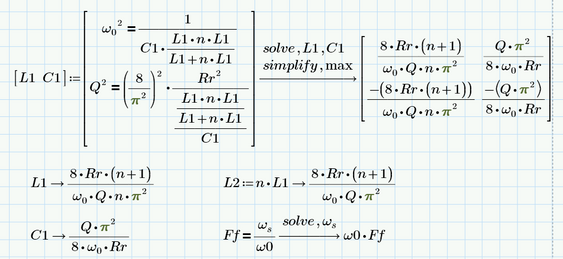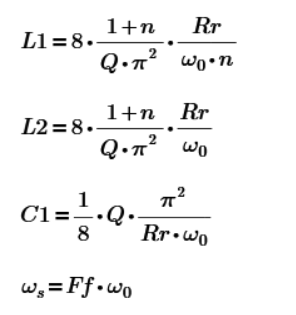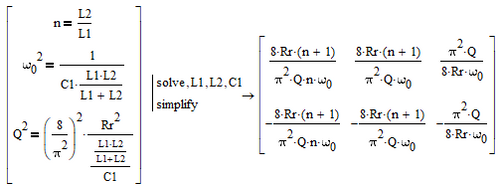Community Tip - Learn all about PTC Community Badges. Engage with PTC and see how many you can earn! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
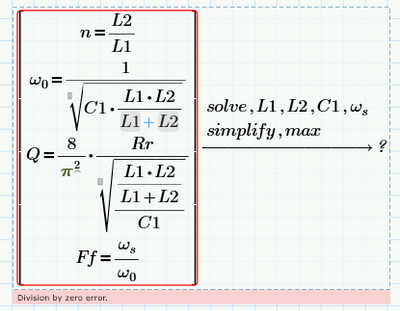
Division by zero error - Mathcad Prime 7
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Division by zero error - Mathcad Prime 7
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi,
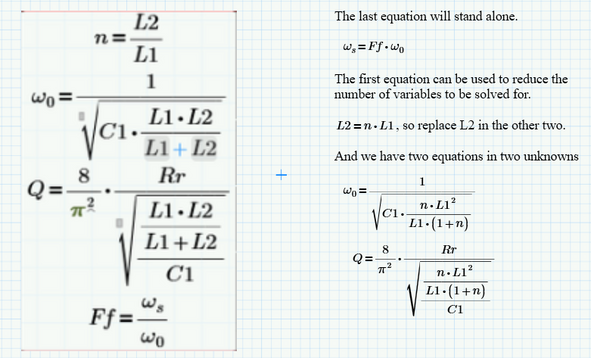
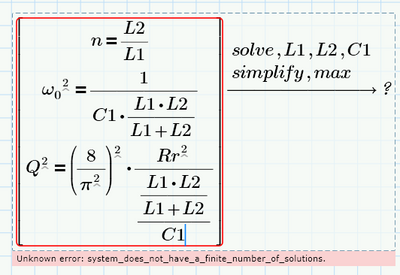
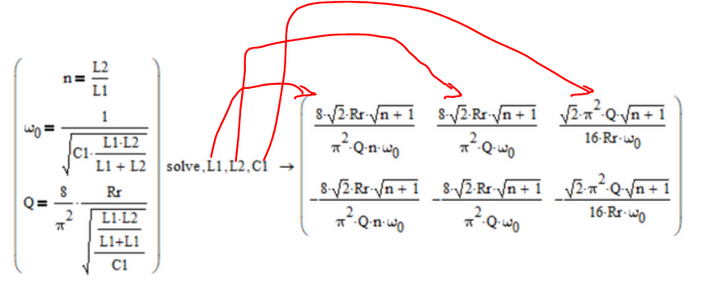
First and last equations of the four can be rearranged and substituted into second and third equations meaning only two independent equations are used to find four variables and this is not possible.
Cheers Terry
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
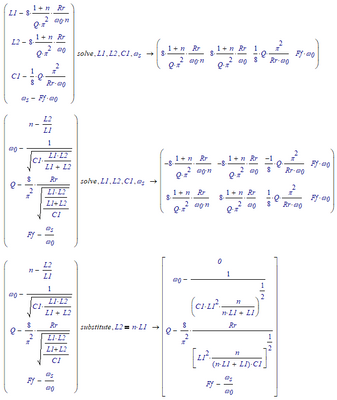
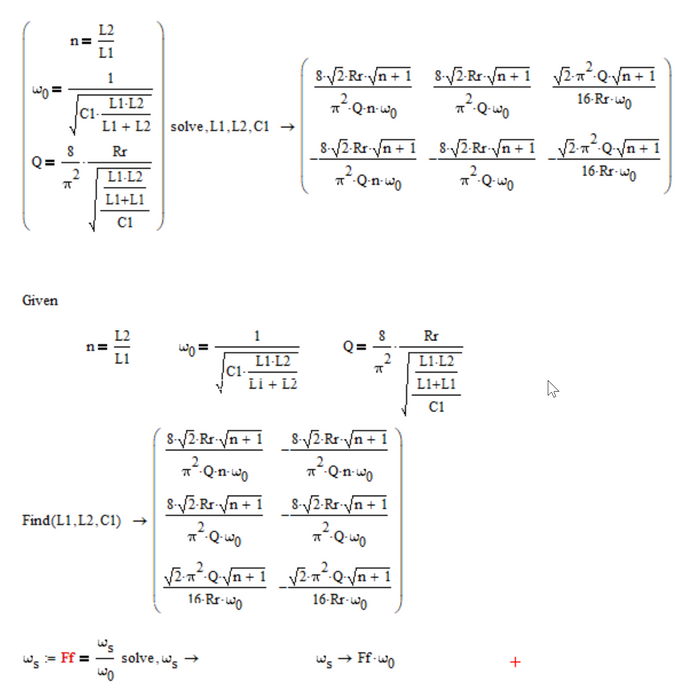
Here are the solutions obtained in Mathcad 11 with above 4 equations and 4 unknown variables (L1, L2, C1, ws):
So, these solutions I want to obtained also in Mathcad Prime 7.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So, these solutions I want to obtained also in Mathcad Prime 7.
Rule of thumb: If you wan to see the result which Maple (the symbolics in MC11) is able to deliver, you should use Maple!
Maple in MC11 was much superior compared to muPad in later versions (up to Prime 6). And muPad on the other hand seems to have been superior to Axom/FriCAS which now is used for symbolic calculations in Prime.
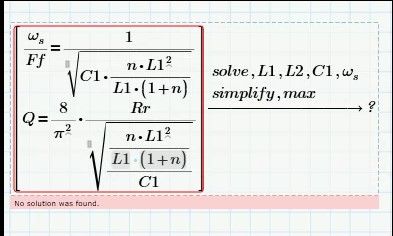
In Prime 6 we can switch over to muPad and also muPad cannot solve your system as it is. But if we do without the last equation (which is independent from the others) and if we help muPad (squaring the two equations to get rid of the roots) it is able to deliver the desired result.
But I was not able to talk the new symbolics into delivering a similar result.
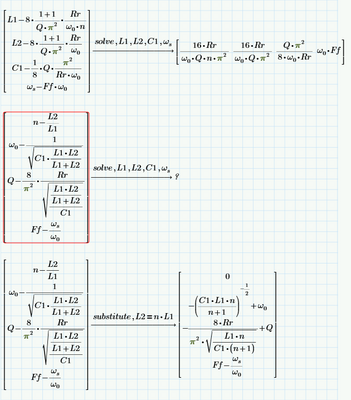
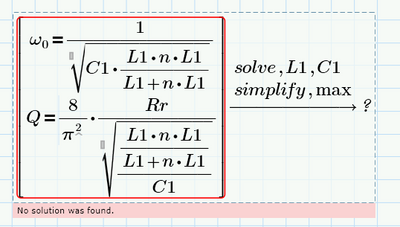
Maybe friCAS in Prime needs even some more manual help, maybe replacing L2 by n*L1 as Fred showed can help. But then why should we use the symbolic engine at all if we have to solve half of the system manually ourselves?
The point is that it looks to me that you are not using the right tool for the problems you'd like to solve. Your various questions here in the forum ever so often show that the symbolic in Prime simply is not capable enough for your needs even if here and there we could find a clumsy workaround to elicit a reasonable result from Prime after all.
Its sad but things got worse from Maple to muPad to friCAS.
Feel free to report this problem here as a bug to PTC support. Then maybe in 10 or 20 years .... who knows?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Well, sorry Terry, let's rethink this a bit:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
To add to the discussion:
Mathcad 11:
Prime 7:
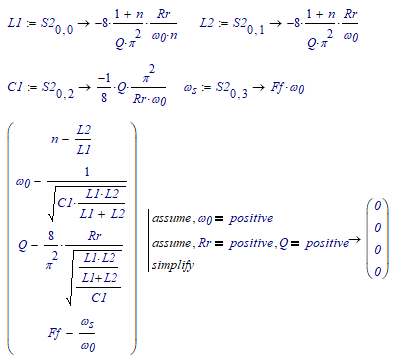
That error is the 'division by zero' error.
To show the Mathcad 11 result for the second set is valid (given positive values for all knowns):
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Just to dot an i
Maple (Flow) still can generate the answer:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Mathcad 15 also has no problems (if we omit the last, independent equation, Neither using the symbolic "solve", nor by symbolically evaluate a solve block with "find".
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
This is what Mathcad Prime (8) says 🙂
Hm...it's fine, even manual in some way for this job...
The big problem is with the other topic with those big powers of s (s^30, etc.). I will post about this thing...
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
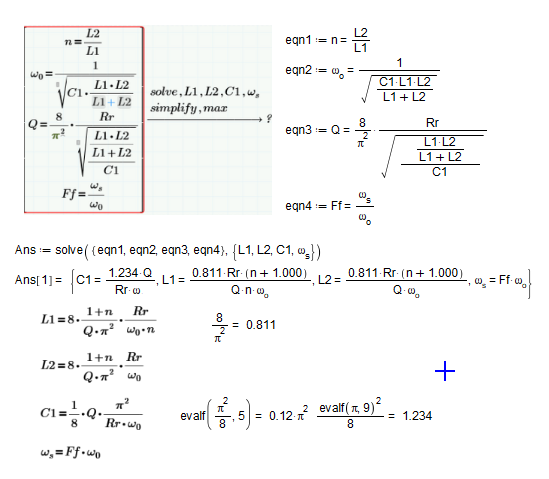
Something seems wrong here...
The correct expressions for L1, L2 and C1 are:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Oops! I had a typo in the last equation (L1+L1 instead of L1+L2) 😞
After correcting this MC15 fails to find a solution. Only after getting rid of the roots we get a solution
So (not surprisingly) it shows the same behaviour which I already had shown with Prime and the legacy engine.