Is already know this oddity ????

[-MFra-]

[Werner_E]

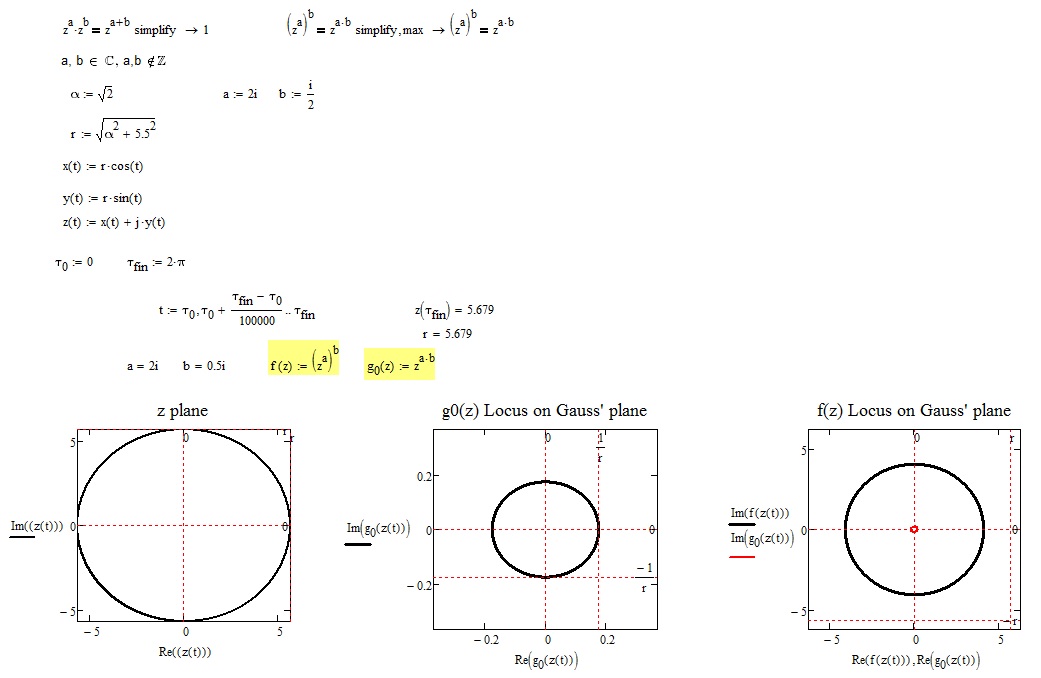
Oddity? Not sure what you mean and then its just a pic, not a sheet to play with.

Exponentiation is not unique in the set of complex numbers. a^b has many results and Mathcads numerics choses one of the many possible "solutions" and which one it chooses obviously depends on how you write that expression.

[-MFra-]

Hi Werner,

I wanted to say, strangeness. I trust the google translator, who translates it, first and foremost, as a oddity.

  About the test function, it  is a  monodroma (it generates a single value for each z) holomorfic and analytic function, whatever you write it.

Instead mathcad draws incredibly two different locus on the Gaussian plane. I think it is yet another bug.

Greetings

FM

[Werner_E]

I won't call it a bug. After all is the rule (a^m)^n=a^(m*n) not necessarily fully valid in the set of complex numbers.

And Mathcad is not really good when it comes to expressions with multiple results (the numerics chose one specific and the symbolics often isn't aware of more solutions) - you may look at some older threads of LoiLe which covered that subject sometimes in more depth.

The solutions you get using either of those two expression differ by a factor of e^pi and both are valid.

[-MFra-]

My mistake was to consider z ^ q = exp (q * ln (z)) a monodroma function, when, instead, it is a multivalued function for non-integer q.

[Werner_E]

F.M. wrote:

My mistake was to consider z ^ q = exp (q * ln (z)) a monodroma function, when, instead, it is a multivalued function for non-integer q.

It IS! As long as z is real and positive and q is real.

[-MFra-]

For q non integer namely q is real or complex, z^q is multivalued, while for q integer z^q is single valued.

Greetings

[Werner_E]

> For q non integer namely q is real or complex, z^q is multivalued

with the exception of the definition in R, when q>0 and z>0 - only in this case its unique. e.g. 4^(1/2)=+2 and only +2 per definition.

But as its clear now the confusion was that you used complex numbers and exponentiation is not always unique in that case and Mathcad feels free as to which value to chose.