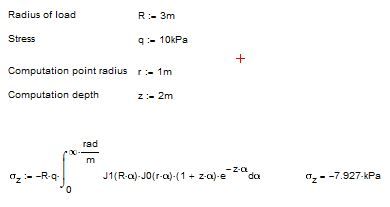Community Tip - You can Bookmark boards, posts or articles that you'd like to access again easily! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Stress below circular loading
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Stress below circular loading
Hello
I'm trying to compute the stress under circular load (not only below center line, but below any point).
I have found the attached article about the topic, but I wasn't able to make the computation indicated in formula 3c. The units doesn't seem to match. (http://onlinepubs.trb.org/Onlinepubs/hrr/1963/39/39-004.pdf)
I have found an old mathcad computation sheet about the problem, but the reference to these formulas is unclear.
The attached sheet includes some of my tries to solve this problem based on the attached article.
But my feeling is that I'm not even close...
Thank you for your help in advance.
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you for your quick answer.
With this change you proposed the computational stress values seem to match with the
older mathcad sheet, which was as attached in that zip file.
Could you, or someone else, explain why the infinity is divided with meters?
I mean, yes the result is apparently correct, but the reason for strange unit 1/m for the upper and lower
integral limit is unclear to me...
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Mathcad requires "unit balance". The integral terms consist of terms in the form R times alpha. R you have given the dimension meters, but R times alpha needs to be unitless or the Bessel functions won't evaluate. So, giving alpha the units of 1/meters allowed the program to evaluate. (It was genius!)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Note that you can use any unit of length since the following are true:
0m = 0cm = 0ft = etc...
∞m = ∞cm = ∞ft = etc...
Or rather, in the example given, these equalities are more applicable:
0/m = 0/cm = 0/ft = etc...
∞/m = ∞/cm = ∞/ft = etc...
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks for the comments and guidances.
As the integration formula are done to evaluate stress distribution under axisymmetric loading,
could it be that the "more correct" integration limit is infinity with units [rad/m].
The resulting stress of course remainst the same as with [1/m].
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Be sure that the unit rad is defined as 1 ( unitless)
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Yes, I agree
So the formula becomes as follows:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Yes,
note that physically/mathematically this is incorrect, because also the lower limit of integration has a unit, and must have the same unit as thye upper limit.
However mathcad is a bit forgiving in that it will apply the correct unit to any 0 it finds/needs.
Luc