ACCEPTED SOLUTION
Accepted Solutions
Oh, I agree that it would stand remarkably little chance of succeding (at least in English and Scottish law, not sure about USA - seems to be almost watertight compared to some of the law suits I've read about 
).
I also agree that somebody at PTC should have got it right in the first place - they must surely have looked at a copy of the SI/ISO/NIST SP?
Stuart
In Prime (3.1) it is easy to change the font of the unit symbol to non-italic, but I fully agree that it should have been upright from the start.
There IS a space between the value and the unit, see L=15 m below, and that is the only option for ISO 80000-1.
However it only appears in the evaluation, when the cursor is away; not in the definition. I think that may be a breach. In Mathcad (no Prime) there was an option to choose a space for the multiplication symbol, haven't found it in Prime yet.
Note that ISO 80000-1 allows no space (if it does not lead to confusion mN <=> Nm for newton metre), a space an x or a half-height dot for a combination (multiplication) of units, Prime uses the half-height dot (the T=1 kg*m example).
Luc
The following is an excerpt from the “International System of Units”:
1.1 Quantities and units
The value of a quantity is generally expressed as the product of a number and a unit. The unit is simply a particular example of the quantity concerned which is used as a reference, and the number is the ratio of the value of the quantity to the unit.
The last phrase could read as: "and the number is the number of units that gives the value of the quantity."
For example, the value of a length may be expressed in the form x = 3 m = 9.84 ft, where m and ft are alternative units for obtaining the same value of the length.
Quantity: x or length
Value of the quantity: 3 m
Unit, i.e. a particular value of the quantity used as a reference : m (m is usually regarded as a base unit)
Number of units: 3
Hence, the value of the length is ‘3 times a meter’ or ‘9.84 times a foot’.
___
The value of a speed v may be expressed in the form v = 25 m/s = 90 km/h, where m/s and km/h are alternative units for obtaining the same value of the speed.
Quantity: v or speed
Value of the quantity: 4 m/s
Unit, i.e. a particular value of the quantity used as a reference: m/s (m/s is usually regarded as a derived unit)
Number of units: 4
The value of the speed is ’25 times a meter per second’ or ’90 times a kilometer per hour’.
___
In Solid Mechanics, also called Strength of Materials, a strain is the measure of a change in length measured over a reference length. The value of a strain espilon may be expressed in the form espilon = 0.005 m/m = 0.005 in/in = 0.005.
The fact that the derived unit m/m is the ratio of two units of the same kind, i.e. units of length, makes strain a dimensionless value. Although ‘strain’ is not a SI units, it is common to use it to indicate that the quantity is a strain. For example, "espilon = 0.005 strain" or more often "espilon = 5000 μstrain" (i.e. microstrain). Note that μ is the prefix micro = 10-6 and therefore is written in roman. (SI Brochure, Section 3.1)
___
A geometric plane angle, simply called an angle, is ratio of the length of a circular arc to its radius. Similarly to the unit of a strain, the unit of an angle is the ratio of two units of the same kind, i.e. units of length (although one is measured along an arc and the other is measured along a radius), which makes angle a dimensionless value.
Because angles are such a common quantity, its dimensionless derived unit m/m = 1 is given the special name radian, where radian is equal to the ratio of 1 m measured along a circular arc to its radius that is 1 m long.
___
Obviously a strain and an angle are two different quantities even if they both happen to have the same derived unit, in this case m/m = 1 called a dimensionless derived unit.
See the “The International System of Units” for more on this subject.
___
I think that mathematicians are more concerned about numbers and the mechanics of counting, calculating, differentiating, etc., whereas physicists and engineers are concerned about quantities, i.e. numbers and units. This is not self-contradictory.
___
Back to the main point of this thread.
I was hoping that someone at PTC Mathcad would say something like: “Dear Dominique, You may have a point. We will consider your suggestion and may modify the factory default setting of the font for units to roman (upright, not bold, blue color) in the next release of Mathcad." 
Dominique Bauer wrote:
A geometric plane angle, simply called an angle, is ratio of the length of a circular arc to its radius. Similarly to the unit of a strain, the unit of an angle is the ratio of two units of the same kind, i.e. units of length (although one is measured along an arc and the other is measured along a radius), which makes angle a dimensionless value.
No it's not. That may be how we determine it's numerical value in radians (assuming we measure both lengths in the same unit), but angle is not simply a ratio - as is common with many other quantities, there is no way to distingush quantities of the same dimension from each other, yet even a five-year old can recognize an angle realize that it's not quite the same thing as saying two lengths are different. To my mind, the official definition is putting the cart before the horse or chicken before the egg. And therein lies the heart of the problem. We have a history of using things like trig functions in a dimensionless fashion and, being both institutionally lazy and reactionary, this means that we are loath to change, or even think about changing, all of our nice equations to be correct under an assumption of angle being a distinct dimension.
Dominique Bauer wrote:
Back to the main point of this thread.
I was hoping that someone at PTC Mathcad would say something like: “Dear Dominique, You may have a point. We will consider your suggestion and may modify the factory default setting of the font for units to roman (upright, not bold, blue color) in the next release of Mathcad."
I see Canada still produces some great stand-up comedians! ....
(
"Units" are just one of many long-standing point-of-debate amongst Mathcad users:
Re: Additional Units for Mathcad Prime 3.0
Re: Additional Units for Mathcad Prime 3.0
As is ISO naming/style adherence:
)
Stuart
Hi Philip. And all.
First: Angles are not the quotient of segments, nor it's rate. That a new (sic) technique to measure them. Quotient it's an algebraic operations, and angles (and segments) have nothing to do with math at the level of elemental things, like those that a 5 years old human can recognize as distinguishable "things".
Second: Also, angles are not dimensionless, have a dimension.
Lets to fundament that. Angles are geometrical entities, so, we must to define angles in therms of geometrical elements, probably some very primitive ones: Plane angles are the intersection of two coplanar half-planes. where a halfplane is defined by "primitive concepts", which have no definition, as they figure in the Euclid's axioms, which we take as "true". Planes, points, lines are primitive concepts. Spatial angles are the intersection of two half-spaces. So, there are not distances in this definition, and, obviously, no units yet.
In the attached page, from "Geometría métrica", of Puig Adams, spanish mathematician, and the book that I use at high school, you can read the definitions for "magnitud" and "cantidad" (quantity), and angles and lengths as magnitude examples. Notice that "métrica" came from μέτρον (metron) which means "measure".
So, every set that meets properties 1 to 4, they elements define a magnitude. It's posible to prove that angles verify properties 1 to 4. But we now have a complicate set of theoretical elements. How can take the "angles" from it? That's important, and not specified in the only one book page: by a "representant" of his class. The situation is similar with Q, the set of the fractions, We have a lot of candidates for represent 5/2 = 10/4 = 15/6 = .... But take only one, definid by the property of that div(num,den)=1. So, that's the quantity: those elements that have the same amount (cantidad) of this magnitude.
Note also that mass can be defined meeting this 4 properties too, from m=F/a, where the force and the acceleration can be measures by the elongation of a spring, and acceleration measuring times and lengths. For check that mass are well defined, must to prove that this relation verifies properties 1 to 4.
In that procedure there are no place for the Higg's boson. Also, for times, we can use pendulums. For measuring angles we don't need segments, actually we need half planes, but it's more easy consider the half-lines, defined by a line, a point over the line, and a orientation. The border of an angle, are the set of two half lines. (Border as a topological operator, represented by δ, and actually have a lot of simulates with the mathematical derivative operator). Notice also that in the referred book, the word for the "angle" quantity is "amplitud", which is the measure for the defined magnitude.
Once we have the magnitude, we can let now to see which units we want to use. For example, we can divide the circle into 360 "sectors" (that's the correct name for the circle "parts"), by purely geometrical procedure, without any calculus, and compare that with the "amplitude" of a particular angle, to measure it.
Or can use length of segments to measure angles. But remembering always that angles have nothing to do with the math quotient operation. That's like the Pythagoras theorem: in geometry you don't squaring numbers, but take the geometrical square built over the sides of the triangle.
Some few final considerations, if the reader if not enough convinced. Angles and sides, point and lines, and other couples for space, are dual elements: can be interchanged at any geometric statement (theorem, property or axiom). So, at each statement that mentions length and magnitude, you can interchange it with angles and get reamains magnitude. See Duality (projective geometry) - Wikipedia, the free encyclopedia . For that, they can take as Generalized coordinates - Wikipedia, the free encyclopedia .
Best regards.
Alvaro.
Hi Philip.
My wrong. By two excuses: First, You post was the last when I post mine, so the "answer" was to you. Even I don't like the new definition for angle, just because isn't one, it's only a measure technique, new (sic) one, I know very well your position about angles, and agree with you on it from a long time ago. Actually, some of your old post about angles and units make me to re-study this point.
Second, and as excuse to, I write "Second, Also ...", but want to write, "Second, and as Philip say ..." or something like this, but stop with also, which is very short.
So, I don't refuses your points, because I agree with them, except, (always there are some but) with the correct definition process for some measure of angles, but this is a minor point, just about how to define an angle.
About the sin, or cosine functions, one can take different path: geometry, as segment projection, analytic, as functional properties (from the definition of the exponential as the function that holds f(a*b) = f(a)*f(b) and then use the Euler relations to introduce sin and cos), calculus, as the solution of some diff equation, or limit for some series, and a big etc.
So, for saying that sin(2 kg) make sense or not, must to say for which definition is that. For real numbers, one can prove that almost of the usual definitions for sin gives the "same" function, but remember that the extension for complexes, as an example, defined by sin(z)=(e^iz-e^-iz)/2 it's only a convention. Identify the vertical projection with the sine function was a convention too, derived of the representation of real numbers in a line. For example as what I'm saying, try to represent the sine function if you represent reals as the unidimensional position in a Peano curve, which cover a plane region.
But for make this conventions, at least, there are some considerations, for example, can ask for some coherence. If sin(2 kg) have sense, in the functional definition for sin, you have this:
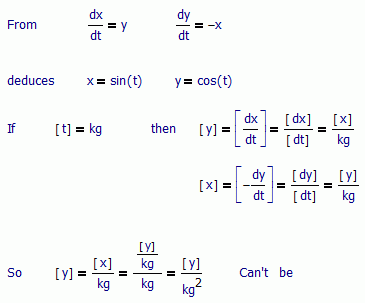
So, with this definition of sin, cos, can't take kg as argument. With this definition, you must to use some unit where [t]^2 = 1. Oh, [rad]^2 = 1:
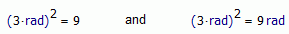
What convenient!
So, also agree with you that could be very difficult to use sin, log nor exp with some other unit, or dimension than a angle.
Best regards.
Alvaro.
