- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
A classical math problem: differential equations
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
A classical math problem: differential equations
Here is the Mathcad 11 sheet that solves nth order differential equations with constant coefficients, referenced in the document thread with similar name here: A Classical Math Problem from a Classical Text (Differential Equation).
Note that this, as it is, is NOT usable for:
- Systems of differential equations,
- Differential equations where the coefficients of the derivatives are not constants (when they depend on t).
But in both cases, similar techniques can be used to solve specific systems.
Luc
- Labels:
-
Calculus_Derivatives
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Nice, but unfortunately it will not work in any version of Mathcad past version 11. The way you are using symbolic math in that first program was never officially supported, and was removed in version 12. Even if that could be fixed in some way, the Mupad symbolic engine can't handle the invlaplace expression ![]()
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
In my original post in this thread I was using Mathcad Prime 3.0. My career with Mathcad is long. I think I started many years ago with Mathcad 3 way before PTC entered the picture. My math challenges were relatively simple algebra problems, so I didn't get into much calculus. In my retirement I'm exploring areas where necessary math skills are more demanding. LucMeekes and Richard Jackson bring up topics unfamiliar to me. The bottom line, however, seems to be that in addition to Mathcad, pencil and paper are still necessary.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You can have Mathcad do most of the manipulations and some solving, even "abiding by the rules".
Then you still shouldn't need to use pencil and paper:

Prime should be able to do the same, using it's symbolics.
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
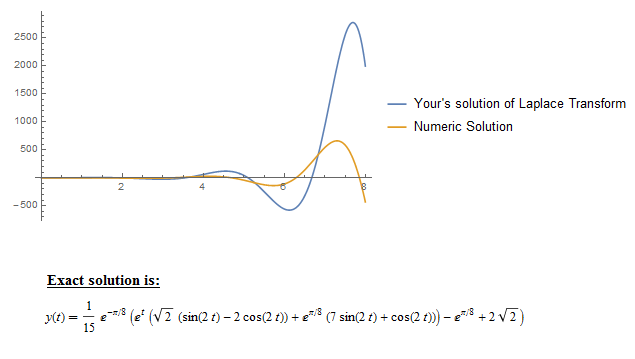
Your's solution is wrong. !You have to check numerically , or otherwise.You can't use this initial contitions like y(Pi/8)=1 only zero in brackets like that:
- y(0)=(any here), y ' (0)=(any here) ,y ' ' (0)=(any here)....... etc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I think that Thomas and the textbook and myself have come to the correct resulting function,
but you're right: It's best to check the result:
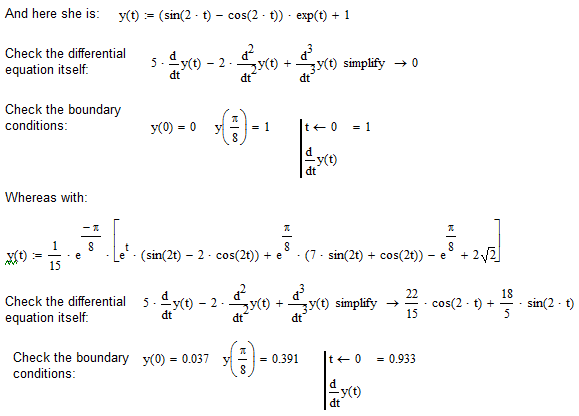
Your definition of y(t) does not simplify the given differential equation to 0,
and the boundary conditions are different.
What did you use to get that symbolic result, and what did you use for the numeric result?
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I'm not convinced that the original questions have been resolved. Is there an easier way, and is pencil and paper necessary? I just finished the course on Signals and Systems at Rice (Discrete Time Signals and Systems, Part 1: Time Domain | edX) and (Discrete Time Signals and Systems, Part 2: Frequency Domain | edX). I passed the time domain part and flunked the frequency domain part. I made the mistake of trying to do all problems using MATLAB and never resorted to pencil and paper. It turns out that half the problems were meant to be solved using pencil and paper. MATLAB cannot index vectors using zero or negative numbers. I don't know what the situation is with Mathcad, but my attempts to use Mathcad's signals functions indicated that I have a lot to learn. My bottom line is still - if you are working with numerical methods it is best to have numbers and not a lot of variables. Many thanks for the interesting comments and elucidations.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi, Luc,
May I ask the reason that you collect the inverse laplace with sin(2t), cos(2t) and exp(t) in this step:
Is it because you found the term on the left side can be traced back to the basic laplace transform like laplace(sinat)= a^2/(s^2+a^2)?
Or collect the inverse laplace with sin(2t), cos(2t) and exp(t) is a universal method in this step?
Thank you for your help!
Shawn
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Jan Kowalski wrote:
Your's solution is wrong. !
Sorry, but it seems that yours is wrong!
You have to check numerically
Agreed on. So check yours and we get y(pi/8)=0.552 and not 1 as it should be!
You can't use this initial contitions like y(Pi/8)=1 only zero in brackets like that:
- y(0)=(any here), y ' (0)=(any here) ,y ' ' (0)=(any here)....... etc
Yes, we need the values for t=0 when doing Laplace. But it looks like you haven't noticed that Luc replaced y''(0) (which we don't know) by a generic c and solved later, after doing invlaplace, for c to fulfill y(pi/8)=1.
As you don't show how you arrived at your wrong solution we cannot tell you where you failed.
LT
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ah, I see that I have to correct. forgot the sqrt(2) before the sine. Correction:
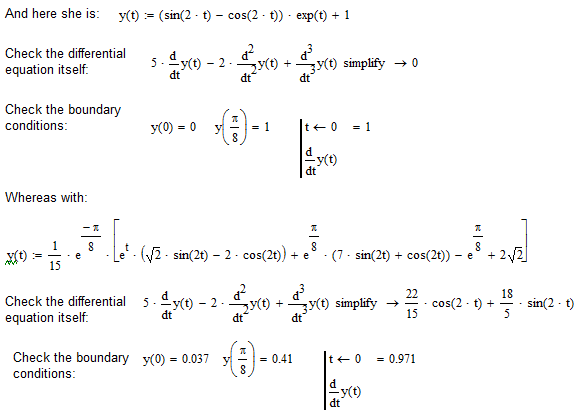
{Leopold, where does your 0.552 come from?}
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
{Leopold, where does your 0.552 come from?}
You have again mistyped Jan's "solution". You have your parenthesis wrong.
With the correct expression all conditions (equation, y(0), y'(0)) but y(pi/8) are OK.
Can't post a screenshot as I had not saved the sheet.
But in the meantime Jan has found his error and came up with the same solution as you did.
LT
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ah, these parentheses.
OK, Now I see that Jan equated y''(pi/8)=1:

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I had a little mistake.Correct one is:
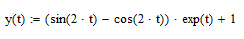
Even his  made a mistake.
made a mistake.![]()
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You are a genius!





