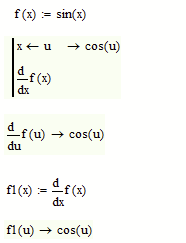Differentialquotient gesucht
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Differentialquotient gesucht
The real-valued function f(x) and its differential quotient df(x)/dx are given. In MC14, this differential quotient for the symbolic value u has to be calculated symbolically. What is the syntax for this? I ask for help.
Kind regards, Alfred Flaßhaar
- Labels:
-
Calculus_Derivatives
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Not sure what you actually are looking for.
Is this coming somewhere close?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
MC 14 is exclusively about symbolic calculations. We know that the real-valued function f(x) is continuously differentiable on the real interval a<= x <=b. This first derivative, as the differential quotient df(x)/dx, is also a function on this interval. At point u from the interval, f(x) has the symbolic value f(u). How do you use MC14 to calculate the symbolic value of df(x)/dx at position u?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Still not clear to me what you would like to see.
Better prepare a worksheet and don't forget to show what you would like Mathcad to return at the end
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Here is the "test". I would like to use the symbolic calculation in higher order of differential quotients too. How is the symbolically calculated value of the differential quotient at point u taken into account as a constant in further/subsequent symbolic calculation?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
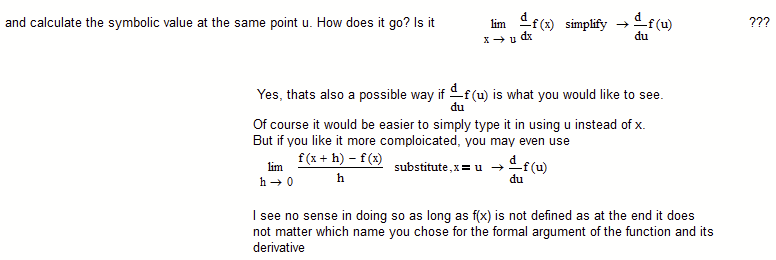
I think you still don't show what you really would like Mathcad to return. But anyway ... lets assume you did in the sheet and all you were looking for is seeing the differential quotient with x substituted by u ...
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The background to my question is a sentence named after Völler:
In the Euclidean plane, a function f(x) is given as a graph of a four-fold continuously differentiable function and on it the points M and N as well as the tangents in these points with their intersection point T. Let F denote the area of the triangle MNT and G denote the segment area under the curve m above the chord of length MN. The ratio G/F = 2/3 must be calculated for the limit case MN ---> 0.
(Source: Archive of Mathematics and Physics, editor Johann August Grunert, 31st part of 1858, pp. 449-453, "About a strange general theorem about curves", author: Andreas Völler)
What is interesting about this theorem is that G/F=2/3 is independent of the specification of a concrete function f(x). The continuous differentiability is sufficient for this result. The original proof is in principle not difficult. The Bernoulli/L`Hospital rule is repeatedly applied. However, considerable paperwork is required. And I'm trying to simplify that with the help of MC14. Since the proof result is independent of the specific specification of a function f(x), the symbolic calculation ability of MC14 should be applicable. And that's my attached problem.
(By the way, I found a simpler proof that works in MC14.)
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
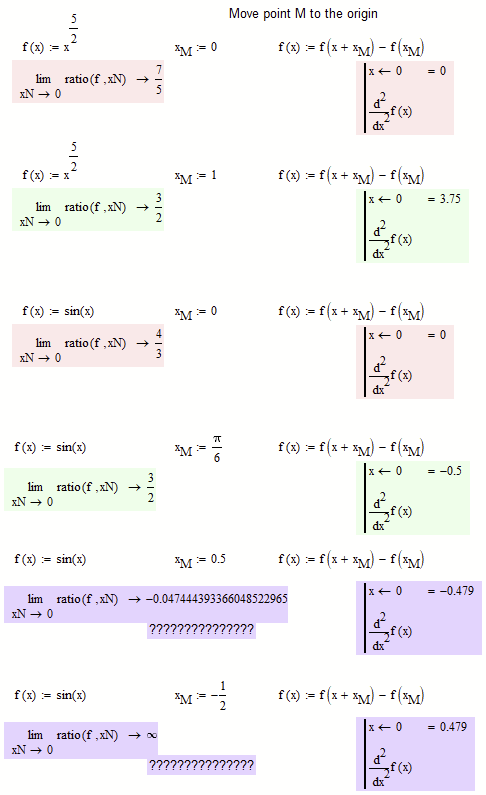
We had this theorem already in a former question of yours if i remember correct and I already wondered at that time why you marked the question as answered as, so far that I could tell, it wasn't. You wrote there that all is OK now and you just made a minor mistake but I had the impression that you could not arrive at 2/3 with the approach shown. As far as I remember you had the first point a the origin (which was OK IMHO) but you also assumed the second one on the x-axis (which I thought not being OK).
When I glance at the new sheet you posted here, I see, that you still not arrive at that desired value, right?
So maybe a calculation software is not the suitable tool to make a formal, general proof ?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hab ich da etwas falsch verstanden oder eine (vielleicht im Text nicht genannte) Voraussetzung für den Satz verletzt?
Wo ist da mein Denk- oder Rechenfehler?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The proof in the original paper tacitly assumes that at the edges of the closed interval MN the second derivatives of f(x) are non-zero. This is only apparent at the end of the evidence published by Völler and was not mentioned in the sentence formulated at the time. Otherwise, after differentiating four times under the Bernoulli/L'Hospital rule, nothing could be reduced in the result break. In the case y=x^3 at point M the second derivative is 6*x=0.
I should have added this to my question - sorry.
Translated by the Community Moderation using Google Translate
Der Beweis in der Originalarbeit setzt stillschweigend voraus, daß an den Rändern des abgeschlossenen Intervalls MN die zweiten Ableitungen von f(x) ungleich Null sind. Dies ist erst am Schluß der von Völler veröffentlichten Beweisführung erkennbar und wurde im damals formulierten Satz nicht erwähnt. Andernfalls würde sich nach viermaligem Differenzieren im Rahmen der Bernoulli/L´Hospital-Regel im Ergebnisbruch nichts kürzen lassen. Im Fall y=x^3 ist im Punkt M die zweite Ableitung 6*x=0.
Dies hätte ich in meiner Frage noch ergänzen müssen - sorry.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
OK, diese Zusatzforderung, dass die zweite Ableitung an der betrachteten Stelle (der Grenzwert des Verhältnisses ist ja eine lokale Größe) nicht verschwinden darf, erklärt die vielen "Gegenbeispiele, die ich fand.
Hab Mathcad versucht zu überreden, das allgemein durchzurechnen, aber bin dann letztlich gescheitert:
Möglicherweise scheitert es daran, dass man Mathcads Symbolik kaum beibringen kann, dass f(0)=0 gilt und dass f''(0) != 0 ist.
Aber immerhin kann man mit dem Blatt den Grenzwert für konkrete Funktionen an jeder beliebigen Stelle x.M ermitteln.
Das Versagen bei den letzten beide Beispielen kann ich mir allerdings nicht wirklich erklären.
Wie sieht dein (wie du schriebst funktionierender) Beweis mit Mathcad aus?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Attached is the requested proof. It is based on Weierstrass's approximation theorem. I left out the “epsilontics” because I was interested in its feasibility in MC14. The approximation eps>0 is a constant property throughout all calculation steps. Unfortunately I cannot open *.xmcdz files with MC14. The last two examples interest me.
Translated by the Community Moderation using Google Translate
Beigefügt der gewünschte Beweis. Er stützt sich auf den Approximationssatz von Weierstrass. Die "Epsilontik" habe ich weggelassen, weil mich die Machbarkeit in MC14 interessierte. Die Näherung eps>0 zieht sich als stetige Eigenschaft durch alle Berechnungsschritte. Leider kann ich Dateien *.xmcdz mit MC14 nicht öffnen. Die beiden letzten Beispiele interessieren mich.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks,
MC14 should also have no problems with xmcdz files and should be able to open them without any problems. Ultimately they are just zipped xmcd files and MC14 itself can create them. When switching from MC14 to MC15, nothing significant was changed, just bug fixes, etc. PTC had taken over MC14 from Mathsoft and probably just wanted to make a mark with the higher version number, which was actually not substantially justified.
Anyway - attached is the file in the xmcd version.
The incorrect results in the last two examples are certainly due to Mathcad and the way in which symbolic evaluations are carried out internally.
P.S.: Your approach with f(x):=x*(s-x)*f(x) and the subsequent border crossing s-->0 is not a proof for arbitrary functions, is it? Ultimately, it only applies to functions of the type f(x)=- x^2*g(x) - or am I seeing something wrong?
As already written above, I was irritated in your first thread on the topic that you assumed that the second (variable) point under consideration was also assumed to be the zero point, since the border crossing changes the function under consideration.
Translated by the Community Moderation using Google Translate
Danke,
Auch MC14 sollte keine Probleme mit xmcdz Dateien haben und sie anstandslos öffnen können. Es sind letztlich ja nur gezippte xmcd Dateien und auch MC14 selbst kann solche erstellen. Beim Wechsel von MC14 auf MC15 wurde nichts Wesentliches geändert, nur Bug Fixes, etc. PTC hatte ja MC14 von Mathsoft übernommen und wollte mit der höheren Versionsnummer, die substantiell eigentlich nicht gerechtfertigt war, wohl nur eine Duftmarke setzen.
Wie auch immer - im Anhang die Datei in der xmcd Variante.
Die fehlerhaften Ergebnisse der letzten beiden Beispiele sind sicher Mathcad und der Art, wie intern symbolische Auswertungen vorgenommen werden, geschuldet.
P.S.: Dein Ansatz mit f(x):=x*(s-x)*f(x) und nachfolgendem Grenzübergang s-->0 ist doch kein Beweis für beliebige Funktionen, oder? In letzter Konsequenz gilt er nur für Funktionen der Bauart f(x)=- x^2*g(x) - oder seh ich da was falsch?
Es hat mich schon, wie bereits oben geschrieben, bei deinem ersten Thread zu dem Thema irritiert, dass du ach dort angenommen hast, dass der zweite betrachtete (variable) Punkt auch als Nullstelle angenommen wurde, da damit der Grenzübergang ja die betrachtete Funktion verändert.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The product approach for f(x) does not restrict generality. According to the theorems of Bernstein and Weierstrass (Natanson: Theory of Functions of a Real Variable, pp. 117-120), the continuous functions on a closed interval are f(x) = x*(s-x)*g(x) and g(x) e.g. B. can be approximated by Bernstein polynomials Bε(x) with a specifiable accuracy ε > 0. Accordingly, there is a polynomial sequence pn(x) that extends to [0 ; s] converges uniformly to f(x). Using the equation pn(x) = x*(s-x)*gn(x), the function gn(x) can be clearly determined by comparing coefficients using the polynomial approach for gn(x). Since {pn(x)} converges, the sequence {gn(x)} generated in this way must also converge and, according to Hausdorff's theorem (Langenbach: Lectures on Higher Analysis, p. 33), have a limit g(x) as a continuous function . The equation in the approach f(x) = x*(s-x)*g(x) is therefore on the interval [0 ; s] with this precision ε as sup |f(x) - x*(s-x)*g(x)| < ε can be satisfied in convergence. The limit value 2/3 to be determined is therefore represented as a constant sequence {2/3}. Detailed functional analytical proof by examining convergent polynomial sequences is omitted here, since the focus is on computational proof in MC14 based on the original proof.
Translated by the Community Moderation using Google Translate.
Der Produktansatz für f(x) schränkt die Allgemeinheit nicht ein. Nach den Sätzen von Bernstein und Weierstrass (Natanson: Theorie der Funktionen einer reellen Veränderlichen, S. 117-120) sind die auf abgeschlossenem Intervall stetige Funktion f(x) = x*(s-x)*g(x) und g(x) z. B. durch Bernstein-Polynome Bε(x) mit vorgebbarer Genauigkeit ε > 0 approximierbar. Demnach gibt es eine Polynomfolge pn(x), die auf [0 ; s] gegen f(x) gleichmäßig konvergiert. Durch die Gleichung pn(x) = x*(s-x)*gn(x) ist mit Polynomansatz für gn(x) die Funktion gn(x) durch Koeffizientenvergleich eindeutig bestimmbar. Da {pn(x)} konvergiert, muß auch die so erzeugte Folge {gn(x)} konvergieren und nach dem Satz von Hausdorff (Langenbach: Vorlesungen zur höheren Analysis, S. 33) einen Grenzwert g(x) als stetige Funktion besitzen. Die Gleichung im Ansatz f(x) = x*(s-x)*g(x) ist demnach auf dem Intervall [0 ; s] mit dieser Genauigkeit ε als sup |f(x) - x*(s-x)*g(x)| < ε in Konvergenz erfüllbar. Der zu bestimmende Grenzwert 2/3 stellt sich daher als konstante Folge {2/3} dar. Auf detaillierten funktionalanalytischen Nachweis durch Untersuchung konvergenter Polynomfolgen wird hier verzichtet, da eine rechnerische Beweisführung in MC14 in Anlehnung an den Originalbeweis im Vordergrund steht.