Accepted Solutions
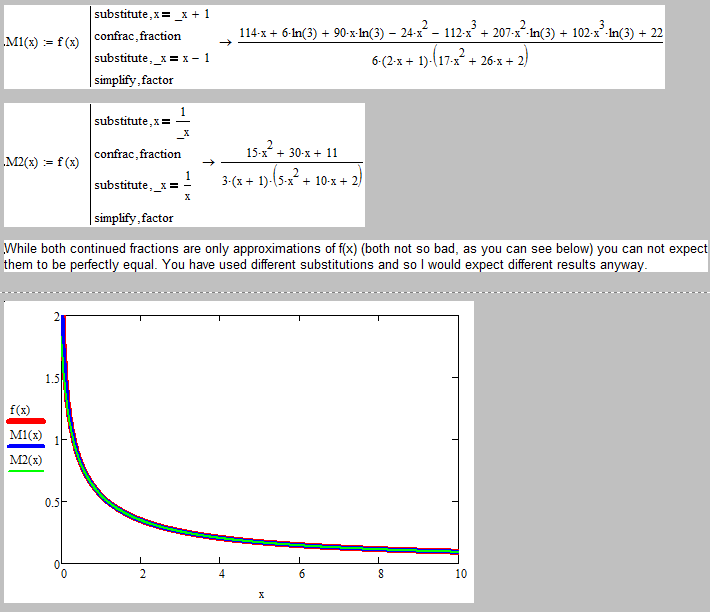
I don't know why you are confused. What you are doing the whole time is calculating approximations. With different substitutions you get different aproximation-functions. This is especially true as you compare a linear substitution to a nonlinear one. Your 1/x substitution has quite some advantages, though.
While I can see no reason why someone would do that I find it strange and have no suitable explanation for tha fact that applying confrac a second time makes the approximation that bad.
As with a Taylor series you can have a continued fraction, but not a standard one with respect to x but e.g. with respect to x-1
I don't know why you are confused. What you are doing the whole time is calculating approximations. With different substitutions you get different aproximation-functions. This is especially true as you compare a linear substitution to a nonlinear one. Your 1/x substitution has quite some advantages, though.
While I can see no reason why someone would do that I find it strange and have no suitable explanation for tha fact that applying confrac a second time makes the approximation that bad.
