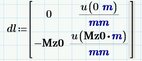Calculate the maximum deflection of a structure subjected to bending
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Calculate the maximum deflection of a structure subjected to bending
Hi
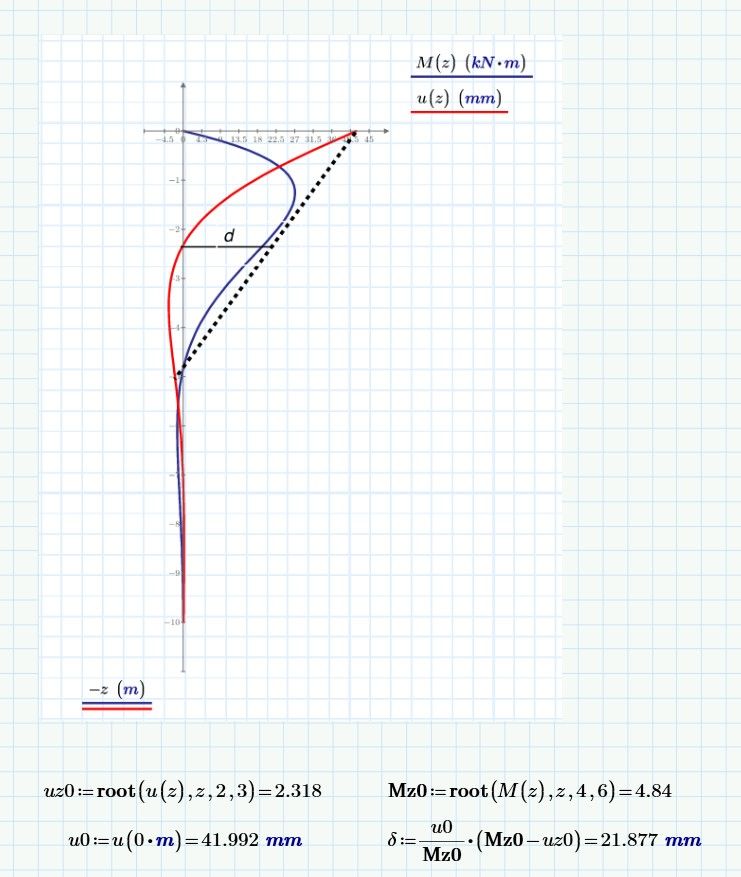
I want to calculate the maximum deflection of a structure subjected to bending. The deflection occurs within the buckling length, defined as the deflection within the points of inflection i.e. where M(z)=0.
I have plotted the moment and the deflection in the attached file, but need further assistance finding the maximum deflection within the buckling length (see page 2 in the attached file).
Any help appreciated!
Solved! Go to Solution.
- Labels:
-
Civil_Engineering
-
Mathcad Usage
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi,
Like this?
And if you include the small negative deflection at the lower point you get.
Cheers
Terry
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you. What I'm really looking for is a function that automatically plots the dashed line and the "d-line" for any generic shape of the deflection curve.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
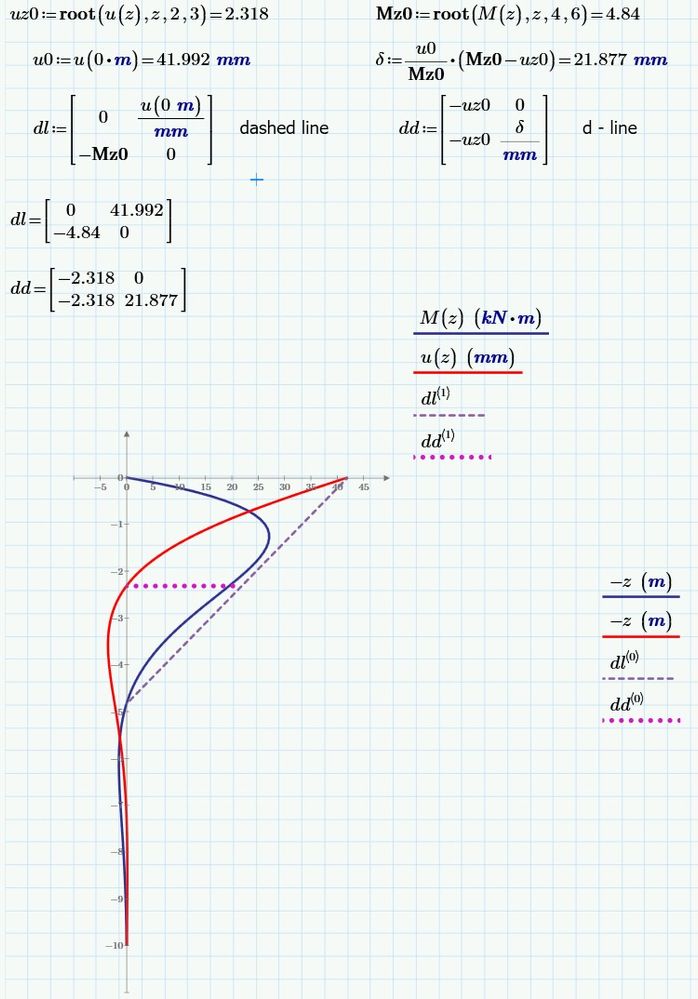
Something like the attached?
Its based on Terrys solution.
You have a confusing mix at the abscissa - two quantities of completely different dimensions (units mm and kN*m).
So I decided for a dimensionless approach - after all Terry already made Mz0 and uz0 dimensionless instead of the appropriate units meter because he used root without units. So all I had to do was to get rid of the remaining units.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Would you mind posting a print screen? I assume you have a more recent version of Prime.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
The lines will update automatically with changes in data
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Here's Werner's file for your version of Prime.
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks to both of you! Very appreciated!
I think the dl-matrix should look like this so the dashed line connects to the red line.
However, I am not sure if the "dd-line" is correct. I want the "dd-line" to automatically plot the largest gap between the dashed line and the red line. As it's plotted right now, It might be correct for this case, but I doubt it's correct for the general case.
Thanks for your support!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
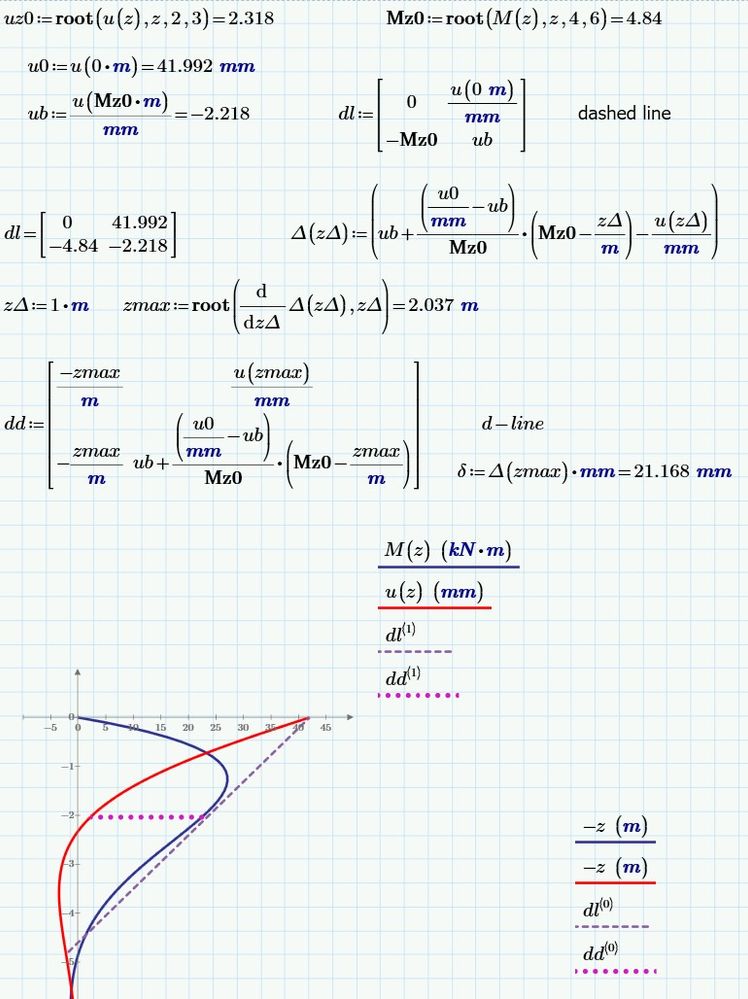
Hi
Here is an adjustment to Werner's file Prime 9 that does the trick.
Cheers
Terry
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Terry's file translated to Prime 7.
Success!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Excellent! I'm grateful for your help