Community Tip - Need to share some code when posting a question or reply? Make sure to use the "Insert code sample" menu option. Learn more! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Mathcad Community Challenge November 2022 - American Football Field Goal Geometry
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Mathcad Community Challenge November 2022 - American Football Field Goal Geometry
This month’s challenge is around field goals in American football.
Create a Mathcad worksheet where you calculate the following angles from a given line of scrimmage for making a field goal:
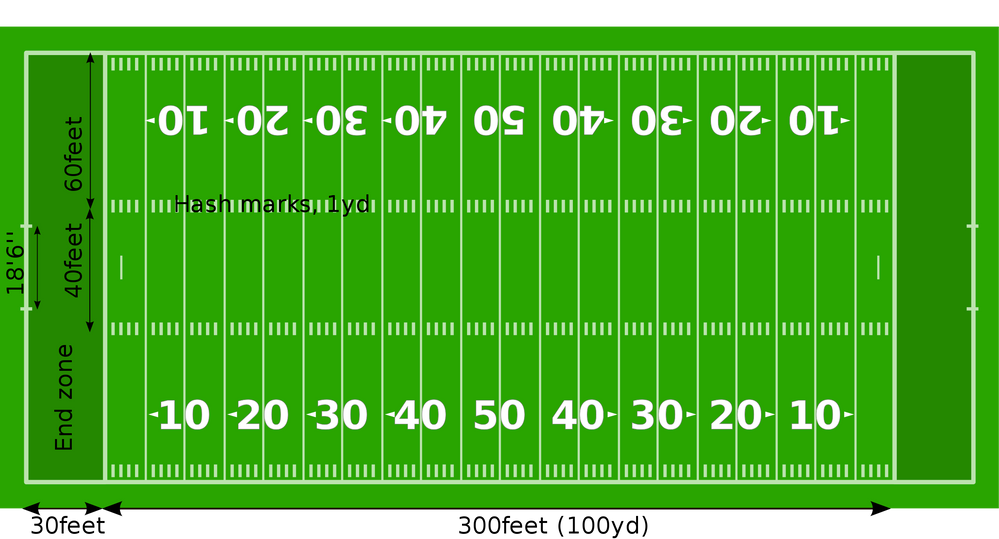
- The horizontal angle between the left and right field goal posts (18.5 feet apart).
- The vertical angle between the horizontal bar of the goal post and the upper height of the goal post arms.
How can you show how those angles change as a function of the line of scrimmage?
Factors to take into consideration:
- Assume the football is kicked from one of the hash marks (offset from the center of the field) and 7 yards behind the line of scrimmage.
- The goal post is located at the back of the end zone. The horizontal bar is 10 feet above the ground and extend another 35 feet. For this exercise, the football must pass through the box defined by the goal post.
- American football uses yards, feet, and even inches, so you might want to take advantage of units in Mathcad.
- Bonus: Can your worksheet handle entering yards from “your” side of the field (beyond the 50 yard line)? If not, feel free to use lines of scrimmage in excess of 50 yards.
- You can ignore air resistance for trajectory calculations. You can also ignore anything pertaining to the shape of the football or its motion through the air (treat it like a point; no need to turn this into a doctoral thesis).
We’re dealing with Euclidean geometry, so the math shouldn’t be that complicated. What matters is your execution. What tools (like plots and charts) can help you communicate the results?
Find the Mathcad Community Challenge Guidelines here!
By the way, the longest field goal in NFL history was kicked by Justin Tucker of the Baltimore Ravens in September 2021. It was 66 yards, meaning the line of scrimmage was the Detroit 49 yard line, and it was kicked from the Baltimore 44 yard line. Next time you’re on a football field, check out how far that is and how narrow the window looks. Field goal range is considered to be within the opposing team’s 35 yard line, which means the kick is 52 yards.
Solved! Go to Solution.
- Labels:
-
Puzzles Games
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I didn't do the best job of documenting my trigonometry and what my variable names refer to, but I think I hit the two objectives!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So that I dont have to talk to Bill Belichick can the kicks be made from the extreme left and right offset or just the middle offsets?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
For this challenge, the kick will be made from either the left or right hash mark in the middle of the field (the ones located 40 feet apart from each other). Not the ones along the sideline that help players judge whether they are in the field of play.
And you spelled Belicheat wrong.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hmmm! Did I miss it? Or have you not specified the initial velocity or the impulse of the kick? If it's me kicking, the ball won't ever get to the goalposts! 😉
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Maybe we have to assume that when the ball goes over the goal it’s vertical velocity is zero.
Then we can work out the time and then the required horizontal velocity.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
So the ball stops falling as it gets to the goal? Does not continue to the ground? Does not bounce?
Hmmm!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ignore the third bullet for now. I thought I had a solution that didn't involve initial velocity. Unfortunately I'm too booked up today to go back to research it. (I came up with this problem weeks ago.) Due to expediency, I will probably just take the third part out.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
With my newly granted editing powers, the third bullet has been... removed!
Hopefully this should get the first brave soul to submit something?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Okay! Challenge to find required velocity? You're on!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
But if I want to make it into a dissertation....
Does the field run the typical North-South direction? And are we kicking north or south?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Okay!
Express can't solve this for a general case. But a solve block can.
Prime 4 Express file attached.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Here is my go at it.
May have done better with soccer!
Prime 8 file attached.
PDF attached
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I didn't do the best job of documenting my trigonometry and what my variable names refer to, but I think I hit the two objectives!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Quick reminder to everyone that November only has 30 days, so this is the last day the challenge will be open for canon submissions!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks to those that participated (and PTC Community badges are given out). Here's Dave's write-up blog: https://www.mathcad.com/en/blogs/community-challenge-football-field-goal-geometry