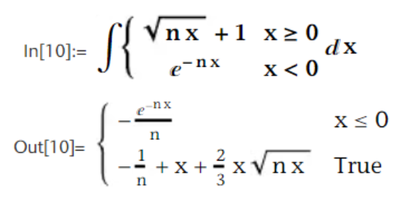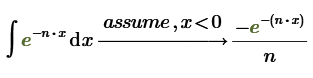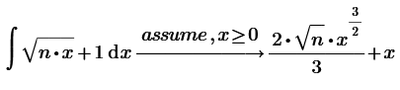1 ACCEPTED SOLUTION
Accepted Solutions
@CornelBejan wrote:
I think this happens because of that constant which normally is added in the indefinite integral calculation:
Correct. And if a software by default ignores/omits that constant of integration in indefinite integrals, it also should omit that summand 1/n. So Prime is also a bit more "correct" here because its result for this integral also covers correctly the case of n=0 whereas the results of Mathematica is wrong and invalid for n=0.
As for the other integral I am disappointed that neither software considers the case n=0 and simply ignores it!
I think this happens because of that constant which normally is added in the indefinite integral calculation:
@CornelBejan wrote:
I think this happens because of that constant which normally is added in the indefinite integral calculation:
Correct. And if a software by default ignores/omits that constant of integration in indefinite integrals, it also should omit that summand 1/n. So Prime is also a bit more "correct" here because its result for this integral also covers correctly the case of n=0 whereas the results of Mathematica is wrong and invalid for n=0.
As for the other integral I am disappointed that neither software considers the case n=0 and simply ignores it!
