Community Tip - Visit the PTCooler (the community lounge) to get to know your fellow community members and check out some of Dale's Friday Humor posts! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Odesolve - in Mathcad 12 OK, in Mathcad 15 and Prime - not OK. Why?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Odesolve - in Mathcad 12 OK, in Mathcad 15 and Prime - not OK. Why?
Odesolve - in Mathcad 12 OK - see please the picture
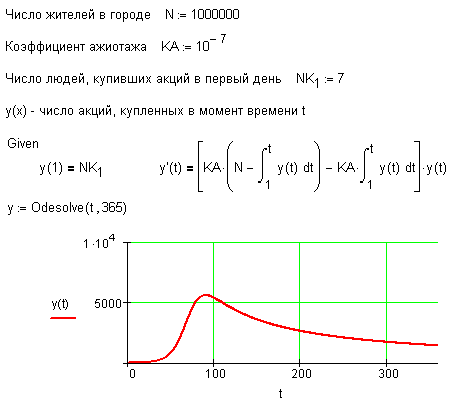
But in Mathcad 15 and Prime - not OK - see please attach.
Why?
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ok. Firstly, the value of KA in FinPyr2.mcd is different from the one in 15-19-Diff-Int-ODesolve.xmcd! However, this doesn't significantly affect the problem.
Interestingly, in Mathcad 11 it only works if, in the integral, you enter y(t)dt (i.e. you use the same symbol, 't', as for the upper limit). It doesn't work if you enter y(tau)dtau, say, where tau is a different symbol from the 't' used as the upper limit! It also doesn't work if you define the integral (using either y(t)dt or y(tau)dtau) as a function before the solve block (say f(y,t):= integral stuff) and replace the integral within the solve block by f(y(t),t).
It looks to me as though the MC11 "solution" is incorrect!
Alan
.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hmmm! Something odd here Valery. I've just tried this in my version of Mathcad12 and it doesn't work. I get the error message: "This definition forms part of a dependency cycle"
Also, the solution I get in M15 (doing it a different way) doesn't look like the solution you provided (see attached)!
Alan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Sorry, it was Mathcad 11
See please this task on Mathcad Application Server 11:
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ok. Firstly, the value of KA in FinPyr2.mcd is different from the one in 15-19-Diff-Int-ODesolve.xmcd! However, this doesn't significantly affect the problem.
Interestingly, in Mathcad 11 it only works if, in the integral, you enter y(t)dt (i.e. you use the same symbol, 't', as for the upper limit). It doesn't work if you enter y(tau)dtau, say, where tau is a different symbol from the 't' used as the upper limit! It also doesn't work if you define the integral (using either y(t)dt or y(tau)dtau) as a function before the solve block (say f(y,t):= integral stuff) and replace the integral within the solve block by f(y(t),t).
It looks to me as though the MC11 "solution" is incorrect!
Alan
.


