Community Tip - Learn all about PTC Community Badges. Engage with PTC and see how many you can earn! X
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
System of Differential Equations - How to solve? - 2nd Edition
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
System of Differential Equations - How to solve? - 2nd Edition
Hello,
I have the same problems to solve a system of differential-equations with the solve-block like in tried in my thread: System of Differential Equations - How to solve?
See attachement please, I'm working with Prime 3.1.
Thank you for your efforts.
best regards, Volker
Solved! Go to Solution.
- Labels:
-
Other
Accepted Solutions
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Setting the Air-resistance to a function below, allows a damped character of the curvation.
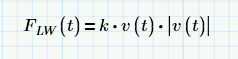
Now it's possible, that the force can take a correct effect into two directions.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Volker,
die erste Gleichung hat, laut .PDF, x(t)'' anstatt x''(t)...
Viel Erfolg!
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hallo Luc,
vielen Dank, hab's geändert.
Leider ergibt das auch noch keine Erfolg.
Gruß, Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Can't read P31 files but how about replacing every lambda by y'/(2xx') ?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
I changed lambda, but no sucess.
anyway thanks
Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Schade! Allerdings kann die numerische Lösung mit einem unbekannten lambda meines Erachtens nicht funktionieren. Denn da nicht nach lambda gesucht wird, wird es auch nie neu berechnet oder variiert und ist vermutlich leider nur einer der Gründe, warum der solver einen Fehler produziert. Ich denke also, dass lambda auf jeden Fall elimiert werden muss, oder aber durch eine Funktion lambda(abhängig von ...?) ersetzt gehört. Denn was soll ein numerisches Verfahren mit einer unbekannten Konstanten anfangen?
P.S.: Mir fällt gerade auf, dass du überhaupt keine der Konstanten (k, mk, mu) mit einem Wert belegt hast. Mathcad kann DGLen nicht symbolisch lösen und für eine numeriosche Lösung, benötigt es auch konkrete Werte!
P.P.S.: Auch die Definition von te ist nicht erkennbar, aber trotzdem produziert die Definition der Bereichsvariablen fürs Plotten keinen Fehler. Ich nehme daher an, dass du die Konstanten in einem Bereich rechts definierst, der in der PDF-Datei nicht sichtbar ist.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
Volker hat die Konstanten definiert, aber in einem zugeklappten Areal gesetzt.
Hier sind sie:
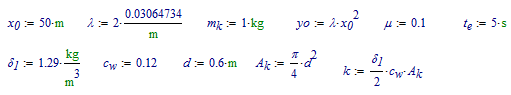
Wie du siehst hab ich versucht die sache in Mathcad (anstatt Prime) zu lösen, ohne Erfolg. Bekomme immer wieder das zu wenig Initialkonditionen gegeben sind.
MathCad hat die Beschränkung das die höchste Ableitung nur linear vorkommen darf. Das war bei das erste Problem sicher nicht so. Hier müsste es klappen, tut's aber nicht und ich weiss nicht warum.
Kannst selber mal probieren ob 's mit Mathcad 15 funktioniert.
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks for the information!
I guess FM (see his answer below) has already solved the problem by substituting for y'(t):
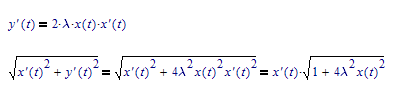
This also works in Mathcad 15 (you additionally had a typo in y.0 and used the Prime syntax for odesolve)
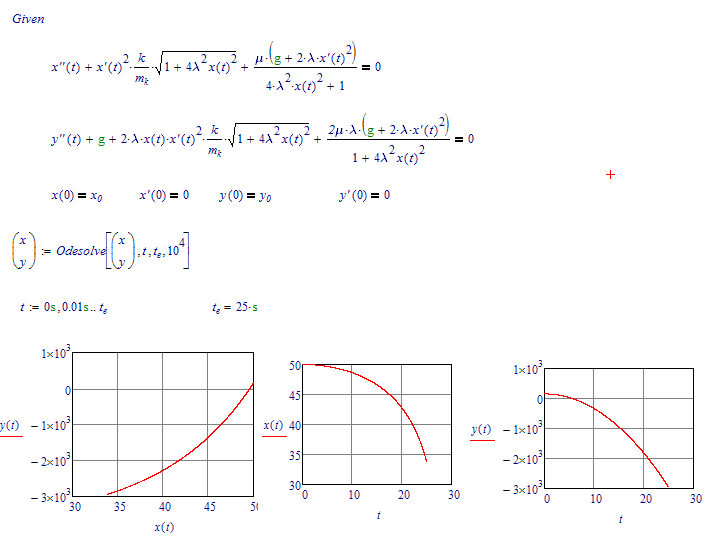
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hmmmm !?
It looks like its not that easy!
Your (Luc) sheet also solves if we omit that extra condition, but that condition is not fulfilled with the given constant lambda then:
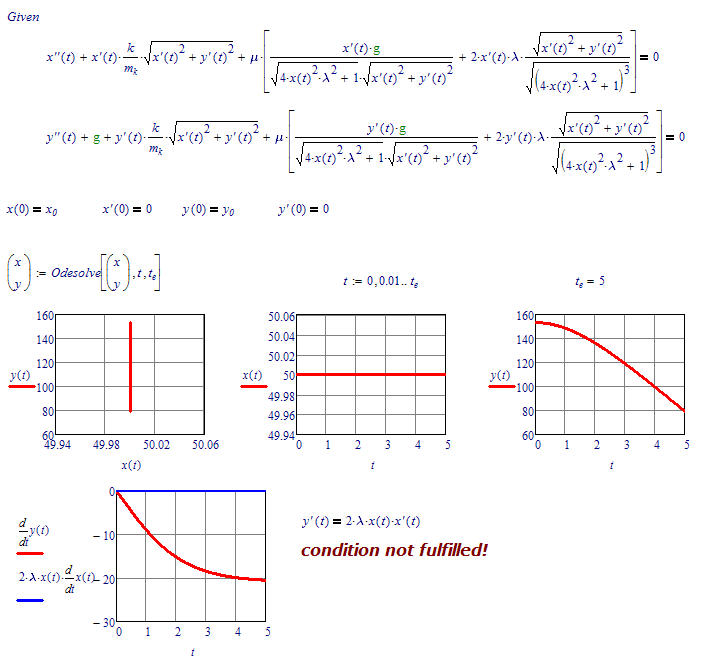
But even if we use LM's substitution as in my post above, the condition still is not fulfilled!!

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You lost an x(t) in the second equation:
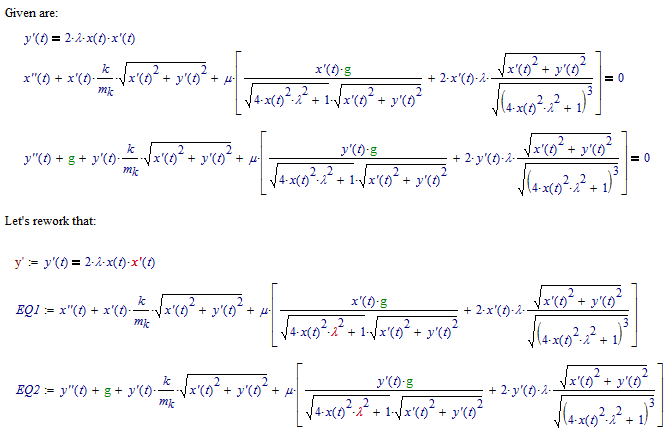
Now:
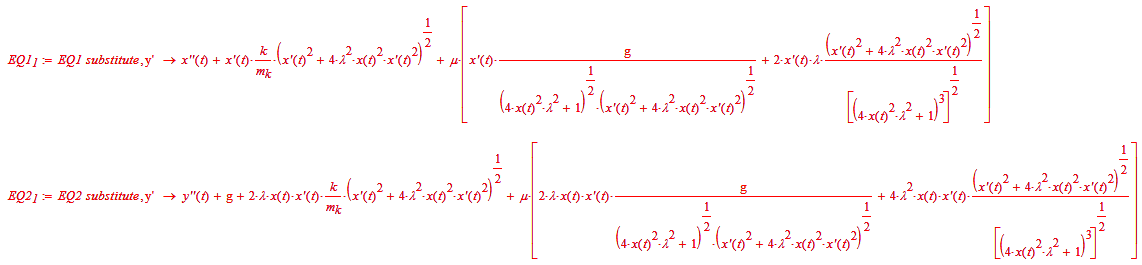
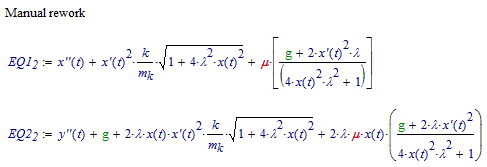
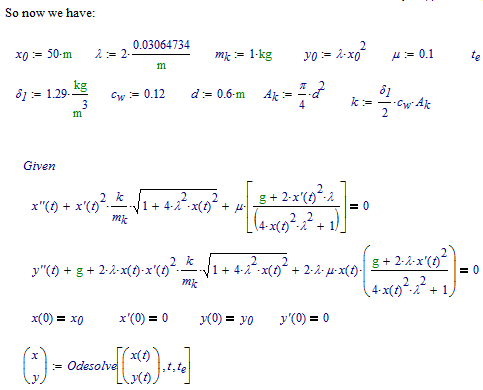
Does not mean that Mathcad 11 is happy to solve it, still too few initial conditions. ![]()
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
LucMeekes wrote:
You lost an x(t) in the second equation:
Oh, I see. Thanks! Nevertheless the condition still is not fulfilled. Three equations for just two functions does not work.
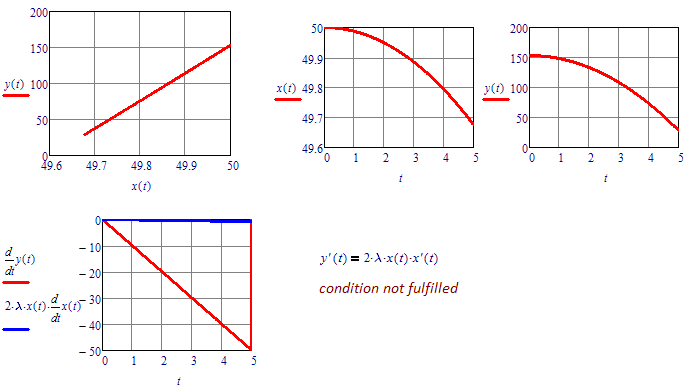
Does not mean that Mathcad 11 is happy to solve it, still too few initial conditions.
Luc
I think you still have the wrong syntax for odesolve (or was it different in MC11 and more similar to the Prime syntax? Can't remember.). In MC15 you simply write x and y, not x(t) and y(t).
I attach the corrected sheet and a pdf-printout.
EDIT: Replaced the MC file for a MC11 version.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Werner,
Thanks for the correction. I don't use odesolve much (not enough?). Now it works, in the sense that I get a result.

Whether it is the solution is up to debate, because the one equation I substituted in is obeyed except for a constant factor.
(I'd expect dy/dt versus 2 lambda blabla to be the identity line, a straight line through (0,0) and (1,1). It is a straight line, goes through (0,0), but not through (1,1).
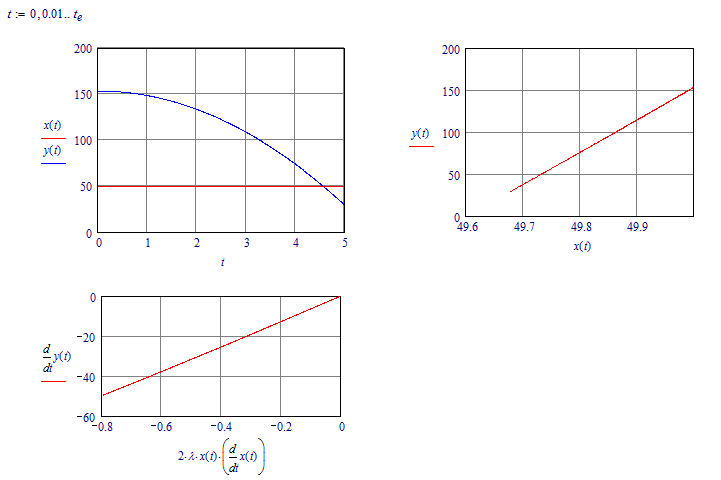
I can stretch t.e all the way to yust before 30, then y(t) versus x(t) deviates from a straight line

Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Someone nicht mit die ordnung with units?

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Units look OK for me in this case:
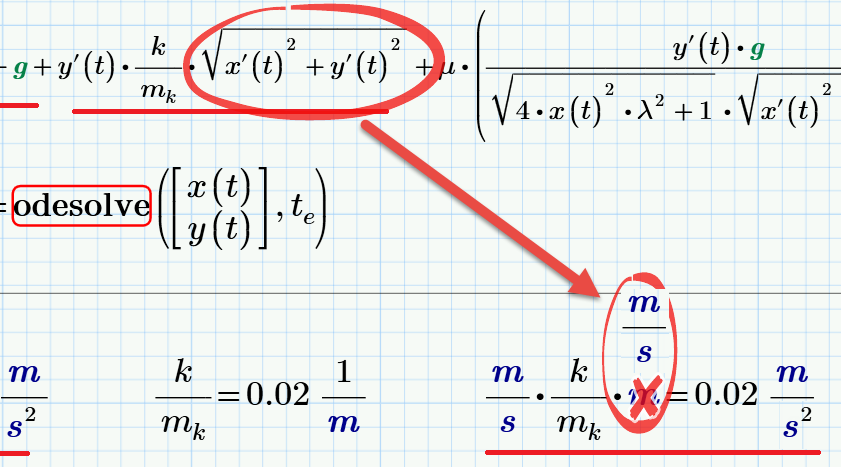
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Werner,
Please find this worksheet for a Prime 3.0 version in attachment.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Volker,
my solution:
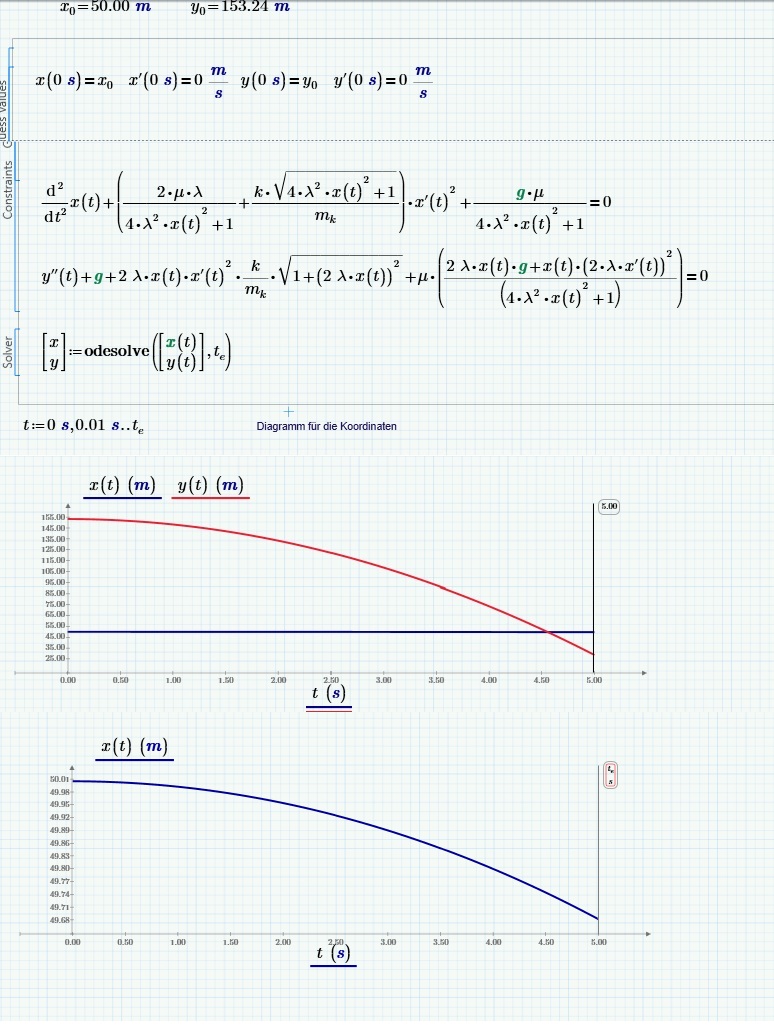
Ich denke eigentlich, dass die Lösung nicht so gut geht .... Ich denke, dass nicht die Lösung der Bewegung einer Parabelbahn ist ....
vielleicht die Unsicherheit ist abhängig von der Tatsache, dass die Berechnung auf den Bereich beschränkt ist 0s <t <= te
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi FM,
your equations looks in comparison to mine very strange. How did you got it?
Lambda is a constant in this system.
To all: Thanks for your efforts again! Good hints- I have to check many things...
regards, Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Volker,
for the constants it seems that it is better to choose the automatic label.
Greetings
FM
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
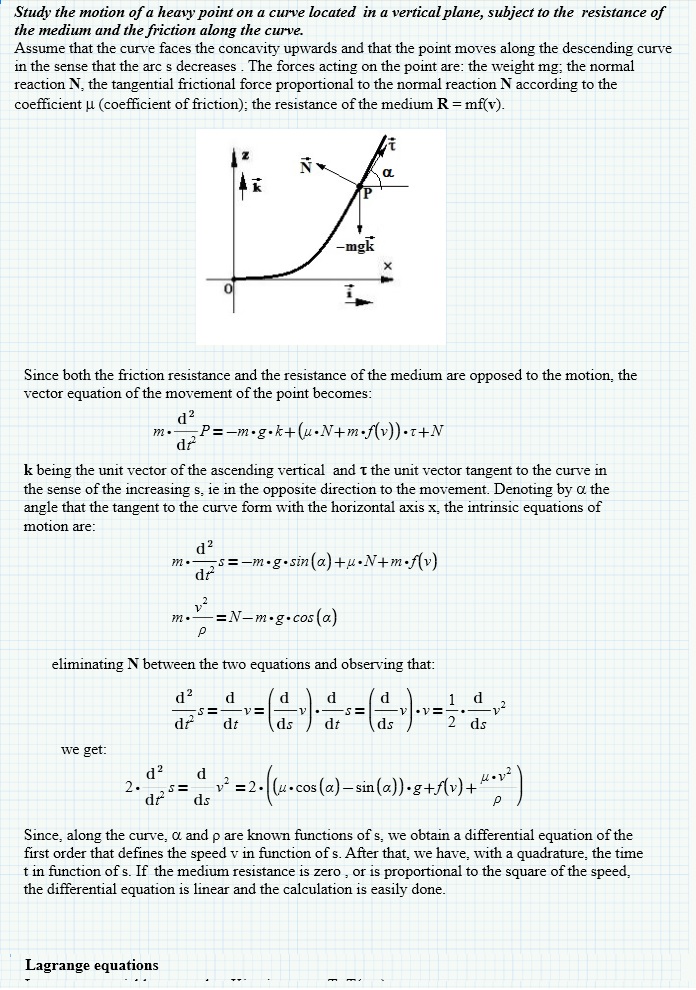
- I made a classical Analysis of all Forces and generated a system of differential equations for the x and y direction. It works.
- I revised the sheet of the Lagrangesche Method according to your tips and it works too.
- The file "Massepunkt Parabelbahn" shows the kinematics without friction and air resistance- a harmonic periodical system.
The curves in the first two cases are looking strange- I expect a periodic-damped curvature but it didn't do so.
What's wrong?
regards, Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Volker,
There's one reason why I can imagine your results don't show the effects of friction and air-resistance, but I realise I might be completely beside the point.
Anyway: You model your system with a point-mass. A point-mass has no surface and no perimeter which means a total lack of parameters where friction or air-resistance can act upon.
Luc
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Luc,
You are right on one side - a mass point does not really occur in nature - but it's applied in many physical problems.
I'll try to take into account the mass of inertia- but the equations will be very large and therefore confusing...don't know if sucess...
Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi F.M.
In this illustration I miss the influence of centrifugal force as an additional influencing variable on the path friction.
Normally we have a radius of curvature which is given by:

Can you send me the complete File please?
Thank you.
regards Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Volker,
I'm sorry, the file is being processed so I can not send it.
In the foregoing description the radius of curvature of the osculating circle to the generic curve, is given by:
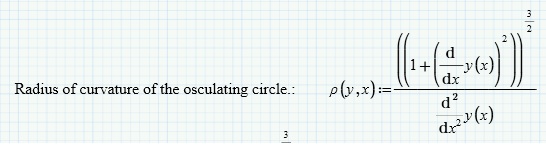
as you have pointed out.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi F.M.
do you have meanwhile a solution?
I checked all again but there's nothing news.
Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello Volker,
although the problem interests me a lot, actually, I have dedicated myself to something else, thinking that you had solved the problem.
When I run into endless calculations or impossible solutions, it is my habit to start all over again.
I would advise you to do the same. Or wait other solutions from the community.
Greetings
F. M.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi,
I only saw the pdf-file, perhaps I miss something, but I see 2 ode`s second order. You need 4 boundary conditions (bc`s) to solve them. But I see 5 bc`s.
Best wishes
Stephan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Stephan,
I have two differential Equations second order with four BC's.
regards, Volker
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Ok, I put some time in the worksheet.
I changed some points and got a solution:
First: I delete every units. (normally it should work with units, it seems there is a mistake in the formulas)
Second: I changed the term x(t)'' in x''(t), see picture below (first arrow).
Third: I changed ("*") into ("+") (second arrow in both ODEs). This is perhaps physically wrong, but there seems to be something wrong in your formula as well, otherwise it should work.

You can see my full changes below. Especially Point 1 und Point 3 are signs for a mistake in the formula, but i am too far away from that topic to see what's wrong.
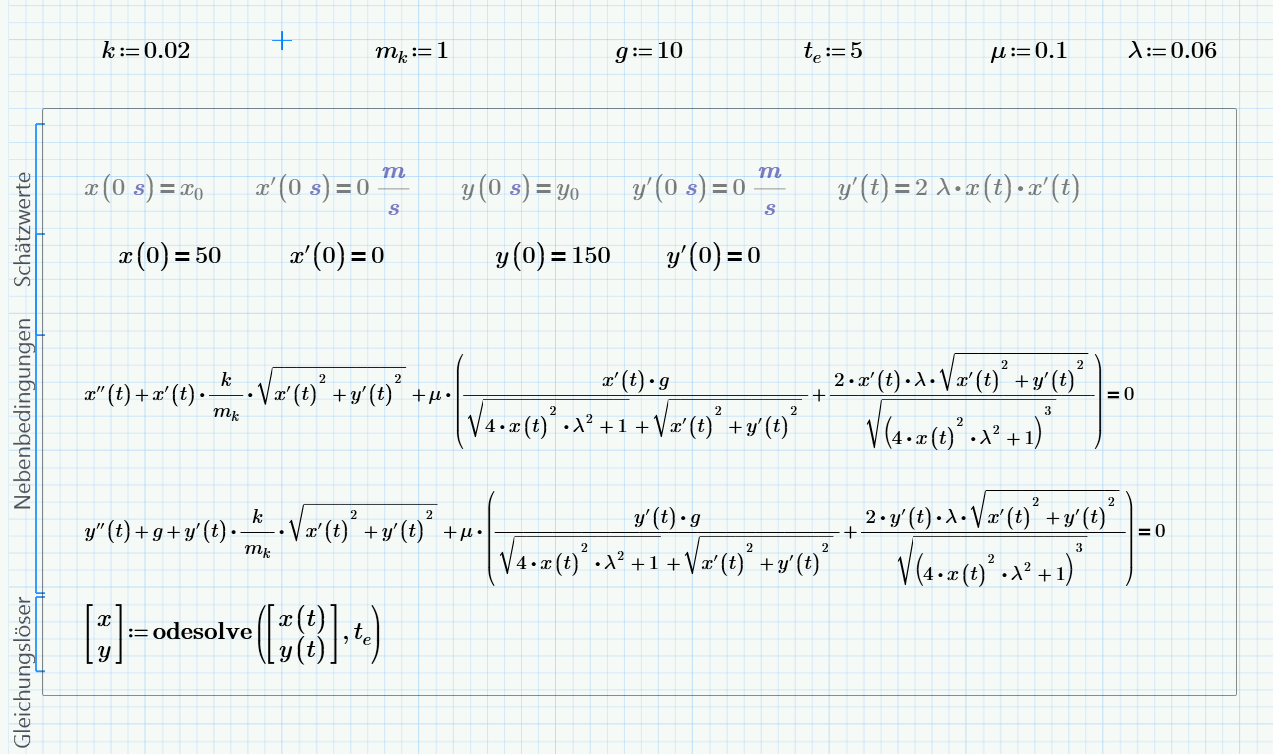
I hope that will help.





