Community Tip - Learn all about PTC Community Badges. Engage with PTC and see how many you can earn! X
- Community
- PTC Education
- PTC Education Forum
- Re: Conch shell in Creo Parametric
- Subscribe to RSS Feed
- Mark Topic as New
- Mark Topic as Read
- Float this Topic for Current User
- Bookmark
- Subscribe
- Mute
- Printer Friendly Page
Conch shell in Creo Parametric
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Conch shell in Creo Parametric
Hi all
how about this one for a problem to solve. I am trying to create a conch shell using the helical sweep tool, I can do it so far and then I hit a brick wall namely my mathematical ability, or should I say lack of mathematical ability. The conch shell is creted in nature obeying rules that can best be described by the using Fibonacci principles. That is the bit that beats me. Using the Vary Section option I can create a pseudo conch shell. See below
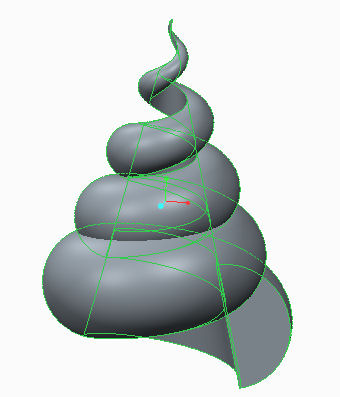
What I would like to do is now vary the pitch so that it changes related to the fibonacci series. Has anyone any clues to how I might do this. The Hel;ical sweep has a variable pitch function in Creo Parametric by setting a series of points along the profile curve axis. If I can set the points in the ratio of 1.618 , the golden section I think I can do it. Am I on the right track? I attach my Schools Edition file just in case anyone else would like to play.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
JohnF,
I think we have some helical sweep experts (@VladimirPalffy, @GregFrankland, @ChristianSmith, @DavidButz) that may have an answer.
-Dan
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Dan
Can I contact these gods of trajpar direct?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You can most certainly network with them by sending a PM (Private Message) or Adding them as a friend. I sent them all a personal note asking if they could help you out when they have a free moment, also by referencing their names within a post, it sends a "Mention" notification alerting them that they may want to be part of the conversation. It's kind of like the Bat Signal
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
How do get my Bat signal to work?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Interesting situation! Unfortunately I cannot read the student version to see what you have tried thus far. Can you explain a little more what you have done in the file attached? Are both the pitch and the section diameter affected by the same 1.618 ratio?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Gregory
The spiral was created using the Helical Sweep tool in Creo Parameteric with a constant pitch but using a surface cone to which the section was locked by a tangent using tehVary Section option in the Helcal Sweep tool. As you can see from the image this creates what looks like a conch but because I cannot vary the pitch geometrically/parametrically it does not create a proper conch spiral or equiangle spiral. The pitch needs to change by 20% per revolution roughly however the math is somewhat complex. I think I willhave to use a Sweep and create the path via trajpar function and vary the section somehow using a relation. So far all I am managing to do is confuse myself!! Hope you can help.
Regards John E Forth
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi all,
I have converted the data from educational to commercial.
http://apps.ptc.com/schools/conche.prt.1
The file is Creo 1.0 Parametric.
Thanks,
Mark
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
We (Roger, John, Lino, and I) played around with the idea using Mathcad Prime and Creo. A Mathcad function is used to generate the Fibonacci Sequence and the (x,y,z) coordinates for a spline curve based on Fibonacci. These coordinates can then be used to generate conche geometry in Creo Parametric.

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
This is interesting.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You can use Creo View Express to view this file (conche.prt.2😞 http://www.ptc.com/products/creo/view/express/
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thank you all for you interest and pointers. I now need to work out how to create the curve for the sweep path using a trajpar function. If someone with more mathematical acumen than I can lay out the formula for me I would be most grateful. I think I can work out the relations to set the section to vary.
Regards John E Forth
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
John,
You started quite a buzz internally here at PTC. Check out this blog, http://blogs.ptc.com/2011/12/01/the-fibonacci-conch/
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Those Mathcad guys are always showing off. I think we can do this in basic Creo... maybe with a datum graph. Trajpar is a wonderful thing but it's also not necessary all the time... there are other ways to create interesting helicals and strange sweeps without it.
Hmm... finally something fun to play with!
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hi Mark...
Can you upload this as a zip. Clicking the link opens the file itself rather than allowing a download. Maybe I should try IE instead of Mozilla.
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Brian,
You should be able to right-click on the link and select Save Target As from the pop-up menu to save the part (rather than open it). If not, let me know and I'll email it to you.
Looking forward to seeing what you come up with!
Cheers,
Adam
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello John
I try to create Fibonacci Spiral through Golden Rectangle with creo Parametric.
Here is some examples of my 3D model "Fibonacci Spiral" (I use some sketch and variable section sweep with Trajpar functions)
If that's what you need, I can make a short video demonstration. Let me know ![]()
Golden Rectangle and Fibonacci Spiral

3D Fibonacci Spiral

3D model of Fibonacci Spiral

Best Regards
Vladimir
Vladimir Palffy
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Thanks Adam.. got it.
While I think Vladimir is on the right track, if you go back to John's original post, he's trying to use the Variable Pitch feature of a Helical Sweep to accomplish the shape. This makes use of an internal datum graph. The problem here is that you don't really have much control over the graph.
If one were to create a Datum Graph as a standalone feature, there are more options available (such as relations) which could help define the shape of the graph. With the pitch graph inside the Helical Sweep feature, you're limited to simply plotting points along your profile sketch. This is where John is getting stuck.
I can make a standalone datum graph... and I think I can use equations to develop a fibonacci curve for the pitch. This doesn't need to be a 3D spiral curve... it just needs to represent the ever-increasing rate of pitch... sounds like a parabolic curve to me (like the shape of the graph of y = x^2 only with fibonacci values). The problem comes in that I cannot use my standlone graph in place of the pitch graph Creo wants me to use. I think this is exactly what John is saying.
The obvious answer is what Vladimir did... he skipped the Helical Sweep feature altogether and went directly for a Variable Section Sweep. This was a logical approach... but I'm still not convinced it's the only way.
I'll keep tinkering... anyone else have any ideas?
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
My life what have I started! I just hope I can do your efforts justice. However a word of caution here. This may well be an interesting mathematical exercise but the reason for it is to produce a shape that can be used as an electric kettle. Therefore it has to be made to, literally, hold water. So far we have been able to create a solid and a surface model of a spiral but neither can be, respectively shelled or thickened. The reason is that the spiral is by nature a self-intersecting solid. The real 'engineering' trick is to create the conch and then turn it into an injection moulded reality. Now that really is the challenge.
Regards
John E Forth
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello John,
you are right - it is interesting mathematical exercise but it has the geometrical solution.
You can combine geometric and mathematical model and create some conch like I tried.
For example: If you need create thin conch - You can create surface model and then create thin feature.
Remember >> it is not possible create self intersection feature.
Good luck,
Vladimir
Vladimir Palffy
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
With two features I was able to create the geometry below. The first feature is a curve driven by equation (cartesian coordinate system). For those of you familiar with equation-driven curves, you know that there's a variable "t" in the equations which does the same thing as trajpar.
A fibonacci spiral, as we've seen, is similar to what Vladimir used in his models. The spiral you get using the golden rectangles as Vladimir used is a very close approximation. Mathcad is giving us better results but Creo can come close. For true a true fibonacci spiral, the spiral needs to increase by a factor of phi (approx. 1.6180339887) every quarter turn. With a bit of playing around, you can get a curve driven by equation to approximate this behavior.
Once you have a suitable curve, this can be used as the basis for a Thin Swept Blend. This absolutely creates self-intersecting geometry. I humbly differ with Vladimir on this point. In my experience, Creo can create certain types of self-intersecting geometry. In fact, the geometry below self intersects multiple times.
While the outside of this conch looks decent, the 'guts' are a mess of mangled goo. With a better sketch for the swept blend, I think I can overcome that, too. This is preliminary geometry. The equation needs to be tweaked until it's matching the curves created by Mathcad. The swept blend needs to be tweaked to be less mangled inside.But I believe this sets us on a good course for an alternate modeling concept for the conch.
Thanks!
-Brian
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
Hello Brian
Your model look nice ![]()
Note: many times, when I try create a Sweep features with intersection geometry, I had failure geometry - GeomCheck - the feature was created but with errors.
For example:

Vladimir
Vladimir Palffy
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
You are all absolutely amazing, I will try to emulate your efforts.
Regars John E Forth
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
^^)

MAKE CURVE (BY HELICAL SWEEP USING VARIABLE PICTH) AND THEN USE SWEPT BLEND
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator

- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
- Mark as New
- Bookmark
- Subscribe
- Mute
- Subscribe to RSS Feed
- Permalink
- Notify Moderator
I've been looking extensively into the use of "curves by equations" in Creo. I would be interested in looking at the model mentioned. It appears the link is dead & the educational version of the file is not compatible. Would someone be nice enough to share again?





